The 50kg homogenous smooth sphere rests on the 30∘ incline A and bears against the smooth vertical wall B.Calculate the contact forces at A and B.
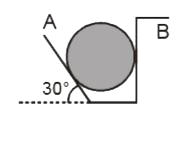
NA=1000√3N,NB=500√3 N
NA=1000√3N,NB=1000√3N
NA=500√3N,NB=500√3N
NA=500√3N,NB=1000√3N
The correct Answer is:A
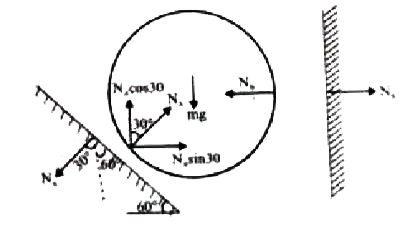
mg−NAcos30=0 [Eqluilibrium in vertical direction]
⇒NA=mgcos30∘
⇒NA=1000√3N
NB−NAsin30=0 [Equilibrium in horizontal]
⇒NB=NAsin30
⇒NB=1000√3×12
⇒NB=500√3N
Topper's Solved these Questions
- LAWS OF MOTION BOOK - PHYSICS WALLAHCHAPTER - LAWS OF MOTION EXERCISE - NEET PAST 5 YEARS QUESTIONS
12 Videos
- LAWS OF MOTION BOOK - PHYSICS WALLAHCHAPTER - LAWS OF MOTION EXERCISE - NEET PAST 5 YEARS QUESTIONS
12 Videos
- KINETIC THEORY BOOK - PHYSICS WALLAHCHAPTER - KINETIC THEORY EXERCISE - NEET PAST 5 YEARS QUESTIONS
11 Videos
- MAGNETISM AND MATTER BOOK - PHYSICS WALLAHCHAPTER - MAGNETISM AND MATTER EXERCISE - NEET PAST 5 YEARS QUESTIONS
10 Videos
Similar Questions
The 50kg homogeneous smooth sphere rests on the 30∘ incline A and against the smooth vertical wall B. Calculate the contact the forces at A and B

View SolutionA uniform beam of length 2 a rests in equilibrium with one end resting against a smooth vertical wall and with a point of its length resting upon a smooth horizontal rod which is parallel to the wall and at a distance b from it. The inclination of the beam to the vertical is sin−1(ba)13.
View Solution
Knowledge Check
The 50kg homogenous smooth sphere rests on the 30∘ incline A and bears against the smooth vertical wall B.Calculate the contact forces at A and B.
 ANA=1000√3N,NB=500√3 NBNA=1000√3N,NB=1000√3NCNA=500√3N,NB=500√3NDNA=500√3N,NB=1000√3N
ANA=1000√3N,NB=500√3 NBNA=1000√3N,NB=1000√3NCNA=500√3N,NB=500√3NDNA=500√3N,NB=1000√3NIf a ladder is not in balance against a smooth vertical wall, then it can be made in balance by :-
ADecreasing the length of ladderBIncreasing the length of ladderCIncreasing the angle of inclinationDDecreasing the angle of inclinationA sphere of radius 0.10m and mass 10kg rests in the corner formed by a 30∘ inclined plane and a smooth vertical wall. Choose the correct option
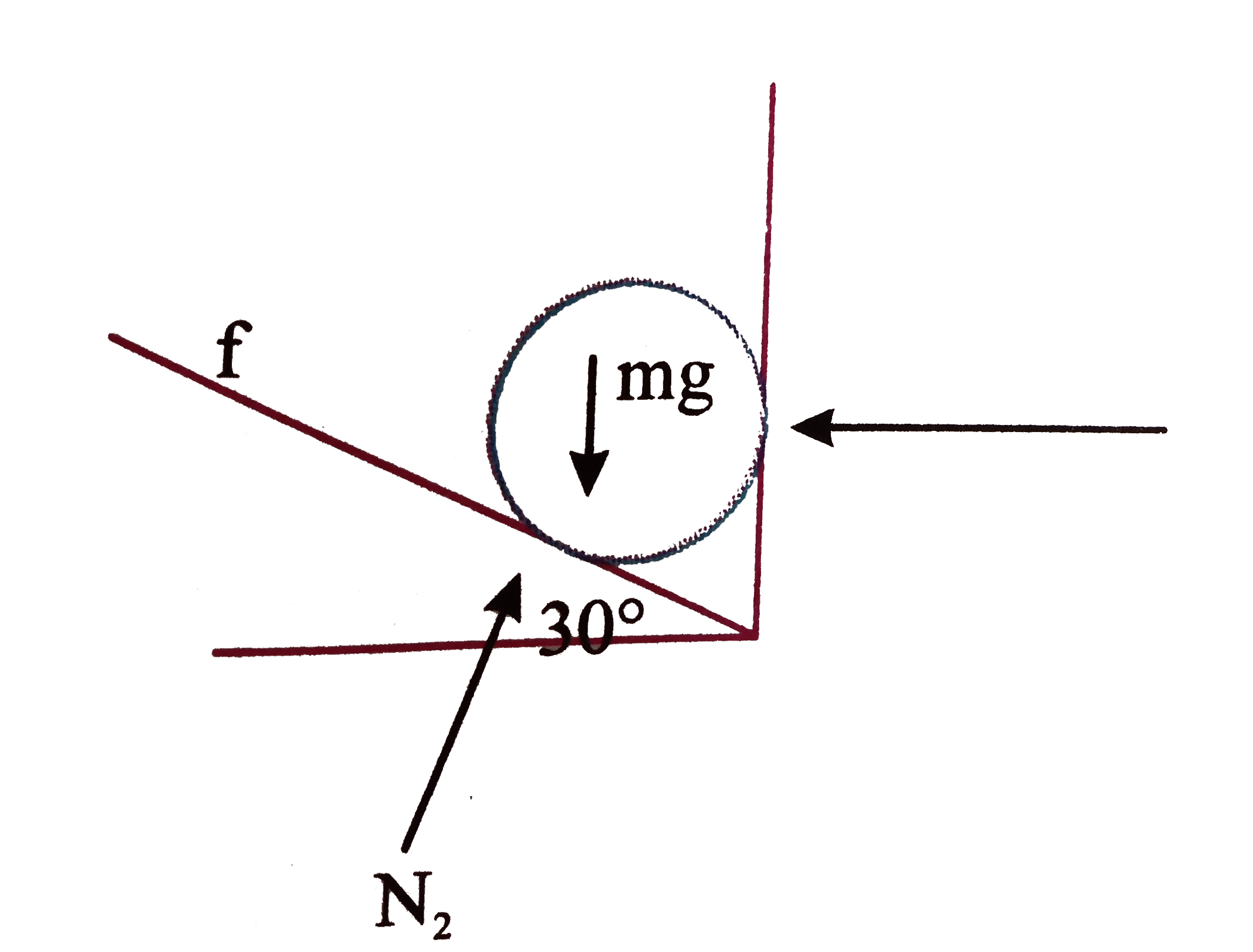 AN1=56.5NBN2=113NCf=0Df≠0
AN1=56.5NBN2=113NCf=0Df≠0
At the bottom edge of a smooth wall, an inclined plane is kept at an angle of 45∘. A uniform ladder of length l and mass M rests on the inclined plane against the wall such that it is perpendicular to the incline.
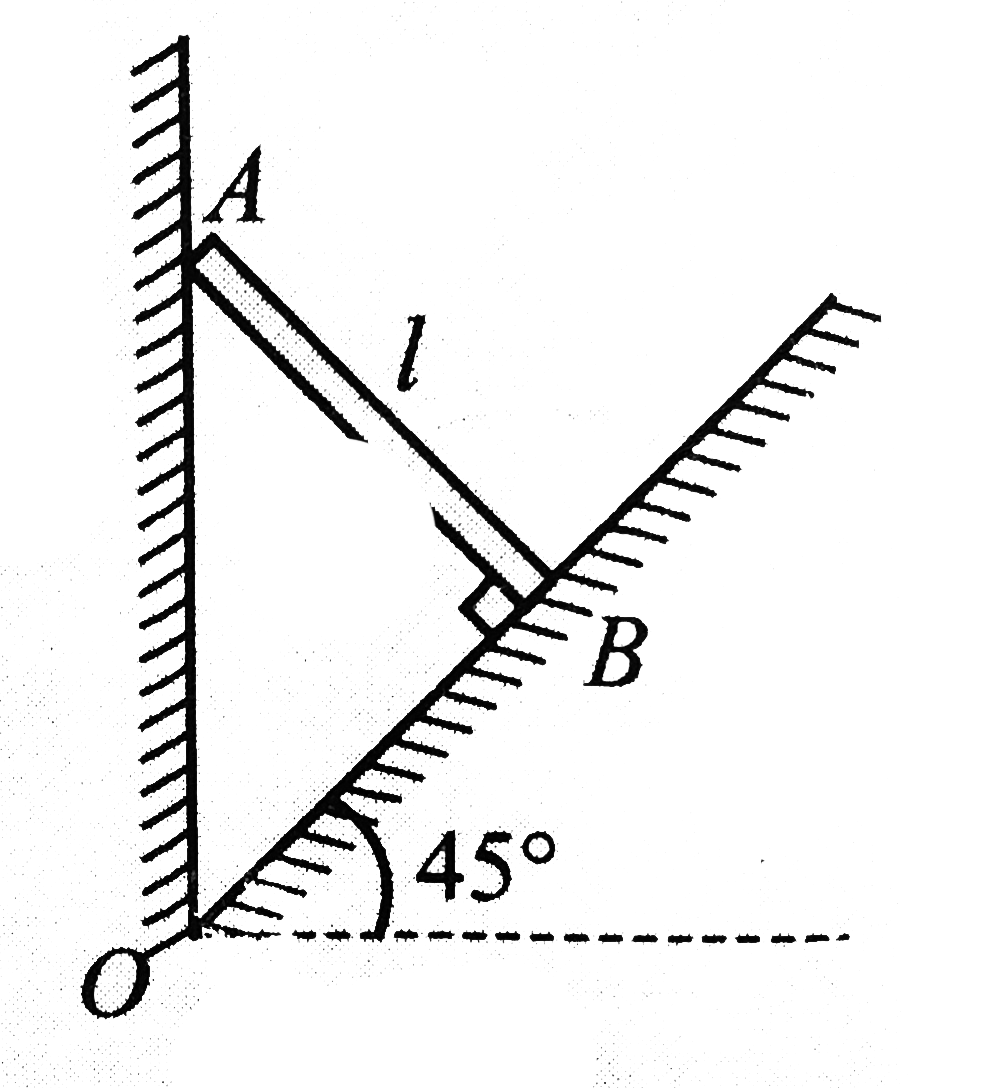
a. If the plane is also smooth, which way will the ladder slide?
b. What is the minimum coefficient of friction necessary so that the ladder does not slip on the incline.
View SolutionA uniform ladder rests in limiting equilibrium with its lower end on a rough horizontal plane with coefficient of friction μ and its upper end against a smooth vertical wall. If θ is the inclination of the ladder with the wall, then θ is equal to
View SolutionA uniform ladder rests in limiting equilibrium with its lower end on a rough horizontal plane with coefficient of friction μ and its upper end against a smooth vertical wall. If θ is the inclination of the ladder with the wall, then θ is equal to
View SolutionAn overweight acrobat, weighing in at 115 kg, wants to perform a single hand stand. He tries to cheat by resting one foot against a smooth frictionless vertical wall. The horizontal force there is 130 N. What is the magnitude of the force exerted by the floor on his hand? Answer in N.
View SolutionThe foot of a uniform ladder is on a rough horizontal ground and the top rests against a smooth vertical wall. The weight of the ladder is 400 units. A man weighing 800 units stands on the ladder one quarter of its length from the bottom. If the inclination of the ladder to the horizontal is 30∘, the reaction at the wall is
View Solution
