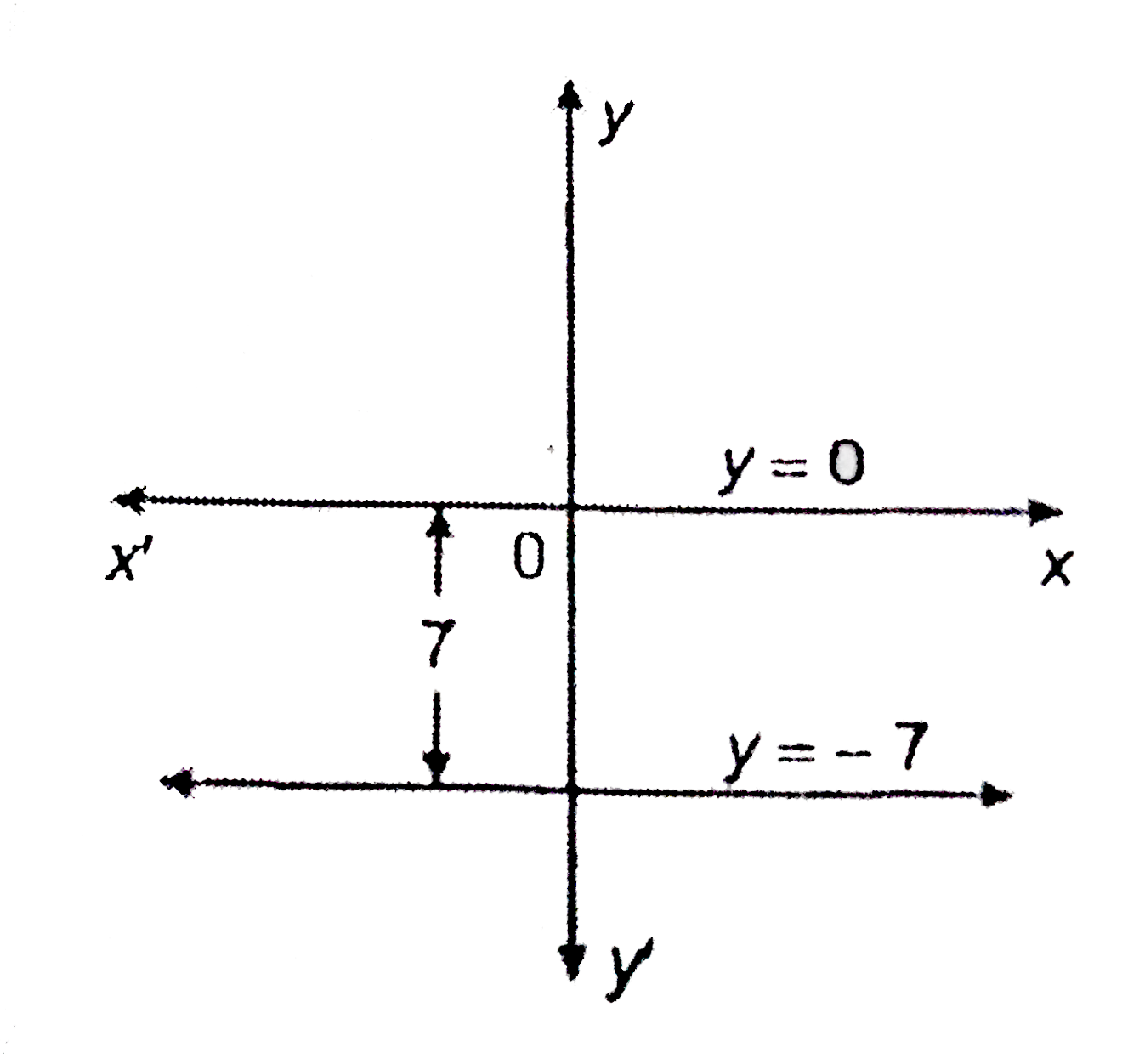
The pair of equations y=0 and y=−7 has
Graphically y = 0 is a straight line which is x - axis and y = - 7 is a straight line parallel to x - axis, 7 units below the origin therefore lines y = 0 and y = - 7 are parallel.
∴ They have no solution.
(because solution is the intersection point of both the lines).
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3a
18 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3A
8 Videos
- INTRODUCTION TO TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- POLYNOMIALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
Knowledge Check
Which of the following (x,y) pairs is the solution for the system of equations x+2y=4and−2x+y=7?
A(-2,3)B(-1,2,5)C(1,1,5)D(2,1)
Graphically, the pair of equations
6x−3y+10=0
2x−y+9=0
represents two lines which are
View SolutionThe linear equation 2x-5y=7 has
View SolutionFor the pair of equations λx+3y+7=0 and 2x+6y−14=0. To have infinitely many solutions, the value of λ should be 1 . Is the statement true ? Give reasons.
View SolutionFor the pair of equations λx+3y+7=0 and 2x+6y−14=0. To have infinitely many solutions, the value of λ should be 1 . Is the statement true ? Give reasons.
View SolutionFor which values of p does the pair of equations given below has unique solution?4x+py+8=02x+2y+2=0
View SolutionIf the system of equations x+ay=0,az+y=0,andax+z=0 has infinite solutions, then the value of equation has no solution is −3 b. 1 c. 0 d. 3
View SolutionFor solving each pair of equations, in this exercise, use the method of elimination by equation coefficients :
y = 2x - 6
y = 0
View Solution
