In Fig.
 diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : ar (DOC)=ar (AOB).Solution in Punjabi
diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : ar (DOC)=ar (AOB).Solution in Punjabi
Step by step video & image solution for In Fig. diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : ar (DOC)=ar (AOB). by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
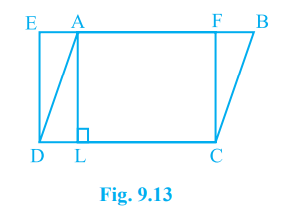
In Fig. 9.13, ABCD is a parallelogram and EFCD is a rectangle. Also, AL⊥DC. Prove that (i) ar (ABCD) = ar (EFCD) (ii) ar (ABCD) = DC × AL
View SolutionGiven an arc of a circle, complete the circle.
View SolutionIn Fig 9.25 ,diagonals AC and BD of quadrilateral ABCD intersect at O such that OB= OD.If AB=CD, then show that
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionIn the figure above (not to scale), ¯¯¯¯¯¯AB⊥¯¯¯¯¯¯CDandAD is the bisector of ∠BAE.AB=3cm and AC=5cm. Find CD.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View SolutionIn the figure (not to scale) AB = AC and BD = CD. Find ∠ADB
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C15_E05_003_Q01.png" width="80%"gt
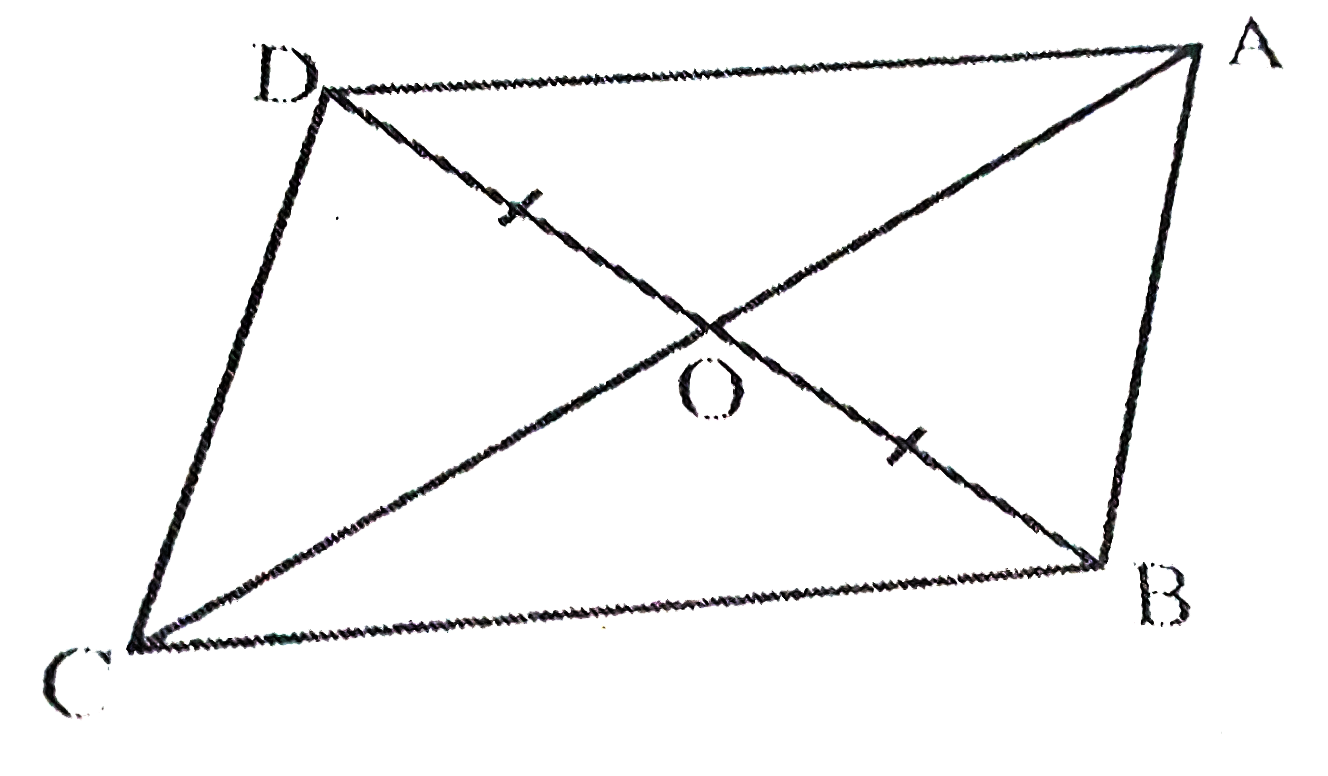
View Solutionआकृति में चतुर्भुज ABCD के विकर्ण AC और BD परस्पर बिंदु O पर इस प्रकार प्रतिच्छेद करते हैं कि OB=OD है । यदि AB=CD है , तो दर्शाइए कि
(i) ar(DOC)=ar(AOB)
(ii) ar(DCB)=ar(ACB)
(iii) DA∣∣CB या ABCD एक समांतर चतुर्भुज है ।
View Solutionदिए गए चित्र में चतुर्भुज ABCD के विकर्ण AC और BD परस्पर बिंदु O पर इस प्रकार प्रतिच्छेद करते है की OB = OD है|यदि AB =CD है ,तो दर्शाइए की
(i)ar(DOC)=ar(AOB)
(ii)ar(DCB)=ar(ACB)
(iii)DA∣∣CB या ABCD एक समांतर चतुर्भुज है|
(##KCSHINMATIXC11SLV028_Q01##)
View SolutionIn the given figure, diagonals AC and BD of quadrilateral ABCD interset at O such that OB = OD. If AB = CD, then show that :
(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA || CB or ABCD is a parallelogram .
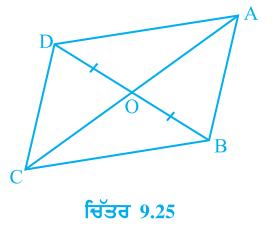
View Solutionਚਿੱਤਰ 9.25 ਵਿੱਚ, ਚਤੁਰਭੁਜ ABCD ਦੇ ਵਿਕਰਣ AC ਅਤੇ BD ਆਪਸ ਵਿਚ ਬਿੰਦੂ O ਤੇ ਇਸ ਤਰ੍ਹਾਂ ਕੱਟਦੇ ਹਨ ਕਿ OB=OD ਹੈ। ਜੇਕਰ AB=CD ਹੋਵੇ ਤਾਂ ਦਰਸਾਓ ਕਿ ar (DOC) = ar (AOB) [ਸੰਕੇਤ:- D ਅਤੇ B ਤੋਂ AC ਉੱਤੇ ਲੰਬ ਖਿਚੋ।]
View Solution
