Let P and Q be any two points on the lines represented by 2x−3y=0 and 2x+3y=0 respectively. If the area of triangle OPQ (where O is origin) is 5, then which of the following is not the possible equation of the locus of mid-point of PO?
4x2−9y2+15=0
4x2−9y2−30=0
9x2−4y2−30=0
4x2+9y2−30=0
The correct Answer is:B
We have, Area (ΔOPQ)=12∣∣
∣
∣
∣∣001a2a31b−2b31∣∣
∣
∣
∣∣=5 (Given)
⇒4ab3=±10
So, 4ab=±30 ......(i)
Aslo 2h=a+b ......(ii)
and 2k=2a−2b3⇒a−b=3k ....(iii)
As 4ab=(a+b)2−(a−b)2⇒±30=4h2−9k2 [Using (i), (ii) and (iii)]
So required locus is 4x2−9y2=±30
Topper's Solved these Questions
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART III : MATHEMATICS (SECTION-2)
5 Videos
- INVERSE TRIGONOMETRYBOOK - VMC MODULES ENGLISHCHAPTER - INVERSE TRIGONOMETRYEXERCISE - JEE ADVANCED ( ARCHIVE )
10 Videos
- JEE MAIN REVISION TEST - 30 | JEE -2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 30 | JEE -2020EXERCISE - MATHEMATICS
25 Videos
Similar Questions
A planet of mass m moves along an ellipse around the sun (mass M) so that its maxima and minimum distances from the sun are equal to r1 and r2 respectively. The angular momentum of this plane relative to the centre of the sun is m√PGMr1r28(r1+r2)
View SolutionTwo regression lines are represented by 2x+3y−10=0 and 4x+y−5=0
Find the line of regression of y on x.
View Solution
Knowledge Check
If P is the point (1,0) and Q is any point on the parabola y2=8x then the locus of mid - point of PQ is
AP1 lies on C2andQ1 lies on C1BPQ≥min{PP1,QQ1}Cpoint P0 on C1 such that P0Q0≤PQ for all pairs of points (P,Q) is (13,109)Dpoint Q0 on C2 such that P0Q0≤PQ for all pairs of points (P,Q) is (109,13)
If O is the origin and Q is a variable, point on x2=4y, find the locus of the mid-point of OQ.
View SolutionIf O is the origin and Q is a variable point on y2=x. Find the locus of the mid point of OQ.
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
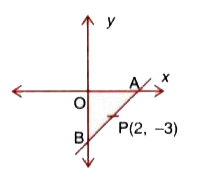
equation of line AB.
View SolutionA line is drawn through the point (1, 2) to meet the coordinate axes at P and Q such that it forms a triangle OPQ, where O is the origin. If the area of the triangle OPQ is least, then the slope of the line PQ is
View Solutionlet P be the point (1,0) and Q be a point on the locus y2=8x. The locus of the midpoint of PQ is
View SolutionThe tangent at any point P on y2=4x meets x-axis at Q, then locus of mid point of PQ will be
View SolutionLet the line y=mx intersects the curve y2=x at P and tangent to y2=x at P intersects x-axis at Q. If area (△OPQ) = 4, find m(m>0).
View Solution
