A bullet is fired at an angle of 15∘ with the horizontal and it hits the ground 3 km away. Can we hit a target at a distance of 7 km by just adjusting its angle of projection ?
To solve the problem, we need to determine whether a bullet fired at an angle of 15 degrees can hit a target located 7 km away by adjusting its angle of projection. We will use the physics of projectile motion to find the maximum range achievable by the bullet.
1. Understanding the Problem:
- A bullet is fired at an angle of 15∘ and hits the ground 3 km away.
- We need to find out if it can hit a target at a distance of 7 km by adjusting the angle of projection.
2. Using the Range Formula for Projectile Motion:
- The range R of a projectile is given by the formula:
R=u2sin(2θ)g
where:
- u = initial velocity,
- θ = angle of projection,
- g = acceleration due to gravity (approximately 10m/s2).
3. Calculating Initial Velocity:
- Given that the bullet hits the ground at a distance of 3 km (or 3000 m) when fired at 15∘:
3000=u2sin(30∘)g
- Since sin(30∘)=12, we can rewrite the equation as:
3000=u2⋅1210
- Simplifying gives:
3000=u220
- Therefore:
u2=3000×20=60000m2/s2
4. Finding Maximum Range:
- The maximum range Rmax occurs at an angle of 45∘:
Rmax=u2g
- Substituting the value of u2:
Rmax=6000010=6000m=6km
5. Conclusion:
- The maximum range achievable by the bullet is 6 km.
- Since the target is located at 7 km, it is not possible to hit the target by just adjusting the angle of projection.
Final Answer:
No, it is not possible to hit a target at a distance of 7 km by just adjusting the angle of projection.
- A bullet is fired at an angle of 15∘ and hits the ground 3 km away.
- We need to find out if it can hit a target at a distance of 7 km by adjusting the angle of projection.
- The range R of a projectile is given by the formula:
R=u2sin(2θ)g
where:
- u = initial velocity,
- θ = angle of projection,
- g = acceleration due to gravity (approximately 10m/s2).
- Given that the bullet hits the ground at a distance of 3 km (or 3000 m) when fired at 15∘:
3000=u2sin(30∘)g
- Since sin(30∘)=12, we can rewrite the equation as:
3000=u2⋅1210
- Simplifying gives:
3000=u220
- Therefore:
u2=3000×20=60000m2/s2
- The maximum range Rmax occurs at an angle of 45∘:
Rmax=u2g
- Substituting the value of u2:
Rmax=6000010=6000m=6km
- The maximum range achievable by the bullet is 6 km.
- Since the target is located at 7 km, it is not possible to hit the target by just adjusting the angle of projection.
No, it is not possible to hit a target at a distance of 7 km by just adjusting the angle of projection.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - SOLVED EXAMPLES20videos
Motion in Straight Line
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - PRACTICE EXERCISE80videos
Motion in Straight Line
- BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE52videos
MOTION IN A STRAIGHT LINE & PLANE
- BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ2videos
Motion in Two Dimensions
Similar Questions
A bullet fired at an angle of 30∘ with the horizontal hits the ground 3.0 km away. By adjusting its angle of projection, can one hope to hit a target 5.0 km away? Assume the muzzle speed to be fixed and neglect air resistance.
View SolutionA bullet fired at an angle of 30∘ with the horizontal hits the ground 3.0 km away. By adjusting its angle of projection, can one hope to hit a target 5.0 away? Assume the muzzle speed to be fixed and neglect air resistance.
View Solution
Knowledge Check
- Question 1 - Select One
A particle is thrown at an angle of 15∘ with the horizontal and the range is 1.5 km. What is the range when it is projected at 45∘ ?
A1.5 kmB6 kmC4.5 kmD3 km - Question 2 - Select One
A body is thrown with a velocity of 9.8 m/s making an angle of 30∘ with the horizontal. It will hit the ground after a time
A3 sB2sC1.5 sD1 s
A particle aimed at a target projected with an angle 15∘ with the horizontal is short of the target by 10m .If projected with an angle of 45∘ is away from the target by 15m then the angle of projection to hit the target is
View SolutionA projectile is fired at an angle of 45∘ with the horizontal. Elevation angle of the projection at its highest point as seen from the point of projection is
View SolutionA bullet fired at an angle of 60∘ with the vertical hits the levelled ground at a distance of 200 m . Find the distance at which the bullet will hit the ground when fired at angle of 30∘. (with same speed).
View SolutionA ball is projected from a point on the floor wilth a speed of 15 m/s at an angle of 600 with the horizontal. Will ilt hit a vertical wall 5 m away from the point of projection and perpendiculaer to the plane of projection without and perpendicular to the plane of projection without hitting the floor? will the answer differ if the wall is 22 m away?
View SolutionPoints A and C are on the horizontal ground and A and B are in same vertical plane at a distance of 1500m. Simultaneously bullets are fired from A,Bll and C and they collide at O. The bullets at B is fired at an angle of 30∘with horizontal towards the ground iat velocity 100m/s. the bullet at C is projected vertically upwards at velocity of 100m/s. the bullet projected from A reaches its maximum height at O.
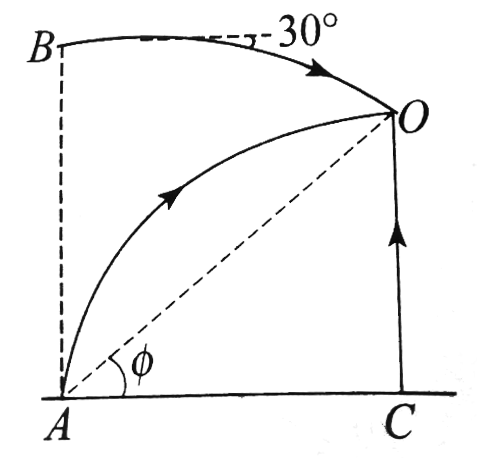
Find the elevation angle ∠θ=∠OAC:View SolutionPoints A and C are on the horizontal ground and A and B are in same vertical plane at a distance of 1500m. Simultaneously bullets are fired from A,Bll and C and they collide at O. The bullets at B is fired at an angle of 30∘with horizontal towards the ground iat velocity 100m/s. the bullet at C is projected vertically upwards at velocity of 100m/s. the bullet projected from A reaches its maximum height at O.
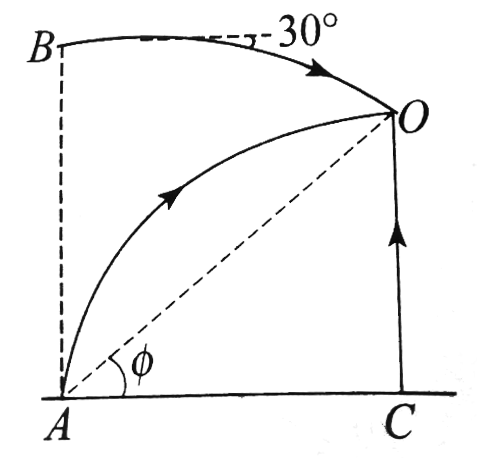
Find the velocity of bullet at A:View Solution
