A parallel plate capacitor of capacitance has spacing between two plates having area The region between the plates is filled with dielectric layers, parallel to its plates, each with thickness δ=dN. The dielectric constant of the mth layer is Km=K(1+mN). For a very large N(>103), the capacitance Cisα(K∈0Adln2). Find the value of α.[∈0 is the permittivity of free space]
The correct Answer is:1.00
To solve the problem, we need to determine the value of α in the expression for the capacitance of a parallel plate capacitor filled with multiple dielectric layers. Let's break down the steps systematically.
1. Understanding the Configuration:
- We have a parallel plate capacitor with a total separation D between the plates.
- The space between the plates is filled with N dielectric layers, each of thickness δ=DN.
- The dielectric constant of the mth layer is given by Km=K(1+mN).
2. Capacitance of Each Layer:
- The capacitance Cm of the mth dielectric layer can be expressed as:
Cm=Kmϵ0Aδ=K(1+mN)ϵ0ADN=K(1+mN)Nϵ0AD
3. Reciprocal of Capacitance:
- Since the capacitors are in series, the reciprocal of the total capacitance Ceq is the sum of the reciprocals of the individual capacitances:
1Ceq=N∑m=11Cm
- Substituting the expression for Cm:
1Ceq=N∑m=1DK(1+mN)Nϵ0A
4. Simplifying the Summation:
- The summation can be rewritten as:
1Ceq=DKNϵ0AN∑m=111+mN
- Changing the variable x=mN gives:
N∑m=111+mN≈N∫1011+xdx
- Evaluating the integral:
∫1011+xdx=ln(2)
- Thus, the summation approximates to Nln(2).
5. Final Expression for Ceq:
- Substituting back, we get:
1Ceq=Dln(2)Kϵ0A
- Therefore, the equivalent capacitance is:
Ceq=Kϵ0ADln(2)
6. Comparing with Given Expression:
- The problem states that Ceq=αKϵ0ADln(2).
- By comparing both expressions, we find:
α=1
Conclusion
The value of α is 1.
- We have a parallel plate capacitor with a total separation D between the plates.
- The space between the plates is filled with N dielectric layers, each of thickness δ=DN.
- The dielectric constant of the mth layer is given by Km=K(1+mN).
- The capacitance Cm of the mth dielectric layer can be expressed as:
Cm=Kmϵ0Aδ=K(1+mN)ϵ0ADN=K(1+mN)Nϵ0AD
- Since the capacitors are in series, the reciprocal of the total capacitance Ceq is the sum of the reciprocals of the individual capacitances:
1Ceq=N∑m=11Cm
- Substituting the expression for Cm:
1Ceq=N∑m=1DK(1+mN)Nϵ0A
- The summation can be rewritten as:
1Ceq=DKNϵ0AN∑m=111+mN
- Changing the variable x=mN gives:
N∑m=111+mN≈N∫1011+xdx
- Evaluating the integral:
∫1011+xdx=ln(2)
- Thus, the summation approximates to Nln(2).
- Substituting back, we get:
1Ceq=Dln(2)Kϵ0A
- Therefore, the equivalent capacitance is:
Ceq=Kϵ0ADln(2)
- The problem states that Ceq=αKϵ0ADln(2).
- By comparing both expressions, we find:
α=1
The value of α is 1.
Topper's Solved these Questions
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 42
1 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 43
1 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 40
1 Videos
- BASIC MATHEMATICS & VECTORSBOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable
50 Videos
- CURRENT ELECTRICITYBOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F
10 Videos
Similar Questions
A parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionTwo parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View Solution
Knowledge Check
A parallel plate capacitor with air between the plates has a capacitance of 10 pF. The capacitance, if the distance bgetween the plates is reduced by half and the space between tehm is filled with a substance of dielectric constant 4 is
A80pFB96pFC100pFD120pF
A parallel plate capacitor has plate area A and plate separation d. The space betwwen the plates is filled up to a thickness x (ltd) with a dielectric constant K. Calculate the capacitance of the system.

View SolutionA parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then

View SolutionA parallel plate capacitor of capacitance C is charged to a potential V and then disconnected with the battery. If separation between the plates is decreased by 50% and the space between the plates is filled with a dielectric slab of dielectric constant K=10. then
View SolutionThe ratio between masses of two planets is 3 : 5 and the ratio between their radii is 5 : 3. The ratio between their acceleration due to gravity will be
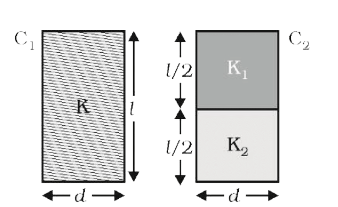
View SolutionTwo identical parallel plate (air) capacitors c1andc2 have capacitances C each. The space between their plates is now filled with dielectrics as shown. If two capacitors still have equal capacitance, obtain the relation betweenn dielectric constants k,k1andk2.
View SolutionThe capacitance of a capacitor between4/3 times its original value if a dielectric slab of thickness t=d/2 is inserted between the plates (d is the separation between the plates). What is the dielectric consant of the slab?
View SolutionThe capacitance of a capacitor between4/3 times its original value if a dielectric slab of thickness t=d/2 is inserted between the plates (d is the separation between the plates). What is the dielectric consant of the slab?
View Solution
