A small drop of water of surface tension σ is squeezed between two clean glass plates so that a thin layer of thickness d and area A is formed between them. If the angle of contact is zero, what is the force required to pull the plates apart?
σAd
2σAd
4σAd
8σAd
The correct Answer is:B
To find the force required to pull the two glass plates apart when a thin layer of water is squeezed between them, we can follow these steps:
Step 1: Understand the Problem
We have a thin layer of water with thickness d and area A between two glass plates. The surface tension of the water is denoted by σ, and the angle of contact is zero.
Step 2: Determine the Length in Contact
The length of the water film in contact with the plates can be determined from the area A and thickness d. Since the area A is given by:
A=length×width
and the width is equal to the thickness d, we can express the length L in contact as:
L=Ad
Step 3: Calculate the Total Length in Contact
Since there are two surfaces (top and bottom) in contact with the water film, the total length in contact is:
Total Length=2L=2×Ad=2Ad
Step 4: Calculate the Force Due to Surface Tension
The force F required to separate the plates is related to the surface tension and the total length in contact. The force due to surface tension is given by:
F=σ×Total Length
Substituting the total length we found in the previous step:
F=σ×2Ad
Final Answer
Thus, the force required to pull the plates apart is:
F=2σAd
We have a thin layer of water with thickness d and area A between two glass plates. The surface tension of the water is denoted by σ, and the angle of contact is zero.
The length of the water film in contact with the plates can be determined from the area A and thickness d. Since the area A is given by:
A=length×width
and the width is equal to the thickness d, we can express the length L in contact as:
L=Ad
Since there are two surfaces (top and bottom) in contact with the water film, the total length in contact is:
Total Length=2L=2×Ad=2Ad
The force F required to separate the plates is related to the surface tension and the total length in contact. The force due to surface tension is given by:
F=σ×Total Length
Substituting the total length we found in the previous step:
F=σ×2Ad
Thus, the force required to pull the plates apart is:
F=2σAd
Topper's Solved these Questions
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE MAIN (LEVEL - 1 )
31 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -1
90 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)
78 Videos
Similar Questions
A cylinder of height 20m is completely filled with water. The velocity of effux of water (∈ms−1) through a small hole on the side wall of the cylinder near its bottom is
View SolutionA vessel containing water is given a constant acceleration a towards the right,a straight horizontal path. Which of the following diagram represents the surface of the liquid.
View SolutionThe liquids shown in figure in the two arms are mercury (specific gravity =13.6) and water. If the difference of heights of the mercury columns is 2 cm, find the height of the water column.
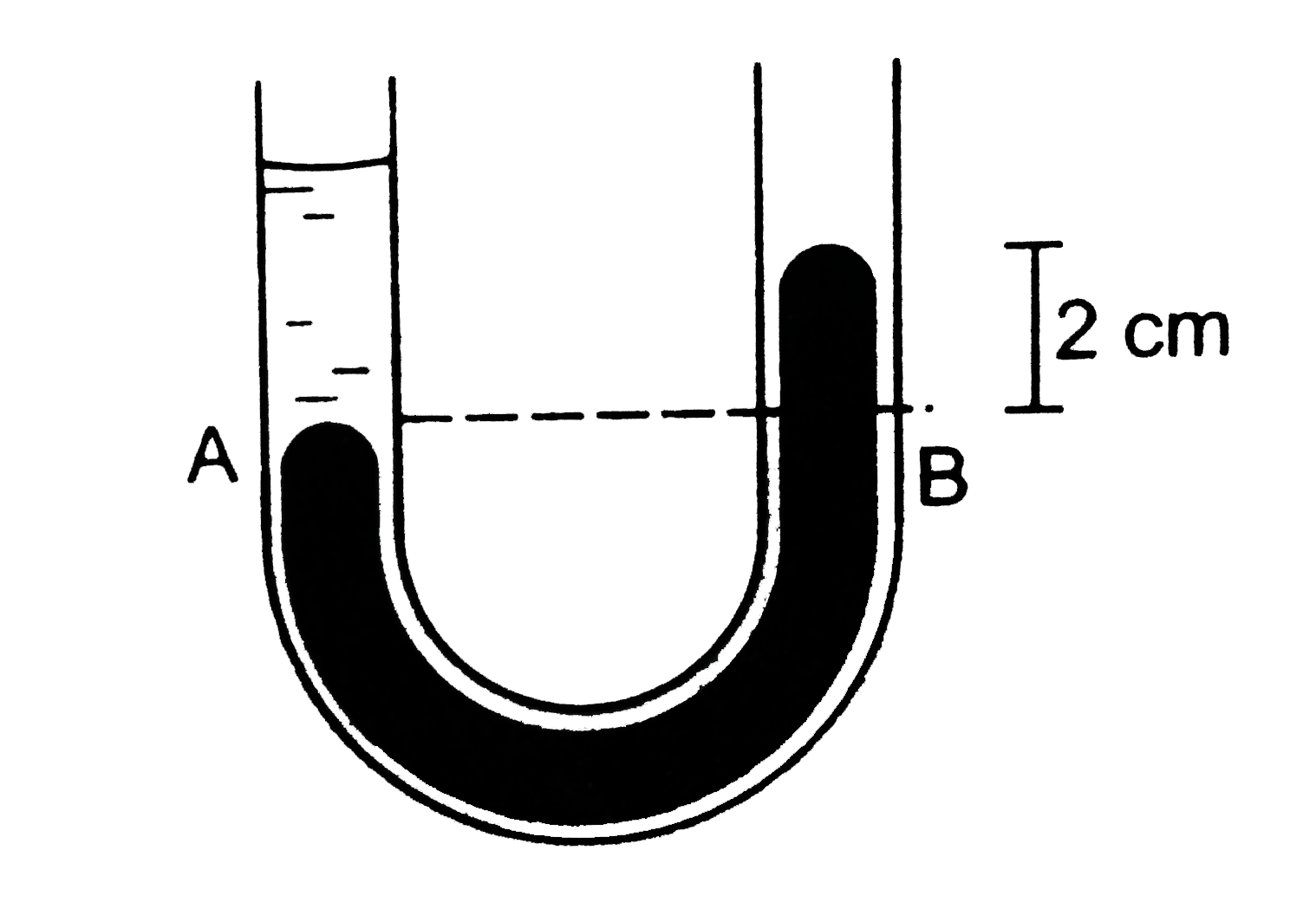 ,
,
View SolutionCoefficient of kinetic friction and coefficient of static friction between two blocks is μk and μs respectively. In general
View SolutionA circular loop of radius R carrying a current I is placed in a uniform magnetic field B perpendicular to the loop. The force on the loop is
View SolutionA small drop of water of surface tension S is squeezed between two clean glass plates so that a thin layar of thickness d and area A is formed between them. If the angle of contact is zero, what is the force required to pull the plates apart?
View SolutionA drop of water of mass m and density ρ is placed between two weill cleaned glass plates, the distance between which is d. What is the force of attraction between the plates?
(T= surface tension)
View SolutionA drop of liquid pressed between two glass plates spreads to a circle of diameter 10 cm. Thickness of the liquid film is 0.5 mm and surface tension is 70xx10^(-3)Nm^(-1) the force required to pull them apart is
View SolutionA drop of water of volume V is pressed between the two glass plates so as to spread to an area. A . If T is the surface tension, the normal force required to separate the glass plates is
View SolutionWater remains as drops on the polythene surface but, it forms a thin layer on the surface of a properly cleaned glass plate. Explain.
View Solutionकाँच की दो प्लेटों के बीच जल की एक बूँद को रखकर प्लेटों को आपस में दबा दिया जाता है जिससे उनके बीच 0.01 मिमी मोटी जल की फ़िल्म बन जाती है | यदि जल से भीगा प्लेटों का पृष्ठ क्षेत्रफल 10 सेमी2 है तो प्लेटों को अलग - अलग करने में कितना बल लगाना होगा ? दिया है : जल का पृष्ठ तनाव =7.2×10−2 न्यूटन/मीटर |
View SolutionThe length of one edge of a glass plate of thickness 0.2cm is 9.8cm .If this edge of the glass plate touches the surface of a liquid of surface tension 60 dyne/cm, then it is pulled down with a force of (Assume that angle of contact to be zero)
View SolutionThe length of one edge of a glass plate of thickness 0.2 cm is 9.8 cm. If this edge of the glass plate touches the surface of a liquid of surface tension 60 dyne/cm, then it is pulled down with a force of (Assume that angle of contact to be zero)
View SolutionTwo glass plates are separated by a thin layer of water of thickness 0.01 mm. If the wetted area corresponding to each plate be 30cm^(2) , calculate the force required to pull the plate apart. Surface tension of water is 75 dyne/cm.
View SolutionA drop of water of volume V is pressed between the two glass plates so as to spread on area A. If T is the surface tension, the normal force required to separate the glass plates is :
View Solution
