the position vector of the centre of mass lt
→
r cm of an asymmetric uniform bar of negligible area of cross - section as shown in figure is :
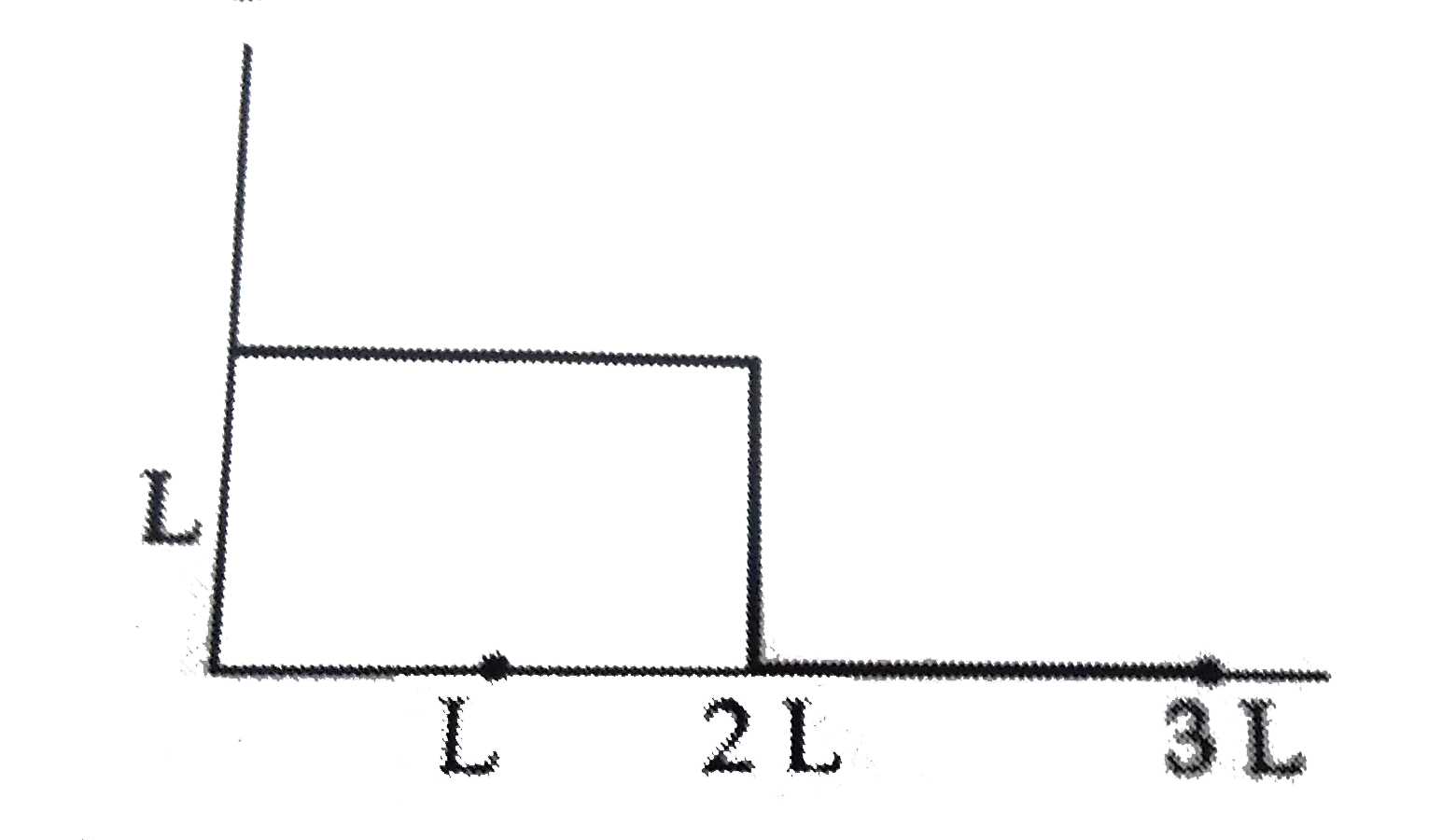
→rcm=38Lˆx+118Lˆy
→rcm=118Lˆx+38Lˆy.
→rcm=138Lˆx+58Lˆy.
→rcm=58Lˆx+138Lˆy.
The correct Answer is:B
m1=2m
m2=m
m3=m
We can assume the com of the three rods located at P,Q,R
Xcom=m1x1+m2x2+m3x3m1+m2+m3
=m4m(2L+2L+5L2)=13L8
Ycom=m1Y1+m2Y2+m3Y3m1+m2+m3
Ycom=2m×L+m×L/2+m×xOm1+m2+m3=m4m(2L+L/2)=5L8
 .
.
Topper's Solved these Questions
- JEE MAIN REVISION TEST 8 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST 8 (2020)EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST 5 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST 5 (2020)EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST- 16BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST- 16EXERCISE - PHYSICS (SECTION 2)
5 Videos
Similar Questions
Knowledge Check
The position of the centre of mass of a cube of uniform mass density will be at
Athe centre of one faceBthe centre of the interaction of diagonals of one face.Cthe geometric centre of the cubeDthe edge of a cube
Find the position of centre of mass of the uniform lamina shown in figure.

View SolutionMagnetic field strength at the centre of regular pentagon made of a conducting wire of uniform cross section area as shown in figure is:

View SolutionThe co-ordinates of the centre of mass of the system as shown in figure are
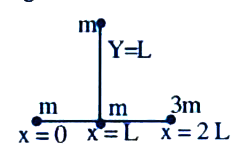
View SolutionThe distance of center of mass from the center of a uniform disc of radius R. if a circular plate is removed from the disc as shown in figure will be (C is centre of complete disc)
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
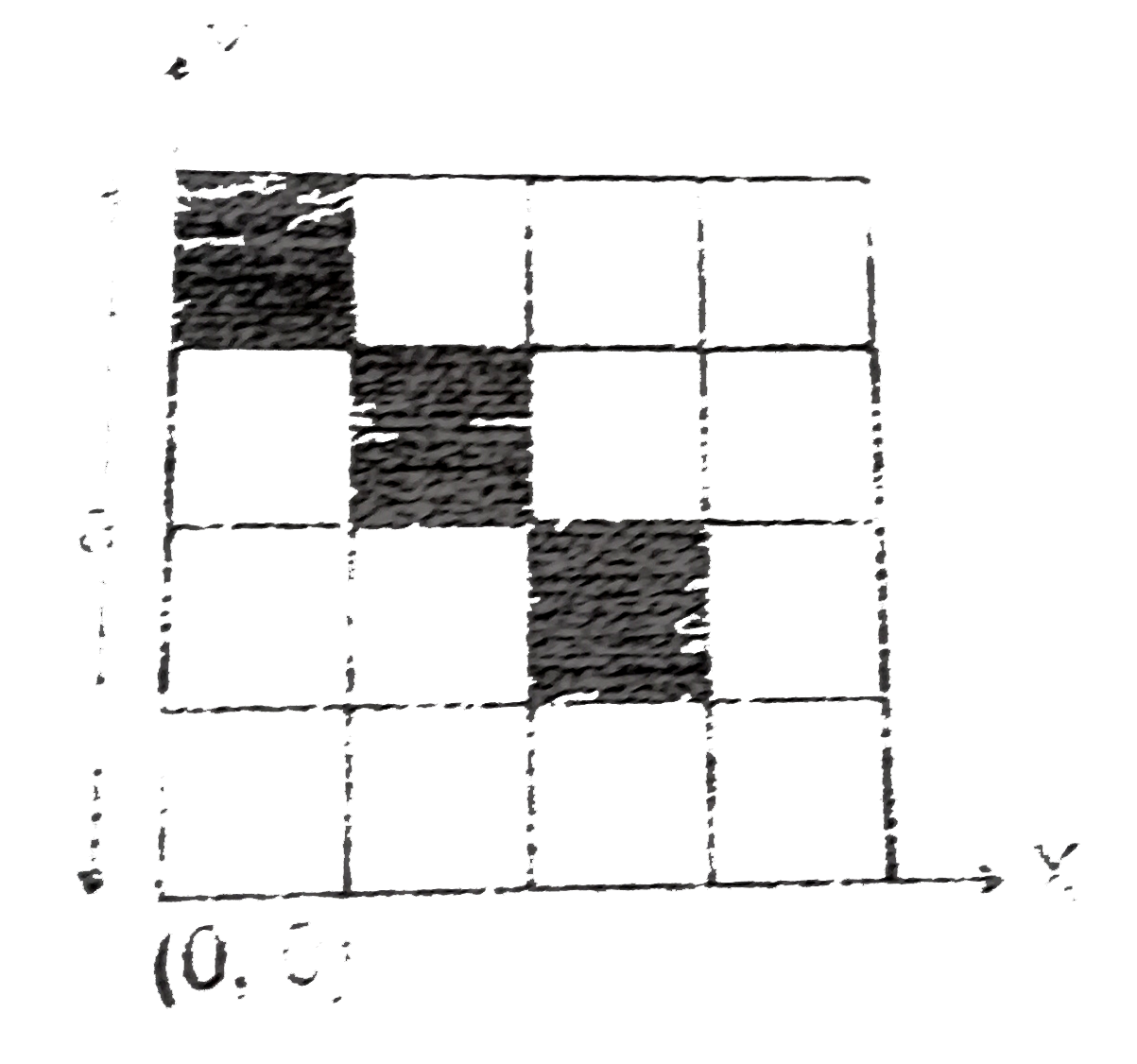
View SolutionFrom a uniform square plate the shaped portions are removed as shown in figure. The coordinates of centre of mass of the remaining plate are x,y Axes and origon are shown in figure.
View SolutionResistance of a conductor of length l and area of cross-section A is R. If its length is doubled and area of cross-section is halved, then find its new resistance.
View Solution


 .
.