0.5 moles of gas A and x moles of gas B exert a pressure of 200 Pa in a container of volume 10m3 at 1000K. Given R is the gas constant in jk−1 ,x is :
4+R2R
2R4−R
2R4+R
4−R2R
The correct Answer is:D
To solve the problem, we will use the ideal gas equation, which is given by:
PV=nRT
Where:
- P = Pressure (in Pascals)
- V = Volume (in cubic meters)
- n = Total number of moles of gas
- R = Gas constant (in Joules per Kelvin)
- T = Temperature (in Kelvin)
1. Identify the given values:
- Pressure, P=200Pa
- Volume, V=10m3
- Temperature, T=1000K
- Moles of gas A, nA=0.5moles
- Moles of gas B, nB=xmoles
2. Calculate the total number of moles:
n=nA+nB=0.5+x
3. Substitute the known values into the ideal gas equation:
PV=nRT
Substituting the known values:
200×10=(0.5+x)R×1000
4. Simplify the equation:
2000=(0.5+x)R×1000
Dividing both sides by 1000:
2=(0.5+x)R
5. Rearranging the equation to isolate x:
0.5+x=2R
Therefore,
x=2R−0.5
6. Express x in a simplified form:
x=2−0.5RR
This can also be written as:
x=4−R2R
Final Answer:
Thus, the value of x is:
x=4−R2R
- P = Pressure (in Pascals)
- V = Volume (in cubic meters)
- n = Total number of moles of gas
- R = Gas constant (in Joules per Kelvin)
- T = Temperature (in Kelvin)
- Pressure, P=200Pa
- Volume, V=10m3
- Temperature, T=1000K
- Moles of gas A, nA=0.5moles
- Moles of gas B, nB=xmoles
n=nA+nB=0.5+x
PV=nRT
Substituting the known values:
200×10=(0.5+x)R×1000
2000=(0.5+x)R×1000
Dividing both sides by 1000:
2=(0.5+x)R
0.5+x=2R
Therefore,
x=2R−0.5
x=2−0.5RR
This can also be written as:
x=4−R2R
Thus, the value of x is:
x=4−R2R
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two large glass containers of equal volume each hold 1 mole of gas. Container 1 is filled with hydrogen gas (2g/mol), and container 2 holds helium (4g/mol). If the pressure of the gas in container 1 equals the pressure of the gas in container 2, which of the following is true?
AThe temperature of the gas is container 1 is lower than the temperature of the gas is container 2.BThe temperature of the gas in container 1 is greater than the temperature of the gas in container 2.CThe value of R for the gas in container 1 is 12 the value of R for the gas is container 2.DThe rms speed of the gas molecules in container 1 is greater than the rms speed of the gas molecules in container 2.
The pressure of a gas is 100 k Pa. if it is compressed from 1 m3 to 10dm3 , find the work done .
View SolutionCalculate the moles of an ideal gas at pressure 2atm and volume 1L at a temperature of 97.5K
View SolutionContainer A holds an ideal gas at a pressure 1×105 Pa and at 300 K. Container B whose volume is 4 times the volume of A has the same ideal gas at 400 K and at a pressure of 5×105
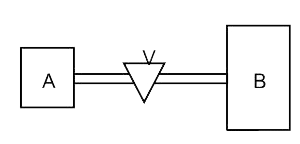
Before the valve V is opened, the ratio of number of moles of gas in A and B is :
View SolutionOne mole of an ideal gas is expanded from a volume of 3L to 5L under a constant pressure of 1 atm. Calculate the work done by the gas.
View SolutionContainer A holds an ideal gas at a pressure 1×105 Pa and at 300 K. Container B whose volume is 4 times the volume of A has the same ideal gas at 400 K and at a pressure of 5×105
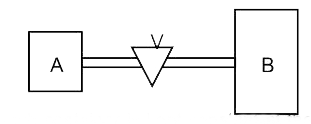
The valve V is adjusted to allow the pressure to equalize, but the temperature of each container is kept constant at the initial value, then the pressure in the two containers is :
View SolutionTwo moles of an ideal monoatomic gas at 5 bar and 300 K are expanded irreversibly up to a final pressure of 1 bar and 240 K against an external pressure of 0.5 bar. The work done by the gas is −xR. The value of x' is
(Here 'R' is gas constant)
View SolutionHalf mole of an ideal monoatomic gas is heated at constant pressure of 1 atm from 20∘C to 90∘C. Work done by gas is close to: (Gas constant R = 8.31 J/mol-K)
View Solution
