Question

The block of mass $m_1$ and $m_2$ are connected with a spring of netural length $l$ and spring constant $k$. The systeam is lying on a smoth horizontal surface. Initially spring is compressed by $x_0$ as shown in figure.

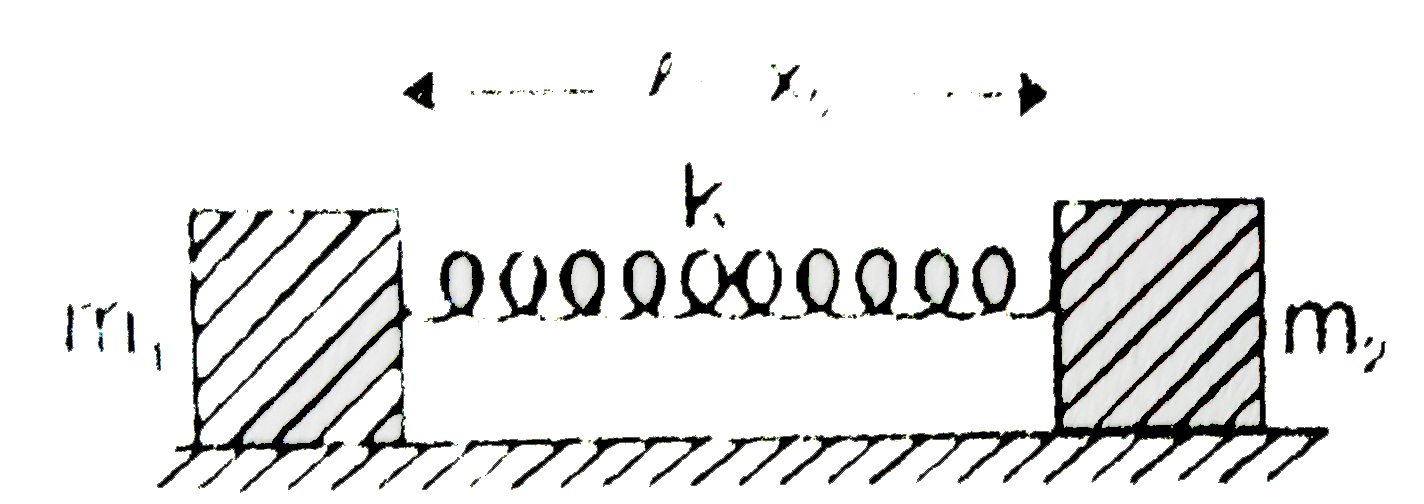
Show that the two blocks will perform $SHM$ about their equilibrium position. Also (a) find the time period, (b) find amplitude of each block and (c) length of spring as a funcation of time.

Explanation

(a) Hence both the blocks will be in equilibrium at the same time when spring is in its natural length. Let `EP_(1)` and `EP_(2)` be equilibrium positions of block `A` and `B` as shown in figure.

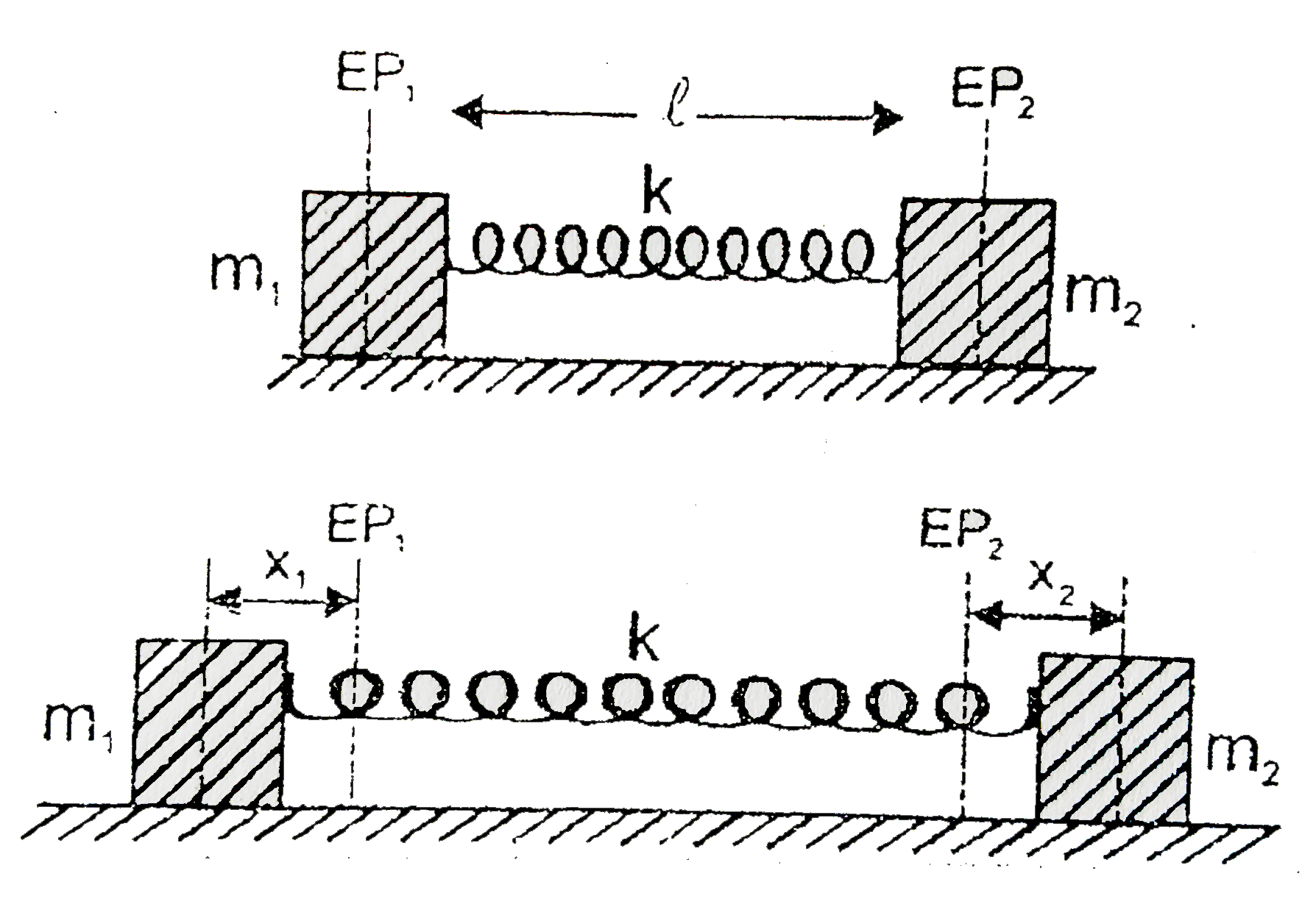
Let at any time during oscillations, blocks are at a distance of `x_(1)` and `x_(2)` from their equilibrium positions.
As no external force is acting on the spring on the spring block systeam
`:. (m_(1) + m_(2))Deltax_(cm) = m_(1)x_(1) - m_(2) x_(2) = 0` or `m_(1)x_(1) - m_(2) x_(2)`
for `1st` particle, force equation can be written as
`k(x_(1) + x_(2)) = -m_(1)(d^(2)x_(1))/(dt^(2))` or `k(x_(1) + (m_(1))/(m_(2))x_(1)) = - m_(1)a_(1)`
or, `a_(1) = -(k(m_(1) + m_(2)))/(m_(1)m_(2))x_(1) :. omega^(2) = (k(m_(1) + m_(2)))/(m_(1)m_(2))`
Hence, `T = 2pisqrt((m_(1)m_(2))/(k(m_(1) + m_(2)))) = 2psqrt((mu)/(K))` where `mu = '(m_(1)m_(2))/((m_(1) + m_(2))` which is known as reduced mass
Ans (a)
Similarly time period of `2`nd particle can be found. Both will be having the same time period.
(b) Let the amplitude of blocks be `A_(1)` and `A_(2)`.
`m_(1)A_(1) = m_(2)A_(2)`
By energy conservation,
`(1)/(2)k(A_(1) + A_(2))^(2) = (1)/(2) kx_(0)^(2)` or, `A_(1) + A_(2) = x_(0)`
or, `A_(1) + A_(2) = x_(0)` or, `A_(1) + (m_(1))/(m_(2))A_(1) = x_(0)`
or, `A_(1) = (m_(2)x_(0))/(m_(1) + m_(2))` similarly, `A_(2) = (m_(1)x_(0))/(m_(1) + m_(2))`
(c) Consider equilibrium position of `1`st particle as origin, i.e. `x = 0`.
`x` co-ordinate of particles can be written as
`x_(1) A_(1)cosomegat` and `x_(2) = l - A_(2)cosomegat`
length `= x_(2) - x_(1)`
`= l - (A_(1) + A_(2))cosomegat`