An air capacitor is completely charged upto the energy U and removed from battery. Now distance between plates is increased slowly by an external agent. If work done by external agent is 3U then ratio of final separation between the plates to the initial separation :
5
4
3
1.5
The correct Answer is:B
To solve the problem, we will analyze the situation step by step.
Step 1: Understand the Initial Conditions
- An air capacitor is charged to an energy U and then disconnected from the battery. This means that the charge Q on the capacitor remains constant.
Step 2: Work Done by the External Agent
- The work done by the external agent while increasing the separation between the plates is given as 3U.
Step 3: Relate Work Done to Change in Potential Energy
- The work done by the external agent is equal to the change in potential energy of the capacitor. We can express this as:
W=Uf−Ui
where Uf is the final potential energy and Ui is the initial potential energy.
Step 4: Substitute Known Values
- We know that the initial potential energy Ui=U and the work done W=3U. Therefore, we can write:
3U=Uf−U
Rearranging gives:
Uf=3U+U=4U
Step 5: Use the Formula for Potential Energy of a Capacitor
- The potential energy U of a capacitor is given by the formula:
U=Q22C
where C is the capacitance. For a parallel plate capacitor, the capacitance C is given by:
C=ε0Ad
where A is the area of the plates and d is the separation between the plates.
Step 6: Analyze the Change in Capacitance
- Since the charge Q remains constant and the separation d is increased, the new capacitance Cf can be expressed as:
Cf=ε0Adf
where df is the final separation.
Step 7: Relate Initial and Final Potential Energies
- The initial potential energy can be expressed as:
U=Q22Ci=Q2di2ε0A
and the final potential energy as:
Uf=Q22Cf=Q2df2ε0A
Step 8: Set Up the Ratio of Energies
- We know Uf=4U, thus:
Q2df2ε0A=4⋅Q2di2ε0A
This simplifies to:
df=4di
Step 9: Find the Ratio of Final to Initial Separation
- The ratio of the final separation df to the initial separation di is:
dfdi=4
Final Answer
The ratio of the final separation between the plates to the initial separation is 4.
---
- An air capacitor is charged to an energy U and then disconnected from the battery. This means that the charge Q on the capacitor remains constant.
- The work done by the external agent while increasing the separation between the plates is given as 3U.
- The work done by the external agent is equal to the change in potential energy of the capacitor. We can express this as:
W=Uf−Ui
where Uf is the final potential energy and Ui is the initial potential energy.
- We know that the initial potential energy Ui=U and the work done W=3U. Therefore, we can write:
3U=Uf−U
Rearranging gives:
Uf=3U+U=4U
- The potential energy U of a capacitor is given by the formula:
U=Q22C
where C is the capacitance. For a parallel plate capacitor, the capacitance C is given by:
C=ε0Ad
where A is the area of the plates and d is the separation between the plates.
- Since the charge Q remains constant and the separation d is increased, the new capacitance Cf can be expressed as:
Cf=ε0Adf
where df is the final separation.
- The initial potential energy can be expressed as:
U=Q22Ci=Q2di2ε0A
and the final potential energy as:
Uf=Q22Cf=Q2df2ε0A
Step 8: Set Up the Ratio of Energies
- We know Uf=4U, thus:
Q2df2ε0A=4⋅Q2di2ε0A
This simplifies to:
df=4di
- The ratio of the final separation df to the initial separation di is:
dfdi=4
The ratio of the final separation between the plates to the initial separation is 4.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.41
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.42
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.39
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
Knowledge Check
A parallel plate air capacitor is charged to a potential difference of V volts. After disconnecting the charging battery the distance between the plates of the capacitor is increased using an isulating handle. As a result the potential difference between the plates
AincreasesBdecreaseCdoes not chargeDbecomes zero
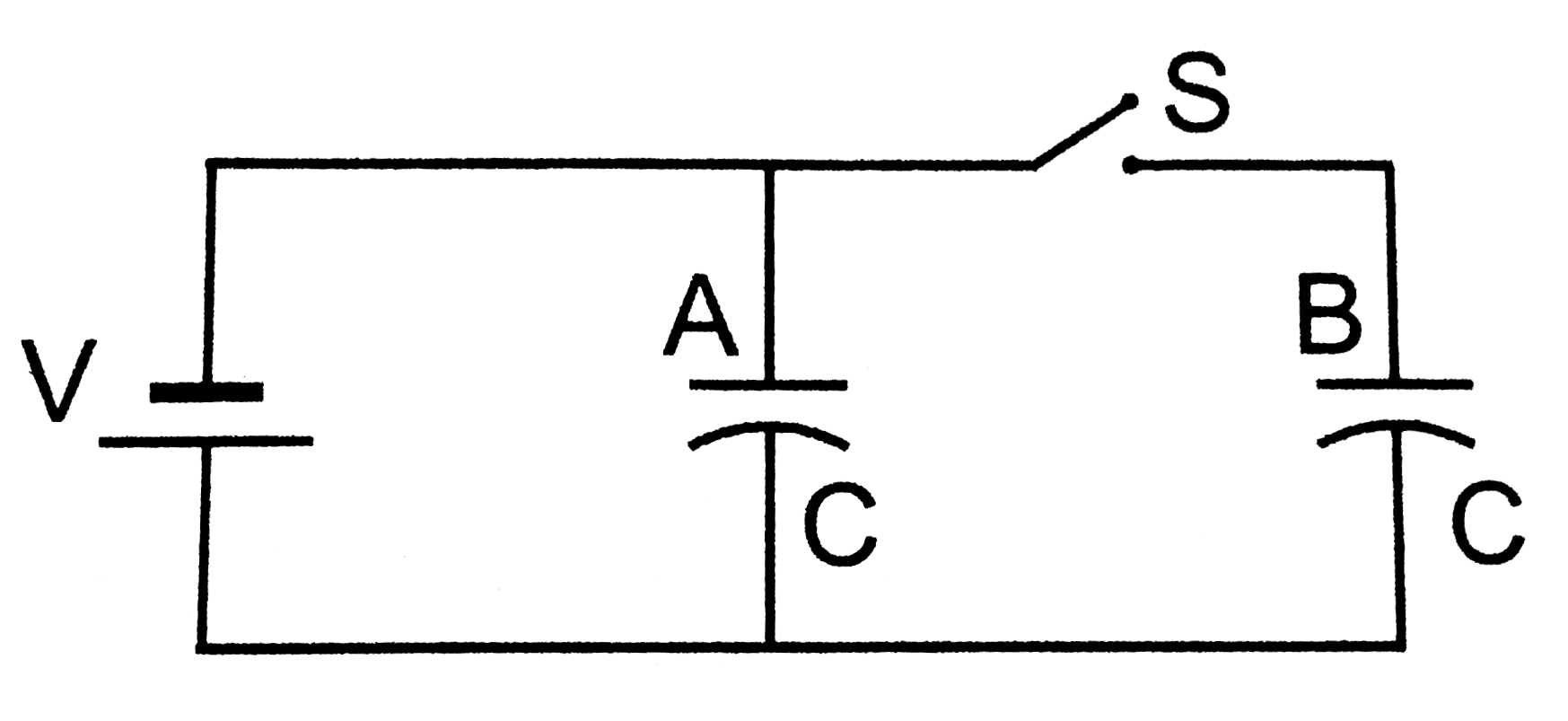
Figure shows two indentical pallel plate capacitors connected to a switch S. Initially ,the switch is closed so that the capacitors are completely charged .The switch is now opened and the free space between the plates of the capacitors is filled with a dielectric of dielectric constant 3. Find the ratio of the initial total energy stored in the capacitors to the final total energy stored.
View SolutionIn front of a uniformly charged infinite non-conducting sheet of surface charge density σ, a point charge q0 is shifted slowly from a distance a to b(b>a). If work done by external agent is W, then find out relation between the given parameters.
View SolutionAfter charging a capacitor the battery is removed. Now by placing a dielectric slab between the plates
View SolutionAnswer the following:
(a) When a parallel plate capacitor is connected across a dc battery, explain briefly how the capacitor gets charged.
(b) A parallel plate capacitor of capacitance 'C' is charged to 'V' volt by a battery. After some time the battery is disconnected and the distance between the plates is doubled. Now a slab of dielectric constant 1<k<2 is introduced to fill the space between the plates. How will the following be affected ?
(i) The electric field between the plates of the capacitor.
(ii) The energy stored in the capacitor. Justify your answer in each case.
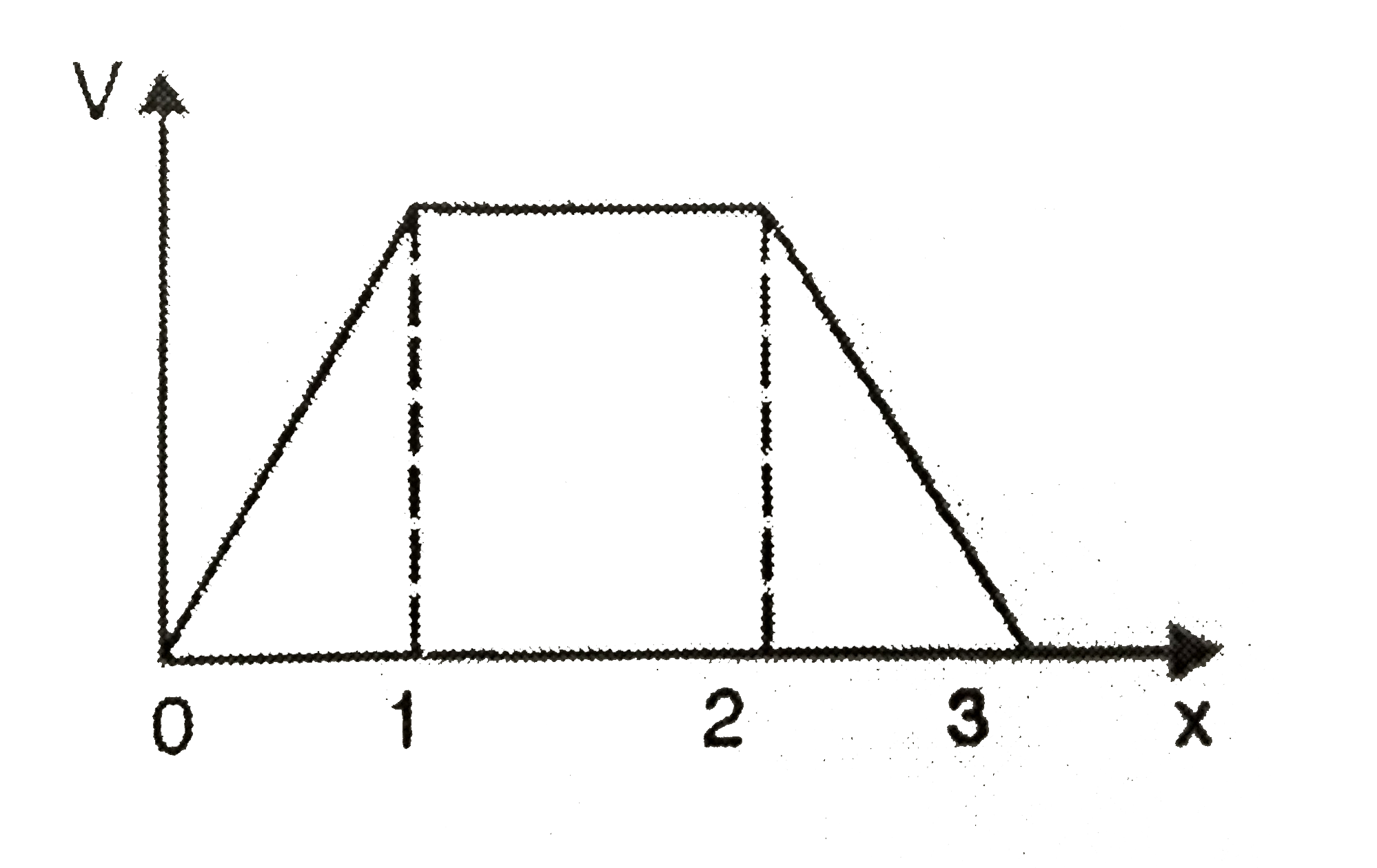
(c) The electric potential as a function of distance 'x' is shown in the figure. Draw a graph of the electric field E as a function of x.
View SolutionAn air-cored parallel plate capacitor having a capacitance C is charged by connecting it to a battery. Now, it is disconnected from the battery and a dielectric slab of dielectric constant K is inserted between its plates so as to completely fill the space between the plates. Compare :
(I) Initial and final capacitance
(ii) Initial and final charge.
(iii) Initial and final potential difference.
(iv) Initial an final electric field between the plates.
(v) Initial and final energy stored in the capacitor.
View SolutionA parallel plate air capacitor is charged to a potential difference of V volts. After disconnecting the charging battery the distance between the plates of the capacitor is increased using an isulating handle. As a result the potential difference between the plates
View SolutionA parallel plate air capacitor is charged to a potential difference of V volts. After disconnecting the charging battery the distance between the plates of the capacitor is increased using an isulating handle. As a result the potential difference between the plates
View Solution
