Two balls of same mass are released simultaneously from heights h & 2h from the ground level. The balls collide with the floor & stick to it. Then the velocity-time graph of centre of mass of the two balls is best represented by :
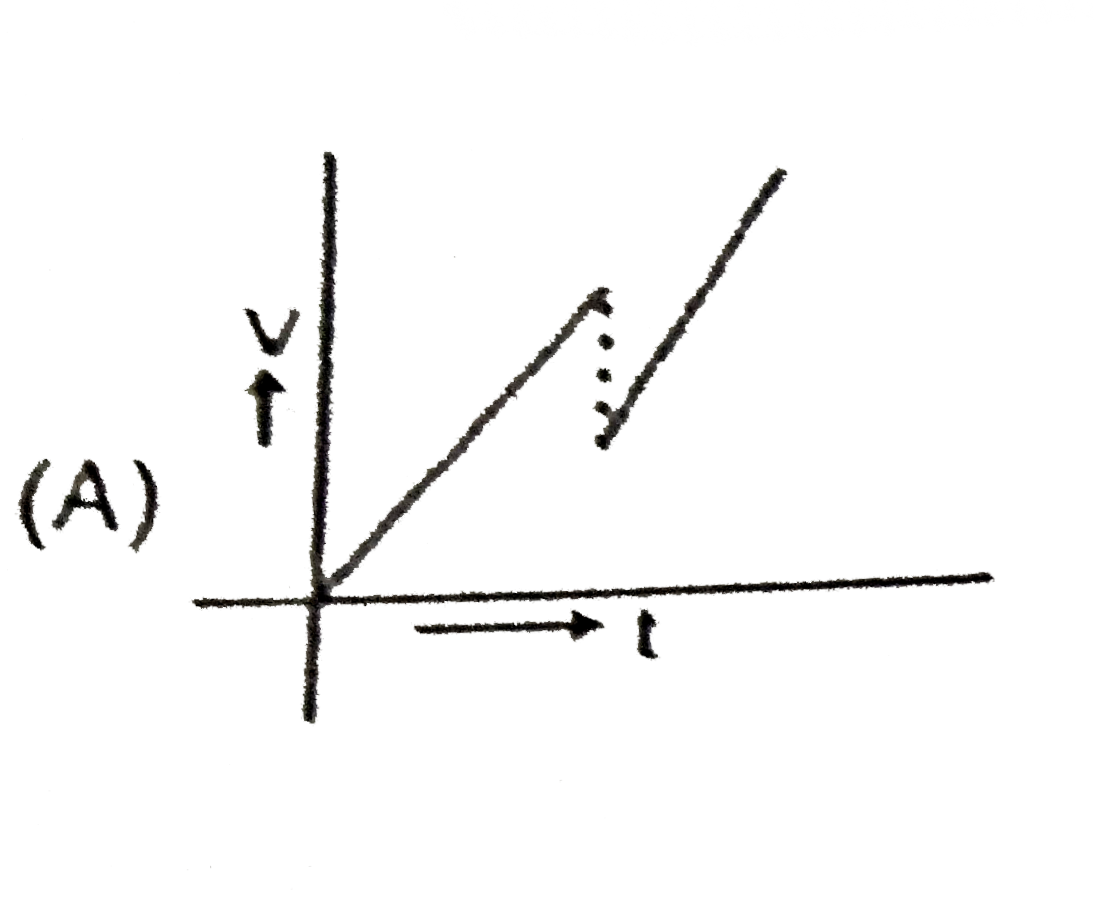
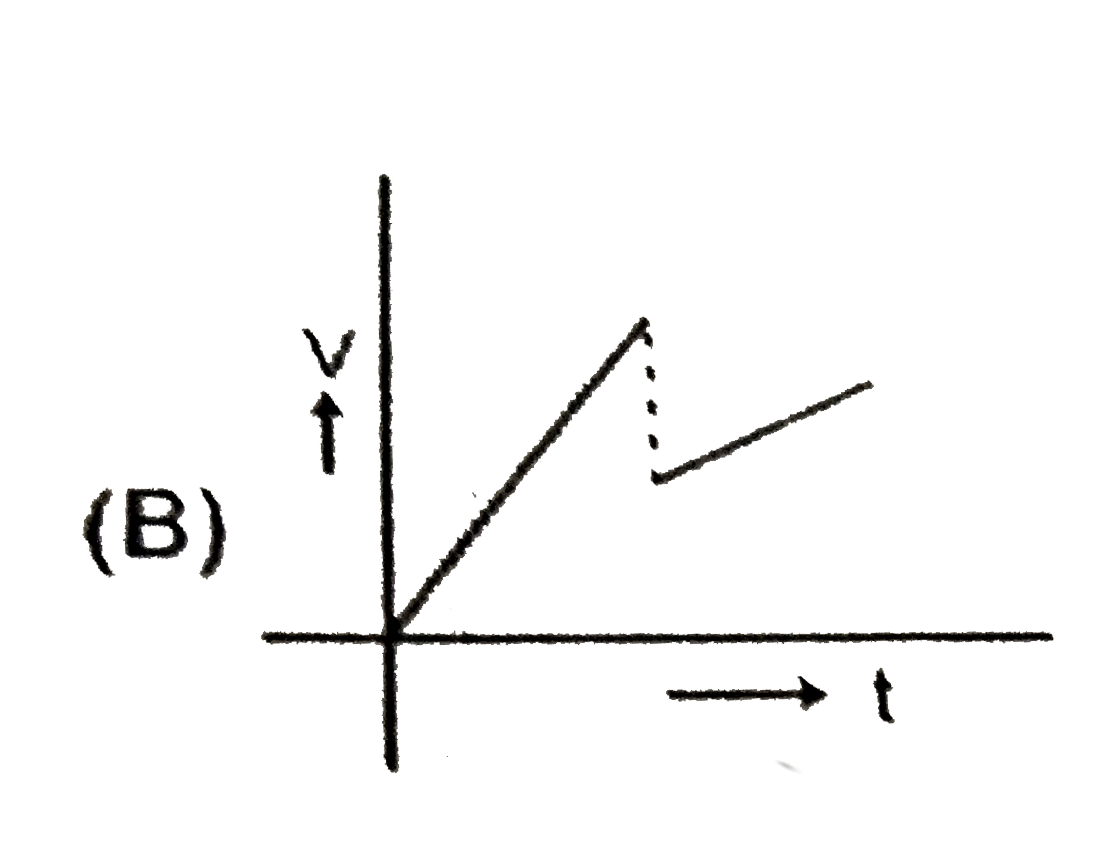
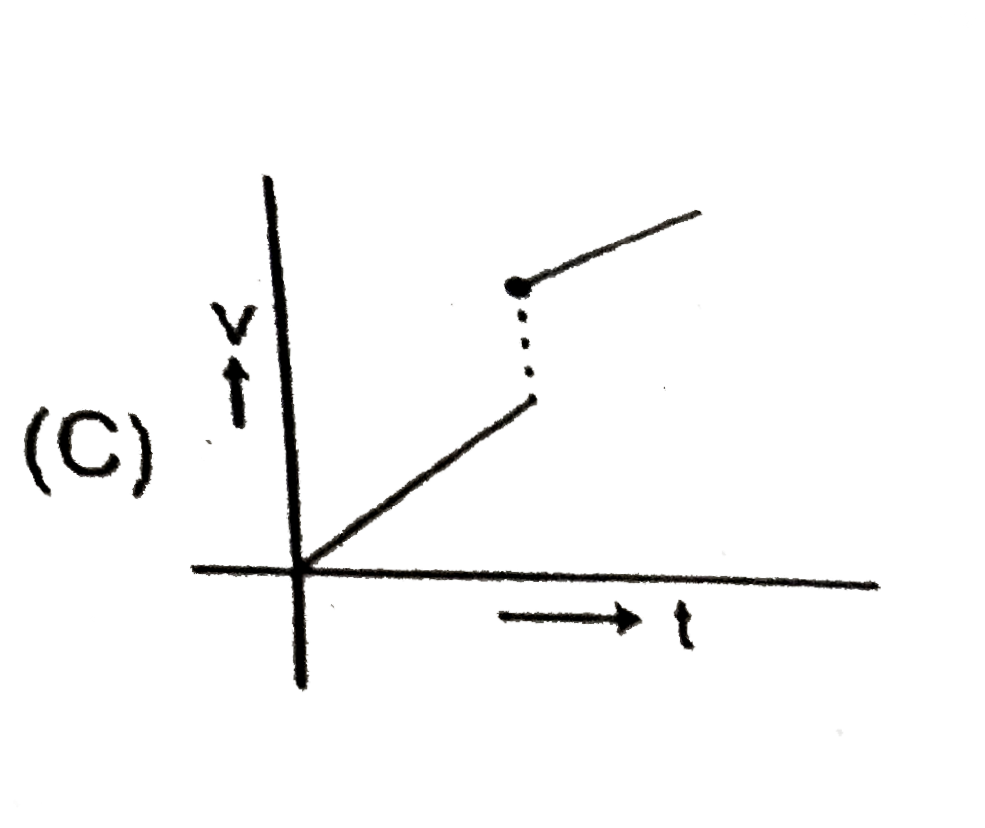
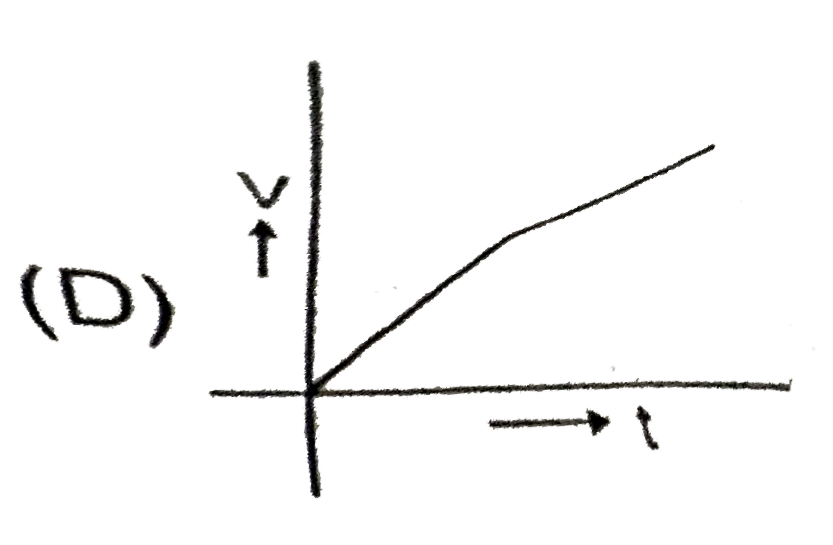
The correct Answer is:B
(B) As both the balls are released simultaneously, at any instant before the lower balls reaches the ground bothe have the same velocity,v=>i.e.,'v'vs.'t' is a straight line graph.
VCM=mv(t)+mv(t)2m=v(t),v(t) beingh the instantaneous velocity.
Just after the lower ball strikes ground and comes to rest :
VCM=mv(t)2m=v(t)2
i.e. the velocity suddently drops to half its value.
Hence graphs (A)&(B) are chosen.
After collision :
aCM=m(g)+m(0)m+m=g2
i.e. the slope ( of v−t curve ) should decrease to half. Hence (B) is the best option.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 51
7 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 52
7 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 49
6 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
53 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
Two balls are thrown simultaneously in air. The acceleration of the centre of mass of the two balls while in air
View SolutionTwo balls of equal mass are projected from a tower simultaneously with equal speeds. One at angle θ above the horizontal and the other at the same angle θ below the horizontal. The path of the center of mass of the two balls is
View SolutionA ball is thrown upwards with a speed u from a height h above the ground.The time taken by the ball to hit the ground is
View SolutionTwo balls are dropped from heights h and 2h respectively from the earth surface. The ratio of time of these balls to reach the earth is.
View SolutionTwo balls of same mass are dropped from the same height h, on to the floor. The first ball bounces to a height h/4, after the collision & the second ball to a height h/16. The impulse applied by the first & second ball on the floor are I1 and I2 respectively. Then :-
View SolutionA ball is projected upwards from a height h above the surface of the earth with velocity v. The time at which the ball strikes the ground is
View SolutionA ball is projected upwards from a height h above the surface of the earth with velocity v. The time at which the ball strikes the ground is
View SolutionA ball of mass 'm is released from the top of a smooth movable wedge of mass 'm'. When the ball collides with the floor, velocity is 'v'. Then the maximum height attained by the ball after an elastic collision with the floor is : (Nelect any edge at the lower end of the wedge)
View SolutionTwo identical balls are set into motion simultaneously from an equal height h. While ball A is thrown horizontally with velocity v, ball B is just released to fall by itself. Choose the alternative that best represents the motion of A and B with respect to an observer who moves with velocity v/2 with respect to the ground as shown in the figure.

View SolutionAn iron ball and a wooden ball of the same radius are released from a height ‘ h ’ in vacuum. The time taken by both of them to reach the ground is
View Solution
