A uniform magnetic field exists in a circular region of radius R centred at O. The field is perpendicular to the plane of paper and is strength varies with time as B=B0t. Find the induced electric field at a distance r from the centre for (i)r<R, (ii) r>R. Also, plot a graph between |E| and r for both the cases.
Induced electric field is given by
∫→E.Vec(dl)=−dϕBdt
(1) r lt R. Consider a ring of radius r (lt R) centered at O. The induced field at the periphery of the ring is circular such that
∮→E.→dl=E×2πr. As ϕB=(B0t)πr2
∴dϕBdt=B0πr2
∴E×2πr=−B0r2
E=−B0r2(⇒E∝r)
(the sign comes when clockwise electric field lines are developed)

(2) r gt R. Consider r ring of radius r ( gt R) centred at O. Again following the same procedure
∮→E.→dl=E×2πr
ϕB=(B0t)πR2 (as field is present only in rigion 0 lt r lt R)
dϕBdt=B0πR2
⇒E2πr=−B0πR2
E=−B0R22r (⇒E∝1r)

Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Try Yourself
42 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - A)
55 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
Similar Questions
A uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
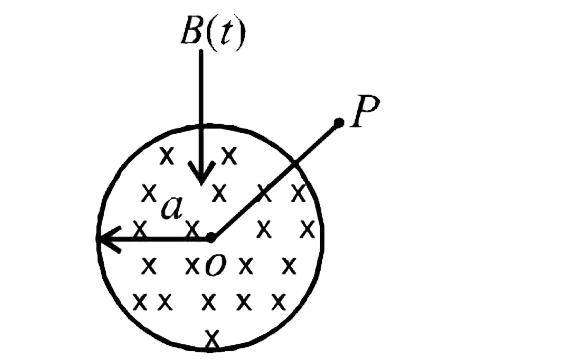
View SolutionA uniform but time varying magnetic field B(t) exist in a circular region of radius a and is directed into the plane of the paper as shown. The magnitude of the induced electric field at point P at a distance r form the centre of the circular region.

View Solution
Knowledge Check
There is a solid sphere of radius R having uniformly distributed charge throughout it. What is the relation between electric field E and distance r from the centre (r is less than R) ?
AE∝r−2BE∝r−1CE∝rDE∝r2
Figure shows a circular region of radius R in which uniform magnetic field B exists. The magnetic field is increasing at a rate dBdt. The induced electric field at a distance r from the centre for r<R is

View SolutionA time varying uniform magnetic field passes through a circular region of radius R. The magnetic field is directed outwards and it is a function of radial distance 'r' and time 't' according to relation B-B_(0)rt. The induced electric field strength at a radial distance R/2 from the centre will be.
View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).

View SolutionA conducting loop of radius R is present in a uniform magnetic field B perpendicular to the plane of the ring. If radius R varies as a function of time t, as R=R0+t. The emf induced in the loop is

View SolutionThe field due to a magnet at a distance R~ from the centre of the magnet is proportional
View SolutionThe flux of electric field through the circular region of radius r as shown in the figure is
_PHY_E05_291_Q01.png)
View SolutionA circular coil of radius R carries an electric current. The magnetic field due to the coil at a point on the axis of the coil located at a distance r from the centre of the coil, such that r>>R, varies as
View Solution
