A particle A is projected with an initial velocity of $60 m / s$ at an angle $30^{\circ}$ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of $50 m / s$ from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection $α$ of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. $(g = 10 m / s^{2})$

Video Solution

Text Solution

Verified by Experts

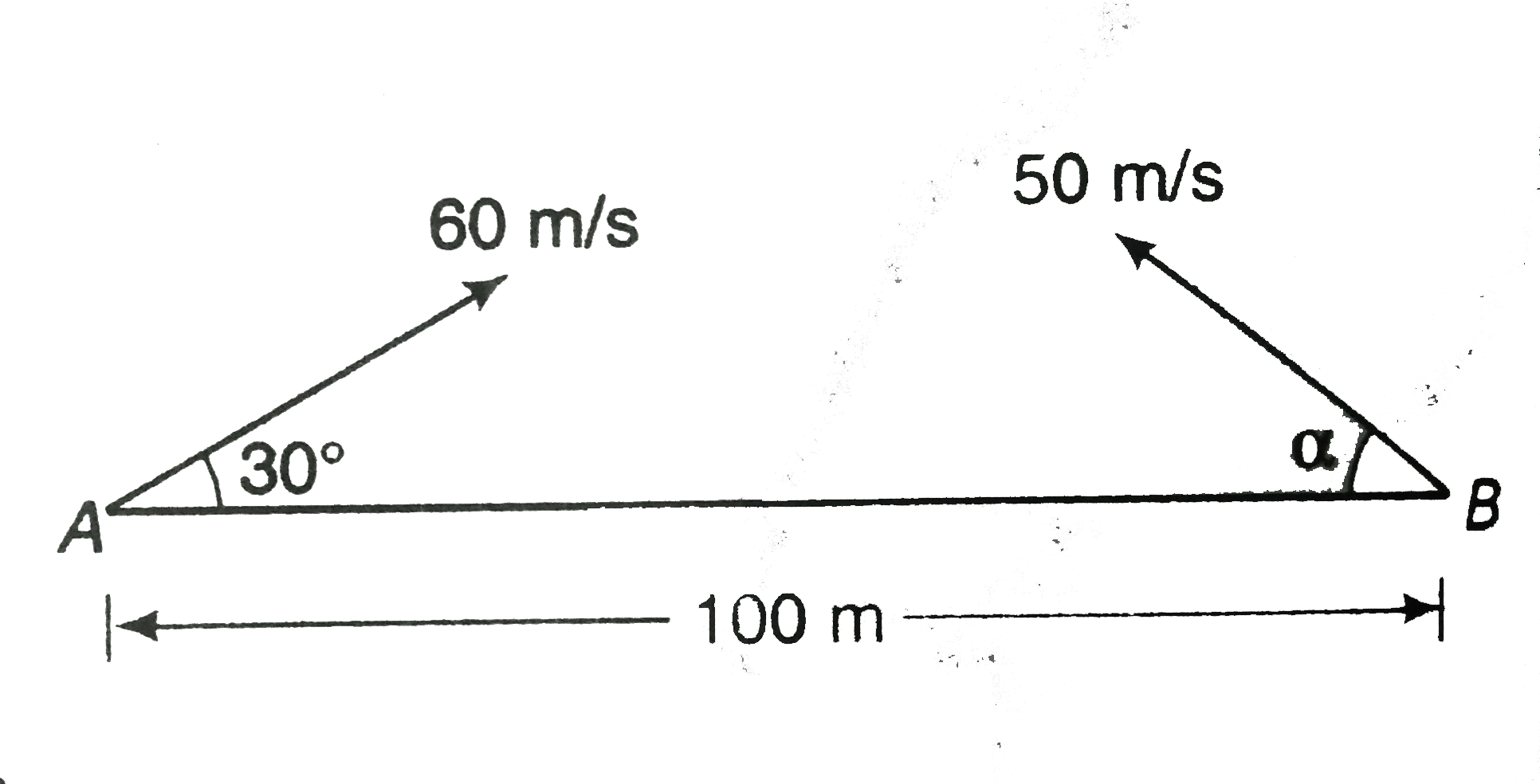
Taking horizontal as x-axis, vertical as y-axis and A as origin
${\to u}_{A} = (60 {cos 30}^{\circ}) ˆ i + (60 {sin 30}^{\circ} ˆ j$
$= 30 \sqrt{3} ˆ i + 30 ˆ j$
${\to u}_{B} = (- 50 cos α) ˆ i (50 sin α) ˆ j$
As both the particles are falling under gravity. their relative acceleration is zero.
Now initial velocity of second particle w.r.t first particle
${\to c}_{BA} = {\to v}_{B} - {\to v}_{A}$
$(- 30 \sqrt{3} - 50 cos α) ˆ i + (50 sin α - 30) ˆ j$
As acceleration of B w.r.t A is zero so B will appear to move in straight line with uniform speed. FOr collision to take palce, velocity , of B ,w,r,t A must be toward , A, i.e. y component of relative velocity must be zero.
$(50 sin α - 30) = 0$
$sin α = \frac{3}{5} \Rightarrow α = {sin}^{- 1} (\frac{3}{5})$
(ii) Time of colision
${\to d}_{A B} = {\to V}_{A B} (T)$
$- 100 ˆ i = (- 30 \sqrt{3} - 50 cos α) I ˆ i$
$or 100 = (30 \sqrt{3} + 50 \times \frac{4}{5}) T$
$or T = \frac{100}{(30 \sqrt{3} + 40)} = \frac{10}{3 \sqrt{3} + 4} = 1.09 s$

Updated on:21/07/2023

|

Class 12 PHYSICS MOTION IN A PLANE

Topper's Solved these Questions

Similar Questions

AAKASH INSTITUTE ENGLISH-MOTION IN A PLANE-Assignement section -J (Aakash Challengers Questions)

Home
Ask Doubt
Profile