A block of mass 0.1 kg attached to a spring of spring constant 400 N/m is putted rightward from x0=0 to x1=15 mm. Find the work done by spring force.
To find the work done by the spring force when a block is displaced from x0=0 to x1=15 mm, we can follow these steps:
Step 1: Understand the formula for work done by a spring
The work done by a spring when it is compressed or stretched is given by the formula:
W=−12kx2
where:
- W is the work done by the spring force,
- k is the spring constant,
- x is the displacement from the equilibrium position.
Step 2: Convert the displacement into meters
Since the displacement is given in millimeters, we need to convert it to meters:
x1=15 mm=15×10−3 m=0.015 m
Step 3: Substitute the values into the formula
Now we can substitute the values into the work done formula. The spring constant k is given as 400 N/m, and the displacement x is 0.015 m:
W=−12×400N/m×(0.015m)2
Step 4: Calculate (0.015)2
Calculating the square of the displacement:
(0.015)2=0.000225m2
Step 5: Substitute and calculate the work done
Now substitute (0.015)2 back into the equation:
W=−12×400×0.000225
W=−200×0.000225
W=−0.045J
Final Answer
The work done by the spring force is:
W=−0.045J
The work done by a spring when it is compressed or stretched is given by the formula:
W=−12kx2
where:
- W is the work done by the spring force,
- k is the spring constant,
- x is the displacement from the equilibrium position.
Since the displacement is given in millimeters, we need to convert it to meters:
x1=15 mm=15×10−3 m=0.015 m
Now we can substitute the values into the work done formula. The spring constant k is given as 400 N/m, and the displacement x is 0.015 m:
W=−12×400N/m×(0.015m)2
Calculating the square of the displacement:
(0.015)2=0.000225m2
Now substitute (0.015)2 back into the equation:
W=−12×400×0.000225
W=−200×0.000225
W=−0.045J
The work done by the spring force is:
W=−0.045J
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
60 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
45 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
a block of mass 0.1 kg attached to a spring of spring constant400Nm pulled horizontally from x=0 tox1=10mm. Find the work done by the spring force
View SolutionA block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View SolutionA block of mass 2kg is attached with a spring of spring constant 4000Nm−1 and the system is kept on smooth horizontal table. The other end of the spring is attached with a wall. Initially spring is stretched by 5cm from its natural position and the block is at rest. Now suddenly an impulse of 4kg−ms−1 is given to the block towards the wall.
Find the velocity of the block when spring acquires its natural length
View SolutionA block of mass 0.01 kg is attached to a spring of force constant 10−1Nm−1 . If the energy of oscillation is 10−5 J, find the angular frequency and amplitude of oscillation.
View SolutionA spring of spring constasnt 50 N/m is compressed from its natural position through 1 cm. Find the work done by the work done by the spring force on the agency compressing the spring.
View SolutionA block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

View SolutionA block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

View SolutionA block whose mass is 2 kg is fastened on a spring whose spring constant is 100 Nm−1 . It is pulled to a distance of 0.1 m from over a frictionless surface and is released at t=0. Calculate the kinetic eneryg of the block when it is 0.05 m away from its mean position.
View SolutionA small block of mass 2 kg is placed on a bigger block of mass 4 kg which is attached to horizontal spring of spring constant K=500N/m as shown in figure, coefficient of friction between block is 0.2 .Maximum amplitude of system so that there is no relative slipping between blocks.
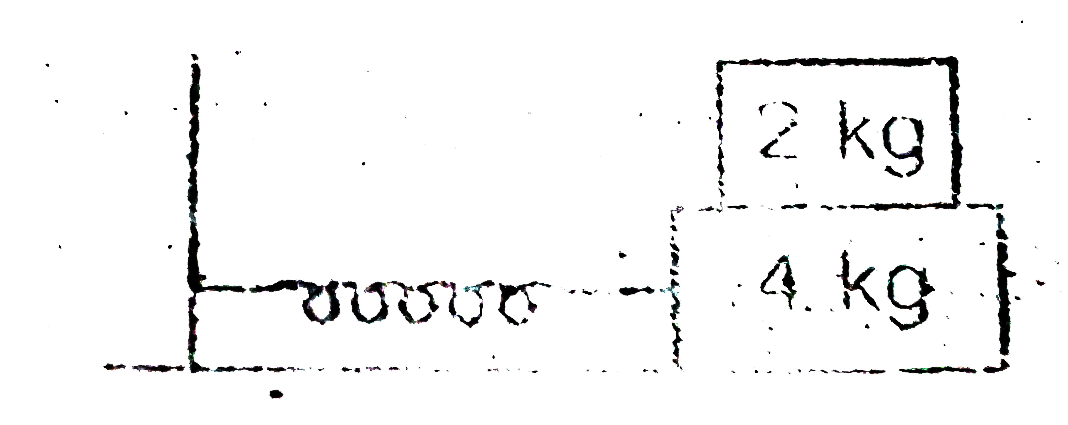
View SolutionA block of mass 0.18kg is attached to a spring of force-constant 2N/m. The coefficient of friction between the block and the floor is 0.1 Initially the block is at rest and the spring is un-stretched. An impulse is given to the block as shown in the figure. The block slides a distance of 0.06m and comes to rest for the first time. The initial velocity of the block in m//s is V=N/10. Then N is :
 .
.
View Solution
