A wire of 9.8×10−3kgm passes over a frictionless light pulley fixed on the top of a frictionless inclined plane which makes an angle of 30∘ with the horizontal. Masses m and M are tied at the two ends of wire such that m rests on the plane and M hangs freely vertically downwards. the entire system is in equilibrium and a transverse wave propagates along the wire with a velocities of 100m/s.
m=20kg
M=5kg
mM=12
mM=2
The correct Answer is:a., d.
To solve the problem step by step, we will analyze the forces acting on the masses and use the information given about the wave velocity and linear mass density.
Step 1: Understanding the Setup
We have a wire with a linear mass density of μ=9.8×10−3kg/m passing over a frictionless pulley. One mass m is on an inclined plane at an angle of 30∘, and the other mass M is hanging vertically. The system is in equilibrium, and a transverse wave propagates along the wire with a velocity of v=100m/s.
Step 2: Free Body Diagram (FBD)
For mass m on the incline:
- The gravitational force acting on it can be resolved into two components:
- Parallel to the incline: mgsin(30∘)
- Perpendicular to the incline: mgcos(30∘)
For mass M hanging vertically:
- The gravitational force acting on it is simply Mg.
Step 3: Writing the Equations of Equilibrium
For mass m on the incline, the tension T in the wire must balance the component of the gravitational force acting down the incline:
T=mgsin(30∘)
For mass M hanging vertically, the tension T must balance the weight of M:
T=Mg
Step 4: Relating the Two Equations
Since both expressions represent the same tension T, we can set them equal to each other:
mgsin(30∘)=Mg
Step 5: Simplifying the Equation
We can cancel g from both sides (as long as g≠0):
msin(30∘)=M
Using sin(30∘)=12:
m⋅12=M
m=2M
Step 6: Finding the Tension in the Wire
The velocity of the wave in the wire is given by:
v=√Tμ
We can rearrange this to find the tension T:
T=v2⋅μ
Substituting the known values:
T=(100)2⋅(9.8×10−3)
T=10000⋅9.8×10−3
T=98N
Step 7: Finding the Mass M
From the equation T=Mg:
98=M⋅9.8
M=989.8=10kg
Step 8: Finding the Mass m
Using the relationship m=2M:
m=2⋅10=20kg
Final Results
- The mass M=10kg
- The mass m=20kg
Conclusion
The values of m and M are 20kg and 10kg respectively.
We have a wire with a linear mass density of μ=9.8×10−3kg/m passing over a frictionless pulley. One mass m is on an inclined plane at an angle of 30∘, and the other mass M is hanging vertically. The system is in equilibrium, and a transverse wave propagates along the wire with a velocity of v=100m/s.
For mass m on the incline:
- The gravitational force acting on it can be resolved into two components:
- Parallel to the incline: mgsin(30∘)
- Perpendicular to the incline: mgcos(30∘)
- The gravitational force acting on it is simply Mg.
For mass m on the incline, the tension T in the wire must balance the component of the gravitational force acting down the incline:
T=mgsin(30∘)
T=Mg
Since both expressions represent the same tension T, we can set them equal to each other:
mgsin(30∘)=Mg
We can cancel g from both sides (as long as g≠0):
msin(30∘)=M
m⋅12=M
m=2M
The velocity of the wave in the wire is given by:
v=√Tμ
We can rearrange this to find the tension T:
T=v2⋅μ
Substituting the known values:
T=(100)2⋅(9.8×10−3)
T=10000⋅9.8×10−3
T=98N
From the equation T=Mg:
98=M⋅9.8
M=989.8=10kg
Using the relationship m=2M:
m=2⋅10=20kg
- The mass M=10kg
- The mass m=20kg
The values of m and M are 20kg and 10kg respectively.
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning
6 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Comprehension
45 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer
1 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A wire of 9.8×10−3kg per meter mass passes over a fricationless pulley fixed on the top of an inclined frictionless plane which makes an angle of 30∘ with the horizontal. Masses M1 & M2 are tied at the two ends of the wire. The mass M1 rests on the plane and the mass M2 hangs freely vertically downwards. the whole system is in equilibrium. Now a transverse wave propagates along the wire with a velocity of 100m/sec. Find the ratio of masses M 1 to M 2 .
View SolutionA person pushes a block of mass 4 kg up a frictionless inclined plane 10 m long and that makes an angle of 30∘ with the horizontal . Then the work done is
View Solution
Knowledge Check
Two blocks each of mass M are resting on a frictionless inclined plane as shown in fig then:
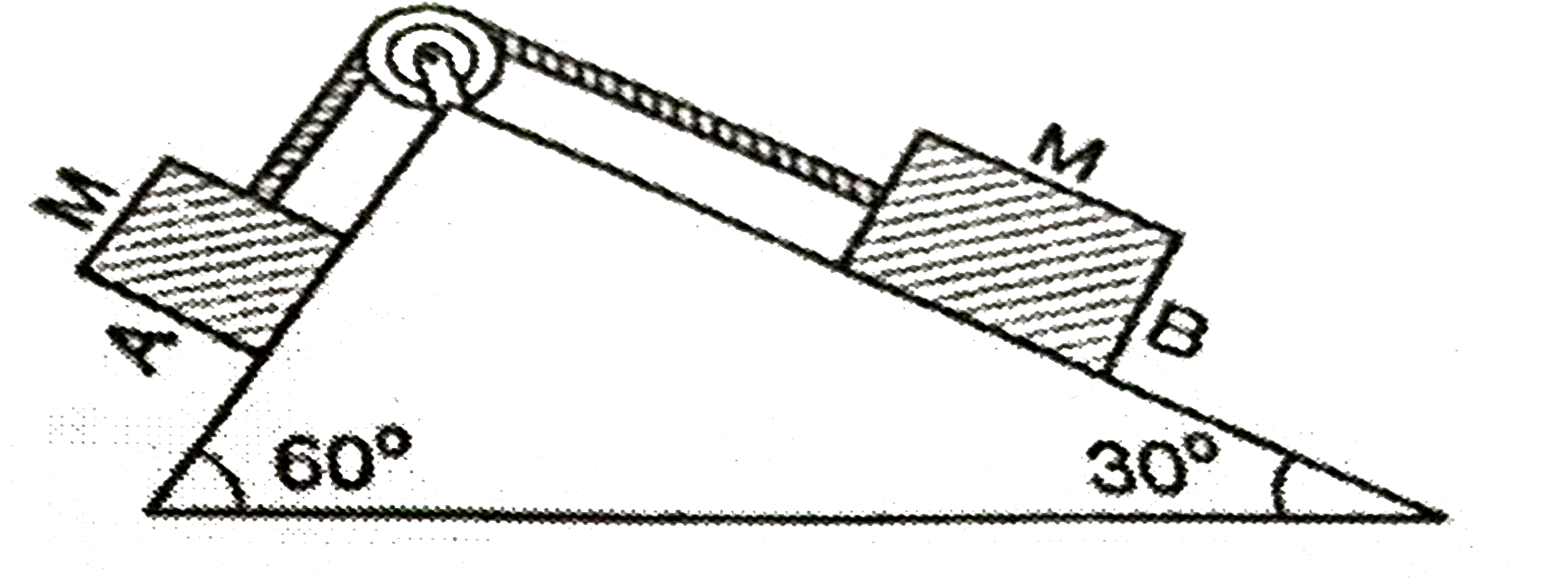 AThe block A moves down the planeBThe block B moves down the plane.CBoth the blocks remain at restDBoth the blocks move down the plane.
AThe block A moves down the planeBThe block B moves down the plane.CBoth the blocks remain at restDBoth the blocks move down the plane.A uniform wire of length 20 m and weighing 5 kg hangs vertically. If g=10ms−2, then the speed of transverse waves in the middle of the wire is
A10ms−1B10√2ms−1C4ms−1D2ms−1A block mass 3 kg slides down a frictionless inclined plane of length 6 m and height 4 m. if the block is released from rest at the top of the incline, what is its speed at the bottom?
A5m/sB6m/sC8m/sD9m/s
The tops of two poles of height 40 m and 25 m are connected by a wire. If the wire makes an angle 30∘ with the horizontal, then the length of the wire is
View SolutionAn ideal massless spring S can compressed 1.0 m in equilibrium by a force of 1000N. This same spring is placed at the bottom of a friction less inclined plane which makes an angle θ=30∘ with the horizontal. A 10kg mass m is released from the rest at top of the inclined plane and is brought to rest momentarily after compressing the spring by 2.0m. the distance through which the mass moved before coming to rest is.
View SolutionTwo bodies of masses 4 kg and 6 kg are tied to the ends of a massless string. The string passes over a frictionless pulley. The acceleration of the system is :
View SolutionThe tops of two poles of height 20 m and 14 m are connected by a wire. If the wire makes an angle of 30∘ with horizontal, then the length of the wire is
(a) 12 m
(b) 10 m
(c) 8 m
(d) 6 m
View SolutionThe work done in pushing a block of mass 10 kg from bottom to the top of a frictionless inclined plane 5 m long and 3 m high is-
(g=9.8m/sec2)
View Solution
