A particle is subjected to two mutually perpendicular simple harmonic such that its x and y coordinates are given by The path of the particle will be
an ellipse
a straight line
a parabola
circle
The correct Answer is:A
Hence
ltbgt
or
or
or
or
This is an equation of ellipse.
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct
35 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Assertion Reasoning
6 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Subjective
21 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
A particle is subjected to two mutually perpendicular simple harmonic motions such that its X and y coordinates are given by X=2sinωt , y=2sin(ω+π4)
The path of the particle will be:
View SolutionA particle is subjected to two mutually perpendicualr simple harmonic motions such that its x and y-coordinates are given by
x=sinωt, y=2cosωt
The path of the particle will be :
View Solution
Knowledge Check
A particle is acted simultaneously by mutually perpendicular simple harmonic motions x=a cosωt and y=asinωt. The trajectory of motion of the particle will be
Aan ellipseBa parabolaCa circleDa straight line.
A xandy co-ordinates of a particle are x=Asin(ωt)andy=Asin(ωt+π/2). Then, the motion of the particle is

View SolutionTwo simple harmonic motions given by, x=asin(ωt+δ) and y=asin(ωt+δ+π2) act on a particle will be
View SolutionIn y= A sin ωt + A sin (ωt+(2π)/3) match the following table.
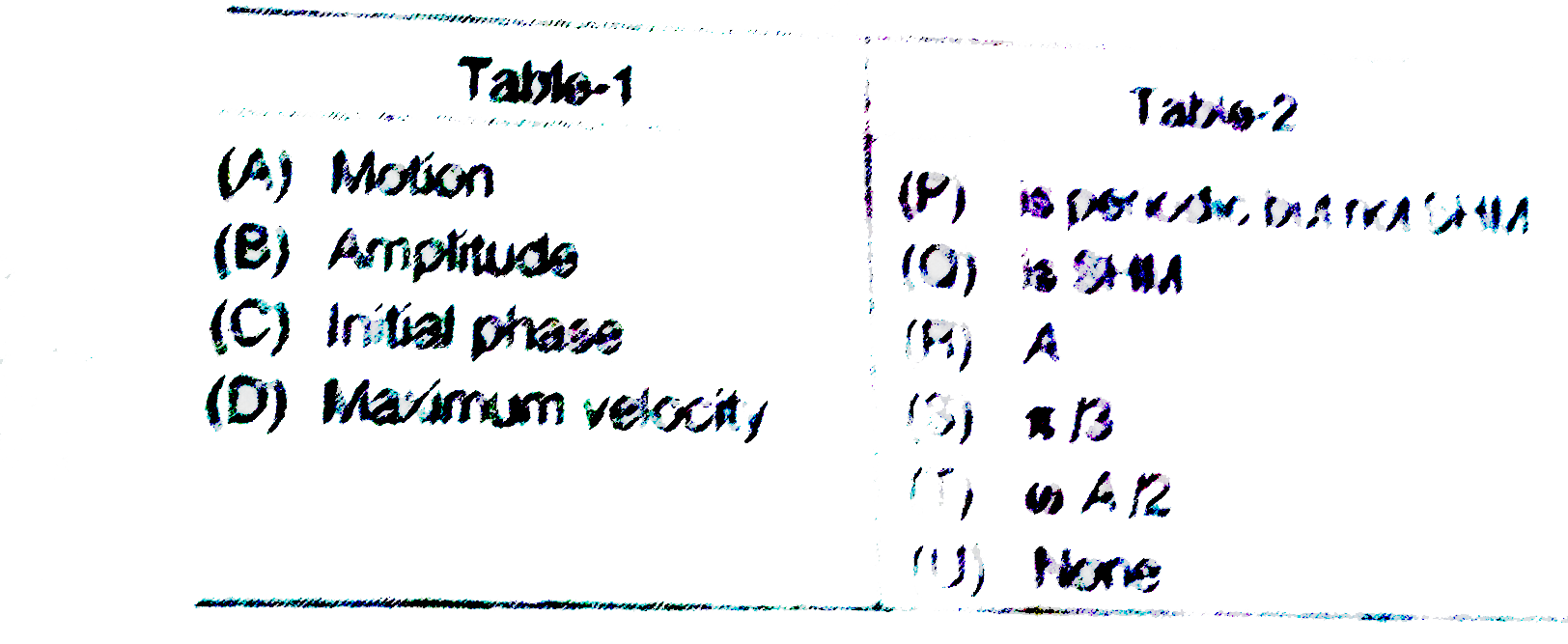
View SolutionA particle is acted simultaneously by mutually perpendicular simple harmonic motions x=acosωtandy=asinωt. The trajectory of motion of the particle will be
View SolutionA particle is subjected to two simple harmonic motions along x and y directions according to x=3sin100πt, y=4sin100πt.
View SolutionThe position vector of a particle is r=asinωtˆi+acosωtˆj
The velocity of the particle is
View SolutionA particule moves in x - y plane acording to rule x = a sin ωt and y = a cos ωt. The particle follows
View Solution
