The frequency of sound produced by a bell is 500 Hz the velocity of the source relative to still air is 60 m/s. An observer moves at 30 m/s along the same line as the source. Calculate the frequency of sound wave measured by the observer. Consider all possible cases (speed of sound .)
To solve the problem, we need to calculate the apparent frequency of sound measured by an observer in different scenarios involving the source and the observer's motion. We will use the Doppler effect formulas for sound waves.
Given Data:
- Frequency of the source (f) = 500 Hz
- Velocity of sound in air (v) = 340 m/s
- Velocity of the source (Vs) = 60 m/s
- Velocity of the observer (Vo) = 30 m/s
Case 1: Source and Observer Moving in the Same Direction
In this case, the formula for the apparent frequency (f') is given by:
f′=f⋅v−Vov−Vs
Substituting the values:
f′=500⋅340−30340−60
f′=500⋅310280
f′=500⋅1.1071≈553.57 Hz
Case 2: Source Moving Towards Observer and Observer Moving Towards Source
In this case, the formula for the apparent frequency (f') is:
f′=f⋅v+Vov−Vs
Substituting the values:
f′=500⋅340+30340−60
f′=500⋅370280
f′=500⋅1.3214≈660.71 Hz
Case 3: Source and Observer Moving Away from Each Other
In this case, the formula for the apparent frequency (f') is:
f′=f⋅v−Vov+Vs
Substituting the values:
f′=500⋅340−30340+60
f′=500⋅310400
f′=500⋅0.775≈387.5 Hz
Case 4: Source Moving Away from Observer and Observer Approaching Source
In this case, the formula for the apparent frequency (f') is:
f′=f⋅v+Vov+Vs
Substituting the values:
f′=500⋅340+30340+60
f′=500⋅370400
f′=500⋅0.925≈462.5 Hz
Summary of Results:
1. Case 1: 553.57 Hz (Same direction)
2. Case 2: 660.71 Hz (Towards each other)
3. Case 3: 387.5 Hz (Away from each other)
4. Case 4: 462.5 Hz (Source moving away, observer approaching)
- Frequency of the source (f) = 500 Hz
- Velocity of sound in air (v) = 340 m/s
- Velocity of the source (Vs) = 60 m/s
- Velocity of the observer (Vo) = 30 m/s
In this case, the formula for the apparent frequency (f') is given by:
f′=f⋅v−Vov−Vs
Substituting the values:
f′=500⋅340−30340−60
f′=500⋅310280
f′=500⋅1.1071≈553.57 Hz
In this case, the formula for the apparent frequency (f') is:
f′=f⋅v+Vov−Vs
Substituting the values:
f′=500⋅340+30340−60
f′=500⋅370280
f′=500⋅1.3214≈660.71 Hz
In this case, the formula for the apparent frequency (f') is:
f′=f⋅v−Vov+Vs
Substituting the values:
f′=500⋅340−30340+60
f′=500⋅310400
f′=500⋅0.775≈387.5 Hz
In this case, the formula for the apparent frequency (f') is:
f′=f⋅v+Vov+Vs
Substituting the values:
f′=500⋅340+30340+60
f′=500⋅370400
f′=500⋅0.925≈462.5 Hz
1. Case 1: 553.57 Hz (Same direction)
2. Case 2: 660.71 Hz (Towards each other)
3. Case 3: 387.5 Hz (Away from each other)
4. Case 4: 462.5 Hz (Source moving away, observer approaching)
Topper's Solved these Questions
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Exercise 6.1
56 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Exercise 6.2
72 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
Similar Questions
a bus is moving towards and stationary observer with speed 20 m/s blows a horn of frequency 200 Hz. the frequency of sound heard by the observer is approximately ( take speed of sound 320 m/s)
View SolutionConsider the vehicle emitting sound wave of frequency 700 Hz moving towards an observer at a speed 22ms−1 . Assuming the observer to be at rest, and speed of sound to be 330ms−1 , the frequency of sound as measured by the observer is
View SolutionA source of sound of frequency 500 Hz is moving towards an observer with velocity 30 m/s . The speed of sound is 330 m/s . the frequency heard by the observer will be
View Solution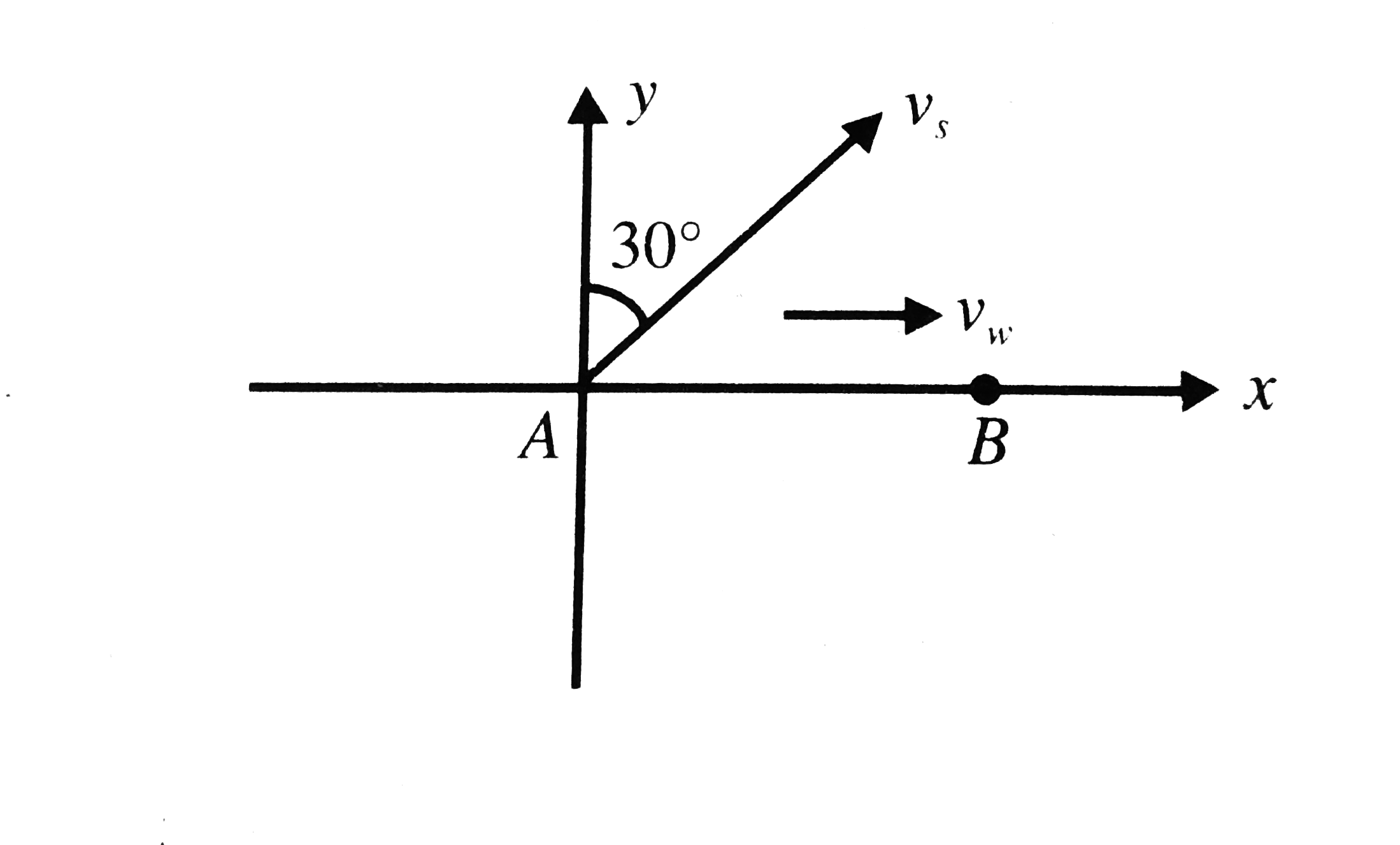
In the figure shown, a source of sound of frequency 510 Hz moves with constant velocity vS=20ms in the direction shown. The wind is blowing at a constant velocity vW=20ms towards an observer who is at rest at point B. corresponding to the sound emitted by the source at initial position A, the frequency detected by the observer is equal to (speed of sound relative to air is 330ms)
View Solution
In the figure shown, a source of sound of frequency 510 Hz moves with constant velocity vS=20ms in the direction shown. The wind is blowing at a constant velocity vW=20ms towards an observer who is at rest at point B. corresponding to the sound emitted by the source at initial position A, the frequency detected by the observer is equal to (speed of sound relative to air is 330ms)
View SolutionAn engine blowing a whistle of frequency 133 Hz moves with a velocity of 60ms towards a hill from which an echo is heard. Calculate the frequency of the echo heard by the driver. (velocity of sound in air in 340ms.)
View SolutionAn engine blowing a whistle of frequency 133 Hz moves with a velocity of 60ms towards a wall from which an echo is heard. Calculate the frequency of the echo heard by the driver. (velocity of sound in air in 340ms.)
View SolutionAn observer moves on the same line on which two sources of sound of frequency 660Hz are present. The observer observes beat frequency of 10Hz. If speed of sound is 300m/s then speed of the observer is:
View SolutionA stationary source of sound is emitting sound of frequency 500Hz. Two observers A and B lying on the same line as the source, observe frequencies 480Hz and 530Hz respectively. The velocity of A and B respectively are (in m/s), speed of sound =300m/s.
View SolutionAt t=0 , observer and source are at same place. Now the source is projected with velocity 60√2m/s at 45∘ . Natural frequency of source is 1000 Hz.find the frequency heard by the observer at t=2s. Take speed of sound = 340 m/s
View Solution
