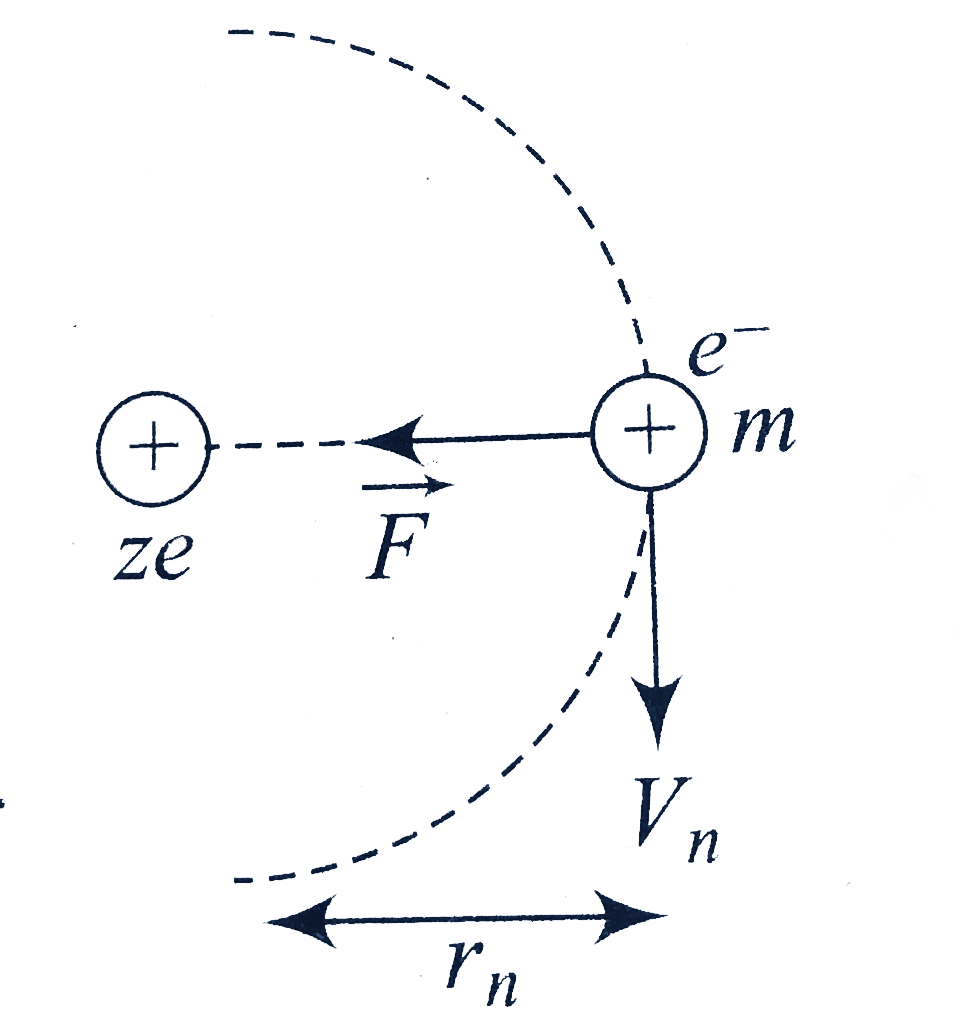
Hydrogen is the simplest atom of nature. There is one proton in its nucleus and an electron moves around the nucleus in a circular orbit. According to Niels Bohr's, this electron moves in a stationary orbit, if emits no electromagnetic radiation. The angular momentum of the electron is quantized , i.e., mvr=(nh/2π), where m= mass of the electron , v=velocity of the electron in the orbit , r=radius of the orbit , and n=1,2,3.... When transition takes place from Kth orbit to Jth orbit, energy photon is emitted. If the wavelength of the emitted photon is λ.
![Hydrogen is the simplest atom of nature. There is one proton in its nucleus and an electron moves around the nucleus in a circular orbit. According to Niels Bohr's, this electron moves in a stationary orbit, if emits no electromagnetic radiation. The angular momentum of the electron is quantized , i.e., mvr = (nh//2 pi), where m = mass of the electron , v =velocity of the electron in the orbit , r =radius of the orbit , and n = 1, 2, 3.... When transition takes place from Kth orbit to Jth orbit, energy photon is emitted. If the wavelength of the emitted photon is lambda. we find that (1)/(lambda) = R [(1)/(J^(2)) - (1)/(K^(2))], where R is Rydberg's constant. On a different planed, the hydrogen atom's structure was somewhat different from ours. The angular momentum of electron was P = 2n (h//2 pi). i.e., an even multipal of (h//2 pi). Answer the following questions regarding the other planet based on above passage: The minimum permissible radius of the orbit will be](https://d10lpgp6xz60nq.cloudfront.net/physics_images/BMS_V04_C04_E01_244_Q01.png)
we find that 1λ=R[1J2−1K2], where R is
Rydberg's constant.
On a different planed, the hydrogen atom's structure was somewhat different from ours. The angular momentum of electron was P=2n(h/2π). i.e., an even multipal of (h/2π).
Answer the following questions regarding the other planet based on above passage:
The minimum permissible radius of the orbit will be
The correct Answer is:B
On other planet: mvr=2nh2π⇒v=nhπmr
mv2r=14πε0e2r2⇒mn2h2n2m2r3=14πε0e2r2
Putting n=1,we≥tr=4πε0mπe2
Topper's Solved these Questions
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Linked Comprehension
62 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Integer
4 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Single Correct
187 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct
10 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
Similar Questions
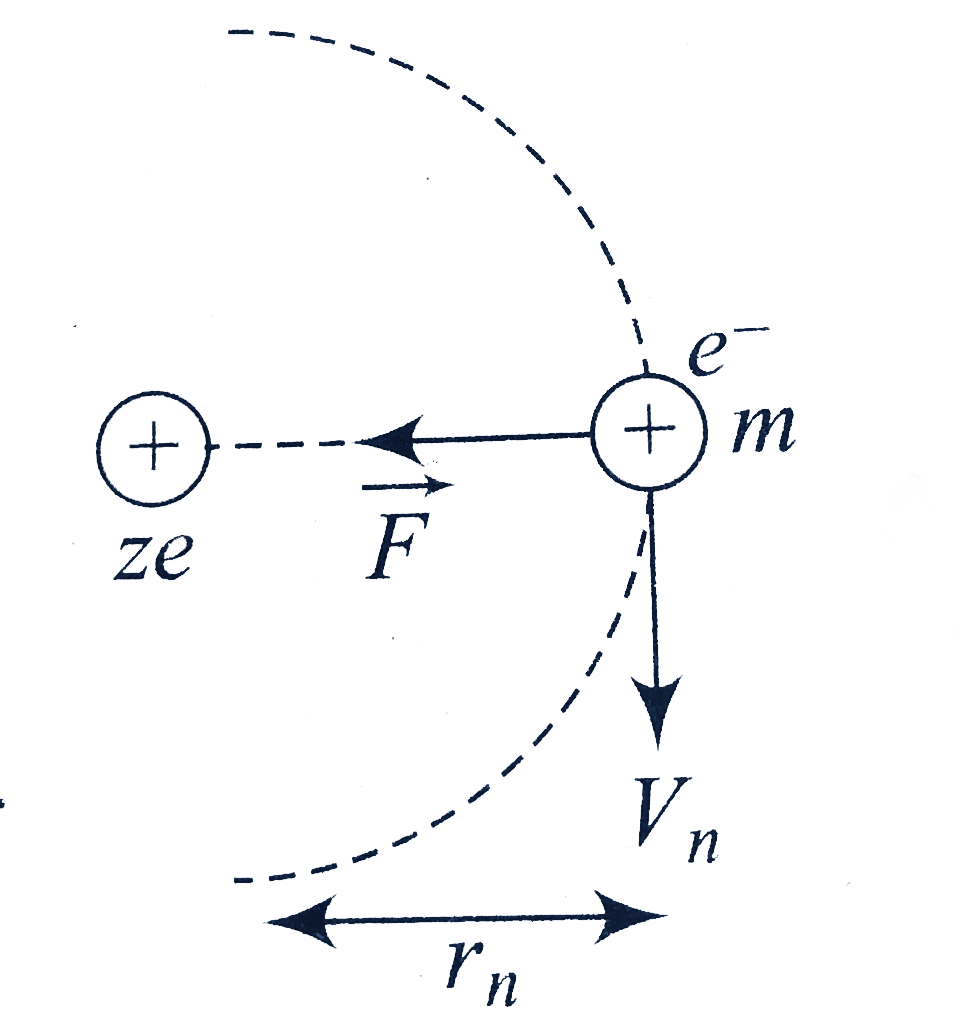
Hydrogen is the simplest atom of nature. There is one proton in its nucleus and an electron moves around the nucleus in a circular orbit. According to Niels Bohr's, this electron moves in a stationary orbit, if emits no electromagnetic radiation. The angular momentum of the electron is quantized , i.e., mvr=(nh/2π), where m= mass of the electron , v=velocity of the electron in the orbit , r=radius of the orbit , and n=1,2,3.... When transition takes place from Kth orbit to Jth orbit, energy photon is emitted. If the wavelength of the emitted photon is λ.
we find that 1λ=R[1J2−1K2], where R is
Rydberg's constant.
On a different planed, the hydrogen atom's structure was somewhat different from ours. The angular momentum of electron was P=2n(h/2π). i.e., an even multipal of (h/2π).
Answer the following questions regarding the other planet based on above passage:
In our world, the velocity of electron is v0 when the hydrogen atom is in the ground state on the other planet should be
View SolutionHydrogen is the simplest atom of nature. There is one proton in its nucleus and an electron moves around the nucleus in a circular orbit. According to Niels Bohr's, this electron moves in a stationary orbit, if emits no electromagnetic radiation. The angular momentum of the electron is quantized , i.e., mvr=(nh/2π), where m= mass of the electron , v=velocity of the electron in the orbit , r=radius of the orbit , and n=1,2,3.... When transition takes place from Kth orbit to Jth orbit, energy photon is emitted. If the wavelength of the emitted photon is λ.
we find that 1λ=R[1J2−1K2], where R is
Rydberg's constant.
On a different planet, the hydrogen atom's structure was somewhat different from ours. The angular momentum of electron was P=2n(h/2π). i.e., an even multiple of (h/2π).
Answer the following questions regarding the other planet based on above passage:
In our world, the ionization potential energy of a hydrogen atom is 13.6eV. On the other planet, this ionization potential energy will be
View Solution
Knowledge Check
The orbital angular momentum of an electron in 2s-orbital is
AZeroBOneCTwoDThreeAccording to Bohr's theory, the electronic energy of H-atom in Bohr's orbit is given by
AEn=−2.18×10−19×Z2n2JBEn=−2.179×10−18×Z2n2JCEn=−21.79×10−18×Z2n2JDEn=−21.8×10−21×Z2n2J
The orbital angular momentum of an electron in 2s-orbital is
View SolutionThe minimum orbital angular momentum of the electron in a hydrogen atom is
View SolutionElectrons revolve around the nucleus in definite orbits called .........
View SolutionThe orbital angular momentum of an electron in 2s orbital is ………
View SolutionAn electron moves round the nucleus of an atom, in a circular path. What is the direction of the angular momentum of the electron ?
View SolutionAn electron moving around the nucleus with an angular momenturm l has a magnetic moment
View Solution
