An isolated coductor, initially free from charge, is charge by repeated conacts with a plate, which afrer each contact has a charge Q due to some mechanism.If q is the charge on the conductor after the first operation, prove that the maximum charge that can be give to the conductor in this way is Qq/Q−q.
To solve the problem step by step, we will analyze the charging process of an isolated conductor through repeated contacts with a charged plate.
Step 1: Initial Conditions
An isolated conductor is initially free from charge, meaning its initial charge Q0=0.
Step 2: First Contact
When the conductor makes contact with the plate that has a charge Q, it acquires a charge q. After the first operation, the charge on the conductor is q.
Step 3: Charge After First Contact
After the first contact, the charge on the plate will reduce to Q−q since it loses charge q to the conductor.
Step 4: Second Contact
When the conductor contacts the plate again, the charge on the conductor will change. The charge on the conductor after the second contact will be q+q2, where q2 is the charge transferred during the second contact.
Step 5: Charge Conservation and Potential Equality
During the contact, the potentials of the conductor and the plate must be equal. The potential of the conductor after the second contact can be expressed as:
Vconductor=q+q2C1
And the potential of the plate is:
Vplate=Q−q+q2C2
Setting these equal gives:
q+q2C1=Q−q+q2C2
Step 6: Relating Capacitances
From the potential equality, we can derive a relationship between the capacitances:
C1⋅(Q−q+q2)=C2⋅(q+q2)
This can be rearranged to give:
C1C2=q+q2Q−q+q2
Step 7: Generalizing for n Contacts
Continuing this process, we can see that after n contacts, the charge on the conductor can be expressed in a series:
qn=Q⋅qn−1Q−qn−1
This forms a geometric series.
Step 8: Maximum Charge Calculation
To find the maximum charge Qmax that can be transferred to the conductor, we sum the series:
Qmax=q+q2+q3+…
This series can be expressed as:
Qmax=q(1+qQ+(qQ)2+…)
This is a geometric series with first term a=q and common ratio r=qQ.
Step 9: Sum of the Geometric Series
The sum of an infinite geometric series is given by:
S=a1−r
Substituting our values:
Qmax=q1−qQ=qQQ−q
Conclusion
Thus, we have proved that the maximum charge that can be given to the conductor through repeated contacts with the plate is:
Qmax=QqQ−q
An isolated conductor is initially free from charge, meaning its initial charge Q0=0.
When the conductor makes contact with the plate that has a charge Q, it acquires a charge q. After the first operation, the charge on the conductor is q.
After the first contact, the charge on the plate will reduce to Q−q since it loses charge q to the conductor.
When the conductor contacts the plate again, the charge on the conductor will change. The charge on the conductor after the second contact will be q+q2, where q2 is the charge transferred during the second contact.
During the contact, the potentials of the conductor and the plate must be equal. The potential of the conductor after the second contact can be expressed as:
Vconductor=q+q2C1
And the potential of the plate is:
Vplate=Q−q+q2C2
Setting these equal gives:
q+q2C1=Q−q+q2C2
From the potential equality, we can derive a relationship between the capacitances:
C1⋅(Q−q+q2)=C2⋅(q+q2)
This can be rearranged to give:
C1C2=q+q2Q−q+q2
Continuing this process, we can see that after n contacts, the charge on the conductor can be expressed in a series:
qn=Q⋅qn−1Q−qn−1
This forms a geometric series.
To find the maximum charge Qmax that can be transferred to the conductor, we sum the series:
Qmax=q+q2+q3+…
This series can be expressed as:
Qmax=q(1+qQ+(qQ)2+…)
This is a geometric series with first term a=q and common ratio r=qQ.
The sum of an infinite geometric series is given by:
S=a1−r
Substituting our values:
Qmax=q1−qQ=qQQ−q
Thus, we have proved that the maximum charge that can be given to the conductor through repeated contacts with the plate is:
Qmax=QqQ−q
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Solved Examples
10 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Exercise 4.1
13 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.3
15 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
Similar Questions
An insulated conductor initiallly free from charge is charged by repeated contacts with a plate which after each contact is replenished to a charge Q. If q is the charge on the conductor after first operation prove that the maximum charge which can be given to the conductor in this way is QqQ−q
View SolutionIn a parallel plate capacitor with plate area A and charge Q, the force on one plate because of the charge on the other is equal to
View SolutionThe charge flowing in a conductor varies with times as Q=at−bt2. Then, the current
View SolutionThe charge flowing in a conductor varies with times as Q=at−bt2. Then, the current
View SolutionThe charge q flowing in a conductor of uniform cross section, varies with time as q=αt−bt2. Then, the current
View SolutionSuppose a charge +Q1 is given to the positive plates and a charge −Q2 to the negative plate of a capacitor. What is the "charge on the capacitor"?
View SolutionA point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
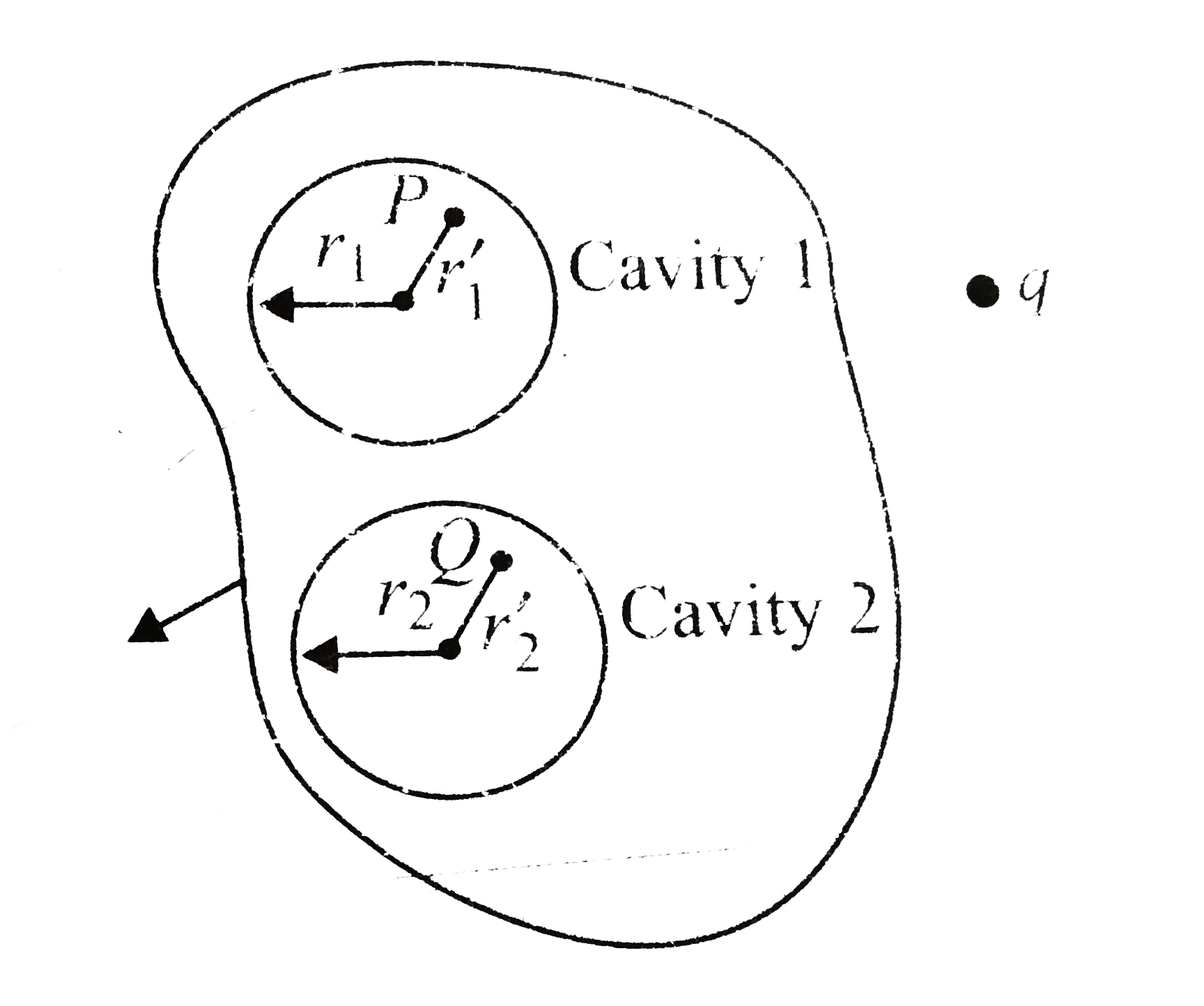
The charge on outer surface of the conductor would be
View SolutionWork done in moving a charge q coulomb on the surface of given charged conductor of potential V is
View SolutionInduced charge q can never be greater than inducing charge Q. Why ?
View SolutionA charge Q is to be divided on two objects. What should be the values of the charges on the objects so that the force between the objects can be maximum?
View Solution
