Find the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
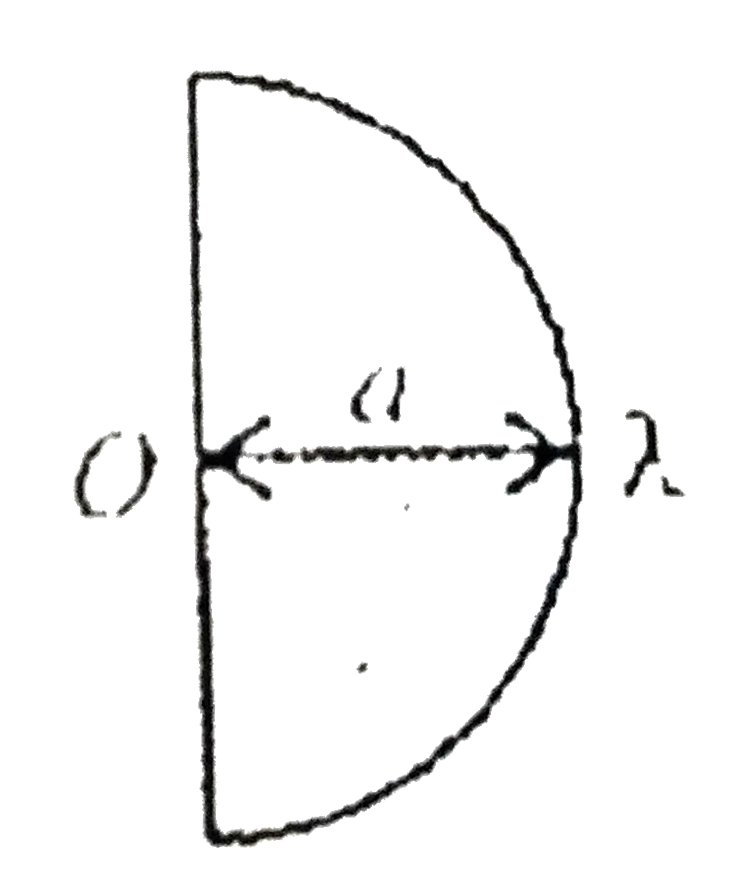
We consider a different elenent dl on the ring that subtends an angle dθ at the center of the ring, i.e. dl=Rdθ. So the charge on this element is dQ=λRdθ. This element creates a field dE, which makes an angle θ at the center as shown in fig.

For each differential element in the upper half of the ring, there corresponds a symmetrically palced charge element in the lower half. the y-components of the field due to these symmetric elements cancle out, and the x-components remain. So we get
dEx=dEcosθ=dQ4πε0r2cosθ=λ(Rdθ)cosθ4πε0R2
On intergrating the expression for dEx with respect to angle θ in limits θ=−(π)/2→θ=+π/2 we obtain
E=∫+π/2−π/2λR4πε0R2cosθdθ=λ2πε0R
In terms of the total charge, say Q, on the ring λ=Q/πR and we get E=Q/2π2ε0R2
If we consider the wire in the form of an are aas shown in fig. the symmetry consideration is not useful in canceling out x- and y- components of the fields , if θ1 and θ2 are different. We will intergrated dEx as well as dEy in limits θ=−θ1 to θ=−θ2.
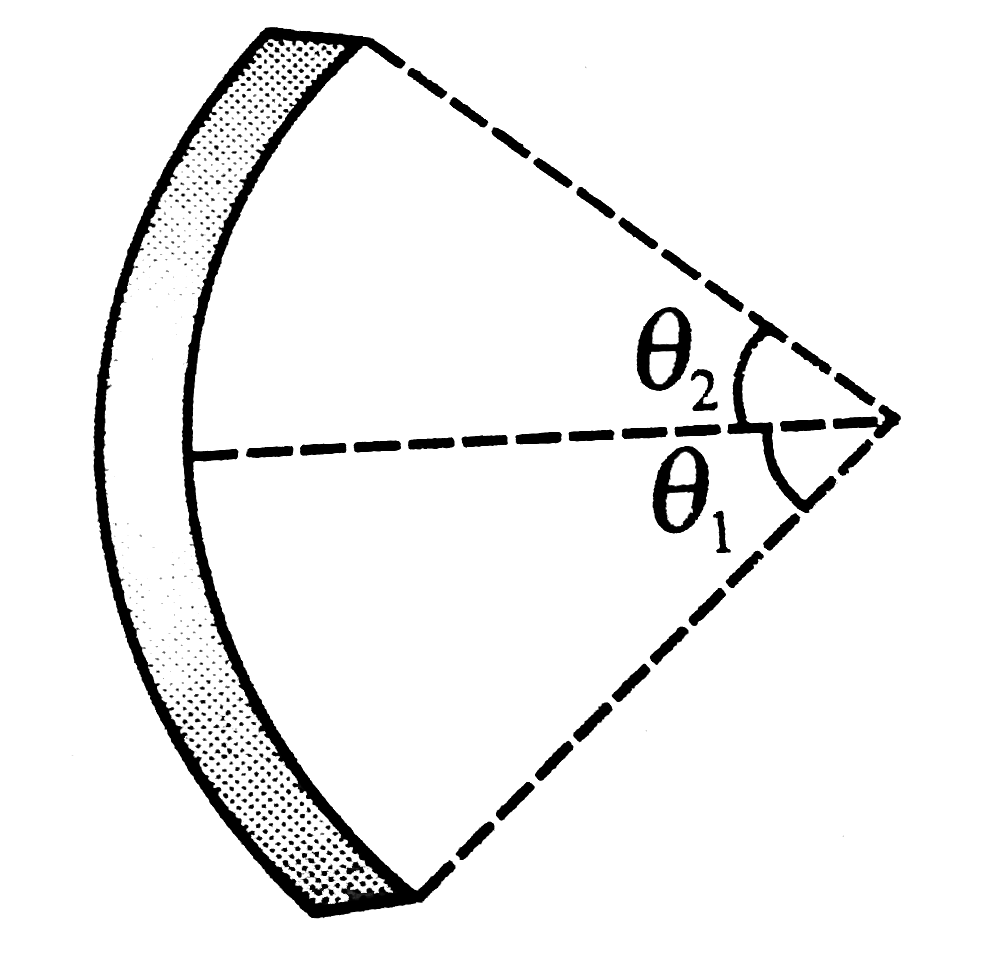
Ex=∫+θ2−θ1λR4πε_(0)R2cosθdθ=λ4πε0R2(sinθ1−sinθ2)
Ey=∫+θ2−θ1λR4πε0R2sinθdθ=λ4πε0R2(cosθ2+cosθ1)
For a sysmmetrical are , θ1=θ2. thus Ey vanishes and
Ex=λsinθ2πε0R.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Solved examples
10 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises
58 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
Similar Questions
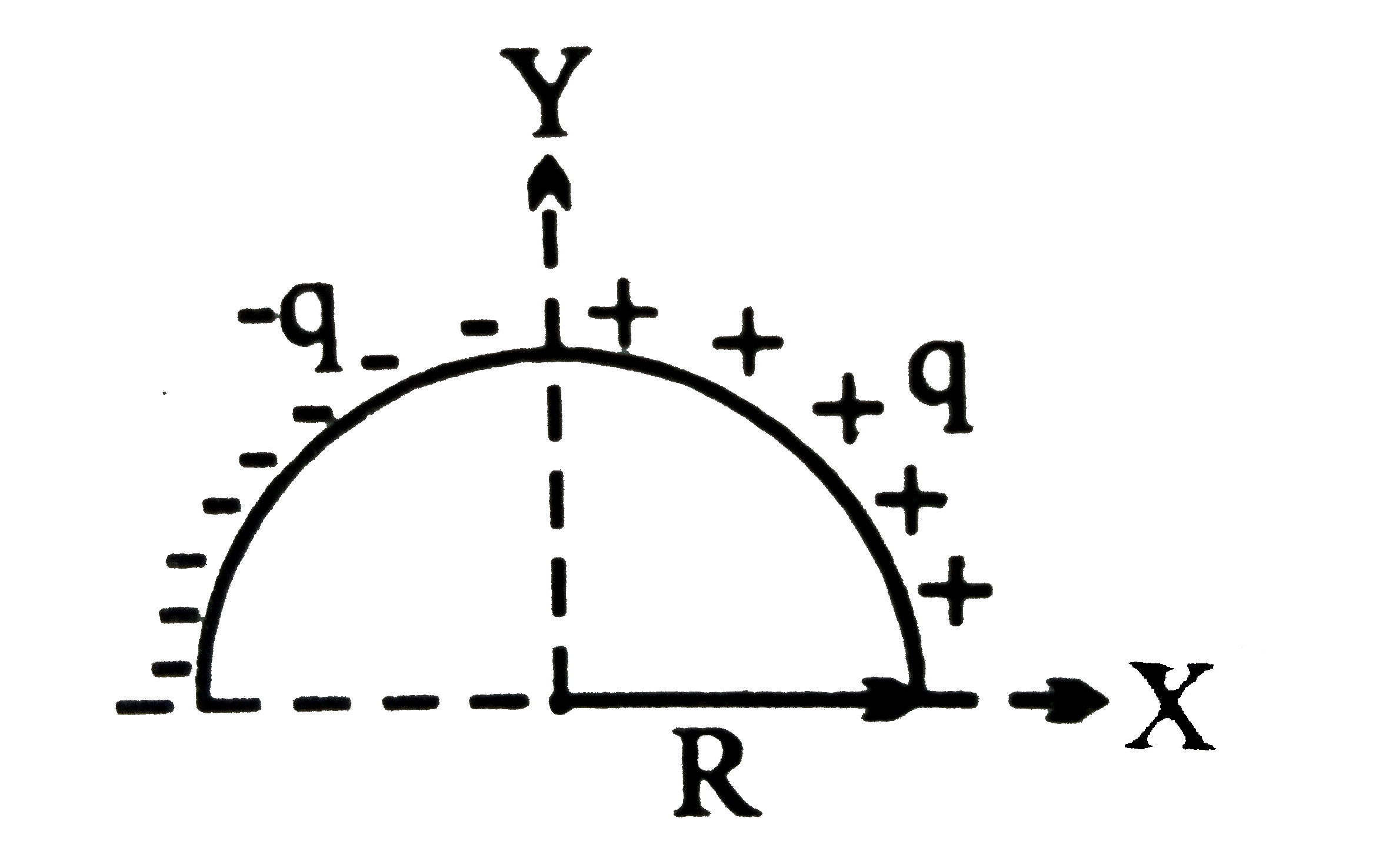
Electric field at centre of a uniforly charged semicirlce of radius a is
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionFind the electric field at centre of semicircular ring shown in figure .
 .
.
View SolutionElectric field at centre O of semicircule of radius 'a' having linear charge density λ given is given by

View SolutionElectric field at the centre of uniformly charge hemispherical shell of surface charge density σ is σnε0 then find the value of n.
View SolutionThe electric field at 2R from the centre of a uniformly charged non - conducting sphere of rarius R is E. The electric field at a distance R2 from the centre will be
View Solution(a) Using Gauss's law, derive an expression for the electric field intensity at any point outside a uniformly charged thin spherical shell of radius R and charge density σC/m2. Draw the field lines when the charge density of the sphere is (i) positive, (ii) negative.
(b) A uniformly charged conducting sphere of 2.5 m in diameter has a surface charge density of 100 μC/m2. Calculate the
(i) charge on the sphere
(ii) total electric flux passing through the sphere.
View SolutionElectric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to
View SolutionFind the electric field due to an infinitely long cylindrical charge distribution of radius R and having linear charge density λ at a distance half of the radius from its axis.
View SolutionA point charge q0 is placed at the centre of uniformly charges ring of total charge Q and radius R. If the point charge is slightly displaced with negligible force along axis of the ring then find out its speed when it reaches a large distance.
View Solution
