A jet of water is projected at an angle θ=45∘ with horizontal from point A which is situated at a distance x=OA=(a)1/2m,(b)2m from a vertical wall. If the speed of projection is v0=√10ms−1, find point P of strinking of the water jet with the vertical wall.
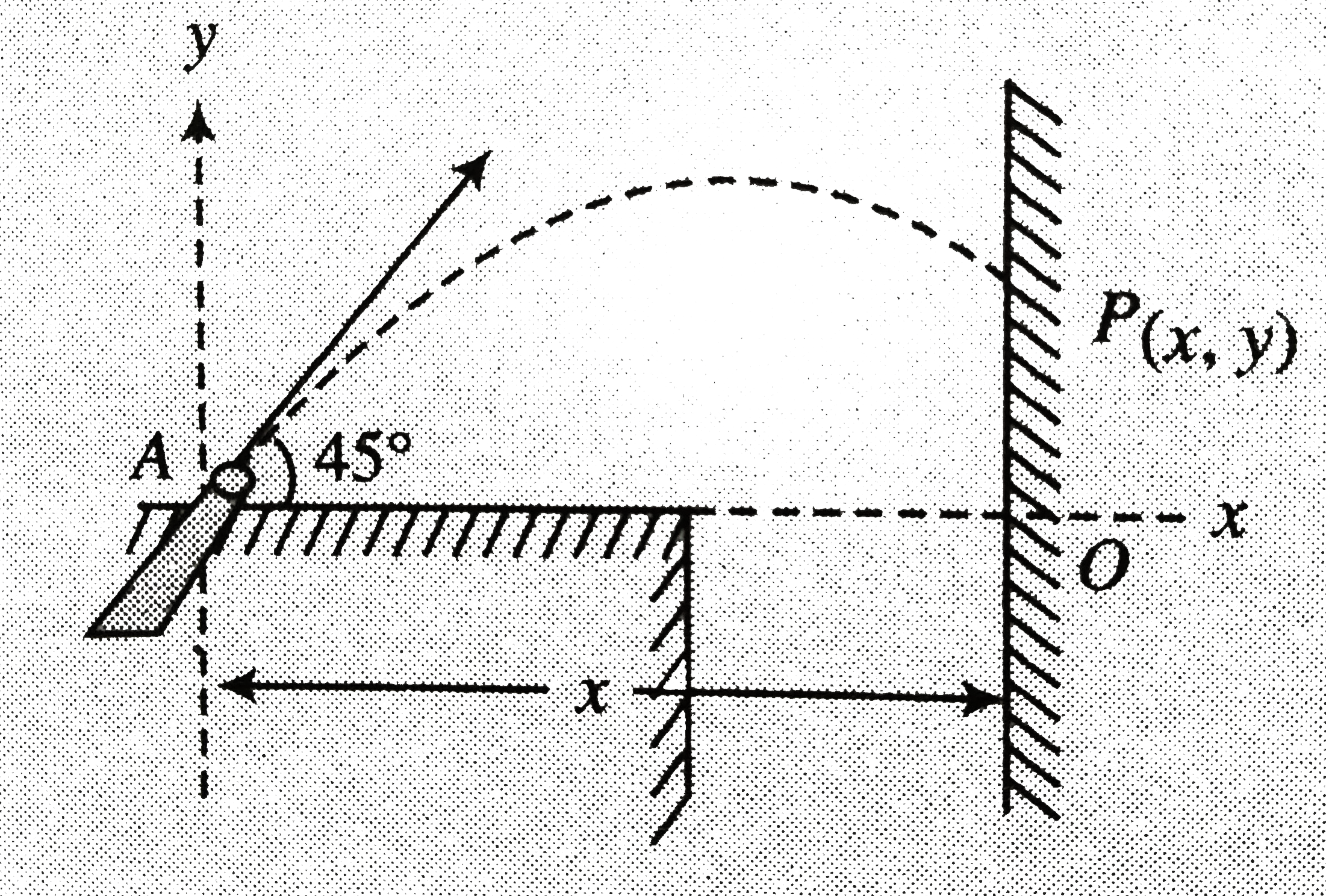 .
.
Point P lies at the trajectory of jet of water. Hence, the coordinate of point P(x,y) should satisfy the trajectory equation.
y=xtanθ(1−xR)…(i)
R=u2sin2θg=(√10)2.sin2×45∘10=1m
(a) If x=1/2m from (i) y=12tan45∘(1−1/21)=12×12=14m
Hence, the coordinate of P=(12m,14m))
 .
.
(b) If x=2m, from (i),
y=2.tan45∘(1−21)=−1m
Hence,water jet will strike below the horizontal dotted line (x - axis) at coordinate (2m,−1m).
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
A ball is thrown from the ground to clear a wall 3 m high at a distance of 6 m and falls 18 m away from the wall. Find the angle of projection of ball.
View SolutionA ball is thrown from the ground to clear a wall 3 m high at a distance of 6 m and falls 18 m away from the wall. Find the angle of projection of ball.
View SolutionA ball is projected at t=0 with velocity v0 at angle θ with horizontal. It strikes a smooth wall at a distance from it and then falls at a distance d from the wall. Coefficient of restitution is 'e' then :
View SolutionA projectile, thrown with velocity v0 at an angle α to the horizontal, has a range R. it will strike a vertical wall at a distance R/2 from the point of projection with a speed of
View SolutionOne second after the projection, a stone moves at an angle of 45∘ with the horizontal. Two seconds from the start,
it is travelling horizontally. Find the angle of projection with the horizontal. (g=10ms−2).
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA particle of mass m=1kg is projected with speed u=20√2m/s at angle θ=45∘ with horizontal find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View SolutionA particle of mass m is projected with speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle of mass m is projected with speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View Solution
