Question

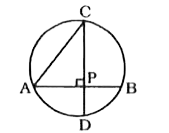
A circle with radius 12 cm has two perpendicular chords AB and CD intersecting at a point R, other than the centre O, where RD=6cm and RB=18cm. Find the length of AR.

Options

A. A)`6root()(3/5)`
B. B)`4root()(3/5)`
C. C)`root(4)(5)`
D. D)data insufficient

Answer

A

Explanation

To solve the problem step by step, we will use the information provided about the circle and the chords.

Step 1: Understand the given information
We have a circle with a radius of 12 cm. The two perpendicular chords AB and CD intersect at point R. The lengths given are:
- RD = 6 cm
- RB = 18 cm

We need to find the length of AR, which we will denote as \( x \).

Step 2: Determine the lengths of the segments
Since the chords intersect at R, we can express the lengths of the segments:
- Let AR = \( x \)
- Since RB = 18 cm, we have \( RB = 18 \)
- Let CR = \( y \)

Step 3: Use the property of perpendicular chords
In a circle, if two chords intersect, the products of the lengths of the segments of each chord are equal. Therefore, we can write:
\( AR \cdot RB = CR \cdot RD \)
Substituting the known values:
\( x \cdot 18 = y \cdot 6 \)
From this, we can express \( y \) in terms of \( x \):
\( y = \frac{18x}{6} = 3x \)

Step 4: Apply the Pythagorean theorem
Since the chords are perpendicular, we can use the Pythagorean theorem. The relationship between the segments and the radius of the circle can be expressed as:
\( AR^2 + RB^2 + CR^2 + RD^2 = \text{Diameter}^2 \)
The diameter of the circle is \( 2 \times \text{radius} = 24 \) cm, so:
\( 24^2 = 576 \)
Substituting the values we have:
\( x^2 + 18^2 + (3x)^2 + 6^2 = 576 \)
Calculating the squares:
\( x^2 + 324 + 9x^2 + 36 = 576 \)
Combining like terms:
\( 10x^2 + 360 = 576 \)

Step 5: Solve for \( x^2 \)
Subtract 360 from both sides:
\( 10x^2 = 576 - 360 \)
\( 10x^2 = 216 \)
Now, divide by 10:
\( x^2 = \frac{216}{10} = \frac{108}{5} \)

Step 6: Find the value of \( x \)
Taking the square root:
\( x = \sqrt{\frac{108}{5}} = \frac{\sqrt{108}}{\sqrt{5}} = \frac{6\sqrt{3}}{\sqrt{5}} \)

Step 7: Final answer
Thus, the length of AR is:
\( AR = 6 \frac{\sqrt{3}}{\sqrt{5}} \text{ cm} \)