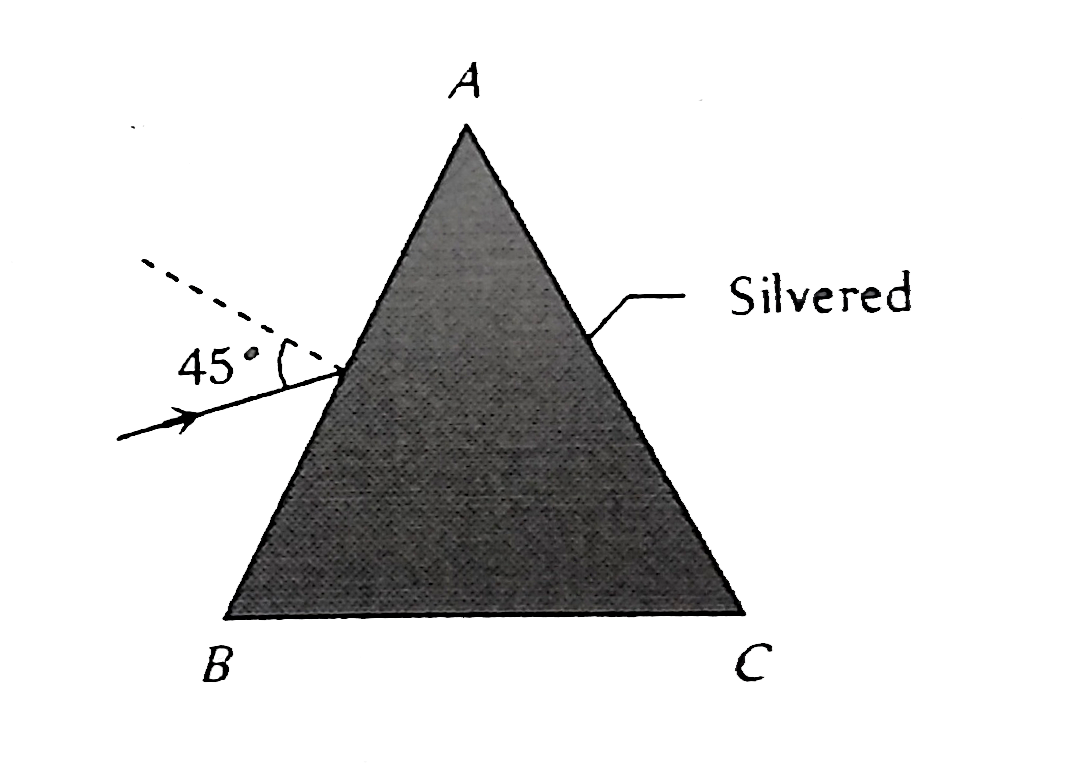
One face of a prism with a refrective angle of 30∘ is coated with silver. A ray of light incident on another face at an angle of 45∘ is refracted and reflected from the silver coated face and retraces its path. What is the refractive index of the prism?
To find the refractive index of the prism, we can follow these steps:
Step 1: Understand the Geometry of the Prism
We have a prism with a refractive angle A=30∘. A ray of light is incident on one face of the prism at an angle i1=45∘.
Step 2: Identify the Angles
When the ray of light enters the prism, it is refracted. Since the ray retraces its path after reflecting off the silvered face, we can conclude that the angle of refraction r2=0∘ (the ray reflects back along the same path).
Step 3: Use the Prism Formula
For a prism, the relationship between the angles is given by:
A=r1+r2
Where:
- A is the angle of the prism,
- r1 is the angle of refraction when the light enters the prism,
- r2 is the angle of refraction when the light exits the prism.
Since r2=0∘, we have:
A=r1+0∘⟹r1=A=30∘
Step 4: Apply Snell's Law
Using Snell's Law at the first interface (air to prism), we have:
μ=sini1sinr1
Where:
- μ is the refractive index of the prism,
- i1=45∘,
- r1=30∘.
Step 5: Calculate the Refractive Index
Substituting the known values into Snell's Law:
μ=sin45∘sin30∘
We know:
- sin45∘=1√2
- sin30∘=12
Thus,
μ=1√212=1√2×2=√2
Final Answer
The refractive index of the prism is μ=√2.
---
We have a prism with a refractive angle A=30∘. A ray of light is incident on one face of the prism at an angle i1=45∘.
When the ray of light enters the prism, it is refracted. Since the ray retraces its path after reflecting off the silvered face, we can conclude that the angle of refraction r2=0∘ (the ray reflects back along the same path).
For a prism, the relationship between the angles is given by:
A=r1+r2
Where:
- A is the angle of the prism,
- r1 is the angle of refraction when the light enters the prism,
- r2 is the angle of refraction when the light exits the prism.
A=r1+0∘⟹r1=A=30∘
Using Snell's Law at the first interface (air to prism), we have:
μ=sini1sinr1
Where:
- μ is the refractive index of the prism,
- i1=45∘,
- r1=30∘.
Substituting the known values into Snell's Law:
μ=sin45∘sin30∘
- sin45∘=1√2
- sin30∘=12
μ=1√212=1√2×2=√2
The refractive index of the prism is μ=√2.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.1
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.2
10 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
A prism ABC of angle 30∘ has its face AC silvered. A ray of light incident at an angle of 45∘ at the face AB retraces its path after refraction at face AB and reflection at face AC . The refractive index of the material of the prism is
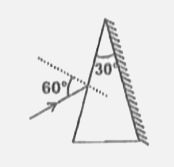
View SolutionOne of the refracting surfaces of a prism of angle of 30∘ is silvered. A ray of light incident at an angle of 60∘ retraces its path. The refractive index of the material of prism is
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View SolutionA ray of light is incident at 65∘ on one face of a prism of angle of 30∘ and the emergent ray makes 35∘ with the incident ray. The refractive index of the prism is:
View SolutionA glass prism has a refractive angle of 90∘ and a refractive index of 1.5. A ray is incident at an angle of 30∘. The ray emerges from an adjacent face at an angle of
View SolutionA ray of light is incident at an angle of 60∘ on the face of a prism having refracting angle 30∘. The ray emerging out of the prism makes an angle 30∘ with the incident ray. Show that the emergent ray is perpendicular to the face through which it emerges and calculate the refractive index of the material of prism.
View SolutionA ray of light is incident at an angle of 60∘ on the face of a prism having refracting angle 30∘. The ray emerging out of the prism makes an angle 30∘ with the incident ray. Show that the emergent ray is perpendicular to the face through which it emerges and calculate the refractive index of the material of prism.
View SolutionA ray of light is incident on the face AB of a glass prism ABC having vertex anlge A equal to 30∘. The face AC is silvered and a ray of light incident on the face AB retraces its path. If the refractive index of the material of prism is √3 find the angle of incidence on the face AB.
View SolutionAn isosceles prism of angle A=30∘ has one of its surfaces silvered. Light rays falling at an angle of incidence 60∘ on the other surface retrace their path after reflection from the silvered surface. The refractive index of prism material is
View SolutionAngle of a prism is 30∘ and its refractive index is √2 and one of the surface is silvered. At what angle of incidence, a ray should be incident on one surface so that after reflection from the silvered surface, it retraces its path ?
View Solution
