Assertion : In x=3+4 , amplitude of oscillation is 4 units.
Reason : Mean position is at x=3.
If both Assertion and Reason are correct and Reason is the correct explanation of Assertion.
If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.
If Assertion is true but Reason is false.
If Assertion is false but Reason is true.
The correct Answer is:B
To solve the given question, we need to analyze both the assertion and the reason provided.
Step 1: Understanding the Assertion
The assertion states that in the equation x=3+4cos(ωt), the amplitude of oscillation is 4 units.
Explanation:
In a simple harmonic motion (SHM), the general form of the equation is:
x=Acos(ωt+ϕ)
where A is the amplitude. In the given equation, x=3+4cos(ωt), we can see that the term 4 is the coefficient of the cosine function, which represents the amplitude. Therefore, the amplitude is indeed 4 units.
Step 2: Understanding the Reason
The reason states that the mean position is at x=3.
Explanation:
In the equation x=3+4cos(ωt), the mean position (or equilibrium position) is the value of x when the cosine function is zero (i.e., when cos(ωt)=0). This occurs when:
x=3+4⋅0=3
Thus, the mean position is indeed at x=3.
Step 3: Conclusion
Both the assertion and the reason are correct:
- The amplitude of oscillation is 4 units (Assertion is true).
- The mean position is at x=3 (Reason is true).
However, the reason does not explain the assertion directly; it simply states a fact about the mean position without linking it to the amplitude.
Final Answer
Both the assertion and reason are correct, but the reason is not the correct explanation for the assertion. Therefore, the correct option is that both assertion and reason are correct, but the reason does not explain the assertion.
---
The assertion states that in the equation x=3+4cos(ωt), the amplitude of oscillation is 4 units.
In a simple harmonic motion (SHM), the general form of the equation is:
x=Acos(ωt+ϕ)
where A is the amplitude. In the given equation, x=3+4cos(ωt), we can see that the term 4 is the coefficient of the cosine function, which represents the amplitude. Therefore, the amplitude is indeed 4 units.
The reason states that the mean position is at x=3.
In the equation x=3+4cos(ωt), the mean position (or equilibrium position) is the value of x when the cosine function is zero (i.e., when cos(ωt)=0). This occurs when:
x=3+4⋅0=3
Thus, the mean position is indeed at x=3.
Both the assertion and the reason are correct:
- The amplitude of oscillation is 4 units (Assertion is true).
- The mean position is at x=3 (Reason is true).
Both the assertion and reason are correct, but the reason is not the correct explanation for the assertion. Therefore, the correct option is that both assertion and reason are correct, but the reason does not explain the assertion.
Similar Questions
Knowledge Check
Assertion : The amplitude of oscillation can never be infinite. Reason : The energy of oscillator is continuously dissipated.
AIf both assertion and reson are true and reason is the correct explanation of assertionBIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
Assertion : In x=Acosωt, x is the displacement measured from extreme position.
Reason : In the above equation x=A at time t=0.
View SolutionAn object of mass 0.2 kg executes simple harmonic oscillation along the x-axis with a frequency 25π. At the position x = 0.04m, the object has kinetic energy 0.5J and potential energy 0.4J. amplitude of oscillation is (potential energy is zero mean position).
View SolutionA body executing shm completes 120 oscillations per minute. If the amplitude of oscillations is 6 cm, find the velocity and acceleration of the particle when it is at a distance of 4 cm from the mean position?
View SolutionThe position of S.H.M. is given by x=2[√3sin πt +cos πt]. The amplitude of motion is [ x is in metre and symbols have the usual meaning]
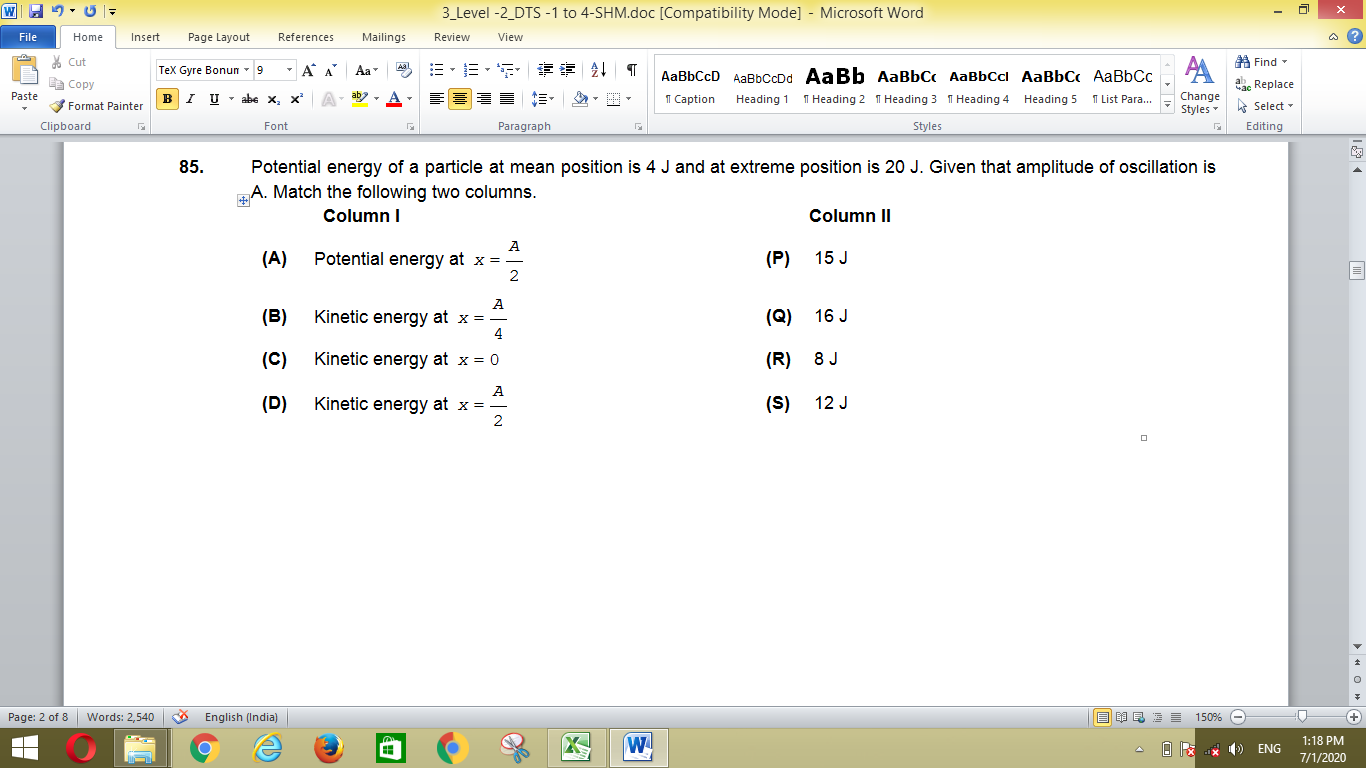
View SolutionPotential energy of a particle at mean position is 4 J and at extreme position is 20 J. Given that amplitude of oscillation is A. Match the following two columns
View SolutionA particle of mass (m) is executing oscillations about the origin on the (x) axis. Its potential energy is V(x)=k|x|3 where (k) is a positive constant. If the amplitude of oscillation is a, then its time period (T) is.
View SolutionA particle of mass (m) is executing oscillations about the origin on the (x) axis. Its potential energy is V(x)=k|x|3 where (k) is a positive constant. If the amplitude of oscillation is a, then its time period (T) is.
View Solution
