When a metal is illuminated with light of frequency f, the maximum kinetic energy of the photoelectrons is 1.2 eV. When the frequency is increased by 50% the maximum kinetic energy increases to 4.2 eV. What is the threshold frequency for this metal?
The correct Answer is:A
To find the threshold frequency for the metal, we can use the photoelectric equation:
KE=hf−ϕ
where:
- KE is the maximum kinetic energy of the photoelectrons,
- h is Planck's constant,
- f is the frequency of the incident light,
- ϕ is the work function of the metal, which is related to the threshold frequency f0 by ϕ=hf0.
Step 1: Write the equations for the two scenarios given in the problem.
1. For the first frequency f:
KE1=hf−ϕ(1)
Given KE1=1.2eV.
2. For the second frequency f′ which is increased by 50%:
f′=1.5f
Thus, the kinetic energy becomes:
KE2=hf′−ϕ(2)
Given KE2=4.2eV.
Step 2: Substitute f′ in equation (2).
Substituting f′ into equation (2):
4.2=h(1.5f)−ϕ
Step 3: Rewrite the equations.
Now we have two equations:
1. From equation (1):
1.2=hf−ϕ(3)
2. From the modified equation (2):
4.2=1.5hf−ϕ(4)
Step 4: Solve for ϕ in terms of f.
From equation (3):
ϕ=hf−1.2(5)
Step 5: Substitute ϕ from (5) into (4).
Substituting (5) into (4):
4.2=1.5hf−(hf−1.2)
Simplifying this:
4.2=1.5hf−hf+1.2
4.2=0.5hf+1.2
Step 6: Solve for hf.
Subtracting 1.2 from both sides:
4.2−1.2=0.5hf
3.0=0.5hf
hf=3.00.5=6.0eV(6)
Step 7: Substitute hf back to find ϕ.
Now substituting hf=6.0eV back into equation (5):
ϕ=6.0−1.2=4.8eV
Step 8: Find the threshold frequency f0.
Using the relation ϕ=hf0:
f0=ϕh
Using h=4.135667696×10−15eV.s:
f0=4.84.135667696×10−15≈1.16×1015Hz
Final Answer:
The threshold frequency f0 for this metal is approximately 1.16×1015Hz.
---
- KE is the maximum kinetic energy of the photoelectrons,
- h is Planck's constant,
- f is the frequency of the incident light,
- ϕ is the work function of the metal, which is related to the threshold frequency f0 by ϕ=hf0.
KE1=hf−ϕ(1)
Given KE1=1.2eV.
f′=1.5f
Thus, the kinetic energy becomes:
KE2=hf′−ϕ(2)
Given KE2=4.2eV.
4.2=h(1.5f)−ϕ
1. From equation (1):
1.2=hf−ϕ(3)
2. From the modified equation (2):
4.2=1.5hf−ϕ(4)
ϕ=hf−1.2(5)
4.2=1.5hf−(hf−1.2)
Simplifying this:
4.2=1.5hf−hf+1.2
4.2=0.5hf+1.2
4.2−1.2=0.5hf
3.0=0.5hf
hf=3.00.5=6.0eV(6)
ϕ=6.0−1.2=4.8eV
f0=ϕh
f0=4.84.135667696×10−15≈1.16×1015Hz
The threshold frequency f0 for this metal is approximately 1.16×1015Hz.
Topper's Solved these Questions
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level -1 Assertion And Reason
10 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 1 Objective
37 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Exercise 33.3
6 Videos
- MODERN PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICSEXERCISE - Integer Type Questions
17 Videos
- MODERN PHYSICS - 2BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 Subjective
10 Videos
Similar Questions
The maximum kinetic energy of a photoelectron is 3 eV. What is its stopping potential ?
View SolutionIf linear momentum if increased by 50% then kinetic energy will be increased by
View SolutionMaximum kinetic energy (Ek) of a photoelectron varies with the frequency (v) of the incident radiation as
View SolutionThe maximum kinetic energy of the photoelectrons is found to be 6.63×10−19J, when the metal is irradiated with a radiation of frequency 2×1015Hz. The threshold frequency of the metal is about:
View SolutionWhen an electro-magnetic radiation is incident on the surface of metal, maximum kinetic energy of photoelectron depends on-
View SolutionThe surface of a metal illuminated with the light of 400nm. The kinetic energy of the ejected photoelectrons was found to be 1.68eV. The work function of the metal is : (hc=1240eV.nm)
View SolutionLight of wavelength 4000A∘ is incident on a metal surface. The maximum kinetic energy of emitted photoelectron is 2 eV. What is the work function of the metal surface?
View SolutionIf the frequency of light in a photoelectric experiment is double then maximum kinetic energy of photoelectron
View SolutionThe maximum kinetic energy of photoelectrons emitted from a metal surface increses from 0.4 eV to 1.2 eV when the frequency of the incident radiation is increased by 40% . What is the work function (in eV) of the metal surface?
View SolutionIn an experiment of photo-electric effect, the graph of maximum kinetic energy Ek of the emitted photoelectrons versus the frequency v of the incident light is a straight line AB as shown in figure below :
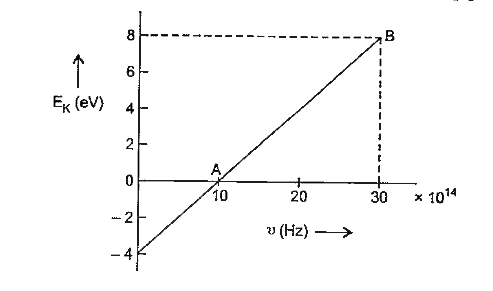
Threshold frequency of the metal.
View Solution
