A wave yi=0.3cos(2.0x−40t) is travelling along a string toward a boundary at x=0. Write expressions for the reflected waves if .
(a) the string has a fixed end at x=0 and
(b) The string has a free end at x=0.
Assume SI units.
The correct Answer is:A, B, C, D
To solve the problem, we need to analyze the behavior of the wave when it encounters two different types of boundaries: a fixed end and a free end. The given incident wave is:
yi=0.3cos(2.0x−40t)
Step 1: Identify the incident wave parameters
The wave function is given in the form of yi=Acos(kx−ωt), where:
- Amplitude A=0.3
- Wave number k=2.0 (which relates to wavelength)
- Angular frequency ω=40 (which relates to frequency)
Step 2: Determine the reflected wave for a fixed end
At a fixed boundary, the wave reflects with a phase change of π (or 180 degrees). This means the reflected wave will have the same amplitude but will change the sign of the cosine function. Therefore, the reflected wave yr can be expressed as:
yr=−Acos(kx+ωt)
Substituting the values of A, k, and ω:
yr=−0.3cos(2.0x+40t)
Step 3: Determine the reflected wave for a free end
At a free boundary, the wave reflects without any phase change. This means the reflected wave will have the same amplitude and will maintain the same cosine function but will change the direction of propagation. Thus, the reflected wave yr is given by:
yr=Acos(kx+ωt)
Substituting the values of A, k, and ω:
yr=0.3cos(2.0x+40t)
Final Expressions
- For the fixed end at x=0:
yr=−0.3cos(2.0x+40t)
- For the free end at x=0:
yr=0.3cos(2.0x+40t)
Summary of Results
1. Fixed End Reflection: yr=−0.3cos(2.0x+40t)
2. Free End Reflection: yr=0.3cos(2.0x+40t)
---
The wave function is given in the form of yi=Acos(kx−ωt), where:
- Amplitude A=0.3
- Wave number k=2.0 (which relates to wavelength)
- Angular frequency ω=40 (which relates to frequency)
At a fixed boundary, the wave reflects with a phase change of π (or 180 degrees). This means the reflected wave will have the same amplitude but will change the sign of the cosine function. Therefore, the reflected wave yr can be expressed as:
At a free boundary, the wave reflects without any phase change. This means the reflected wave will have the same amplitude and will maintain the same cosine function but will change the direction of propagation. Thus, the reflected wave yr is given by:
- For the fixed end at x=0:
yr=−0.3cos(2.0x+40t)
yr=0.3cos(2.0x+40t)
1. Fixed End Reflection: yr=−0.3cos(2.0x+40t)
2. Free End Reflection: yr=0.3cos(2.0x+40t)
Topper's Solved these Questions
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Subjective Questions
6 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 Single Correct
15 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Objective Questions
1 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary
30 Videos
Similar Questions
The harmonic wave yi=(2.0×10−3)cosπ(2.0x−50t) travels along a string towards a boundary at x=0 with a second string. The wave speed on the second string is 50m/s. Write expressions for reflected and transmitted waves. Assume SI units.
View SolutionA wave y=asin(ωt−kx) on a string meets with another wave producing a node at x=0. Then the equation of the unknown wave is
View SolutionThe equation of a transverse wave travelling along a string is y(xt)=0.3sin(200x−0.5t)
what is the equation of the reflected wave when it is reflected by
(i) A rigid boundary?
(ii) A free end?
View SolutionA wave y= 3mm sin(2πx−200πt) is
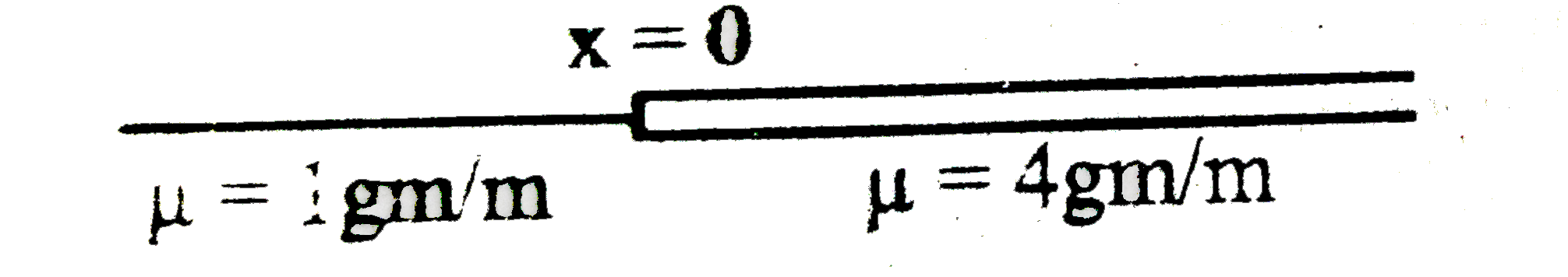
Propagating in the left string. It strikes the boundary at x=0 and is reflected partially and tranmitted partially. Choose the corrects statements (s)
View SolutionA travelling wave y=Asin(kx−ωt+θ) passes from a heavier string to a lighter string. The reflected wave has amplitude 0.5A . The junction of the strings is at x=0. The equation fo the refelcted wave is :
View SolutionA travelling wave y=Asin(kx−ωt+θ) passes from a heavier string to a lighter string. The reflected wave has amplitude 0.5A. The junction of the string is at x=0 The equation of the reflected is :
View SolutionA travelling wave y=Asin(kx−ωt+θ) passes from a heavier string to a lighter string . The juction of the strings is at x=0. The equation of the reflected wave is
View SolutionTwo pulses travelling in opposite directions along a string are shown for t=0 in the figure. Plot the shape of the string at t= 1.0, 2.0, 3.0, 4.0 and 5.0s respectively .

View SolutionA wave frequency 100Hz travels along a string towards its fixed end . When this wave travels back after reflection , a node is formed at a distance of 10cmfrom the fixed end . The speed of the wave (incident and reflected) is
View SolutionA harmonic wave is travelling on string 1. At a junction with string 2 it is partly reflected and partly transmitted. The linear mass density of the second string is four times that of the first string, and that the boundary between the two strings is at x=0. If the expression for the incident wave is
yi=Aicos(k1x−ω1t)
(a) What are the expressions for the transmitted and the reflected waves in terms of Ai,k1 and ω1?
(b) Show that the average power carried by the incident wave is equal to the sum of the average power carried by the transmitted and reflected waves.
View Solution
