A monkey pulls the midpoint of a 10cm long light inextensible string connecting two identical objects A and B lying on smooth table of masses 0.3kg continuously along the perpendicular objects of line joining the masses .The masses are found to apporoach each other at a relative acceleration of 5ms−2 when they are 6cm apart .The constant force applied by monkey is
4N
2N
3N
None of these
The correct Answer is:B
To solve the problem step by step, we will analyze the forces acting on the two masses and the geometry of the situation.
Step 1: Understand the setup
We have two identical masses A and B each with a mass of 0.3kg connected by a 10cm long inextensible string. The monkey pulls the midpoint of the string, causing the masses to approach each other.
Step 2: Determine the distance between the masses
When the masses are 6cm apart, the distance from the midpoint to each mass is 3cm.
Step 3: Calculate the relative acceleration
The problem states that the relative acceleration of the two masses is 5m/s2. Since both masses are identical and are accelerating towards each other, the acceleration of each mass a can be calculated as:
a=ar2=5m/s22=2.5m/s2
Step 4: Analyze the forces acting on the masses
Let F be the force exerted by the monkey. The tension T in the string acts on both masses. The component of tension that pulls each mass towards the other is Tsinθ, where θ is the angle between the string and the line joining the masses.
Step 5: Determine the geometry of the situation
In the triangle formed by the string and the line joining the masses:
- The total length of the string is 10cm, so each half is 5cm.
- When the masses are 6cm apart, the horizontal distance from the midpoint to each mass is 3cm.
- Using Pythagoras' theorem, we can find the vertical distance y:
y=√52−32=√25−9=√16=4cm
Step 6: Calculate tanθ
From the triangle, we can find tanθ:
tanθ=oppositeadjacent=4cm3cm=43
Step 7: Relate the forces and acceleration
The net force acting on each mass can be expressed as:
Tsinθ=ma
Substituting T=F2cosθ into the equation gives:
F2cosθsinθ=ma
Rearranging gives:
F=2macosθsinθ=2macotθ
Step 8: Substitute known values
Substituting m=0.3kg, a=2.5m/s2, and cotθ=34:
F=2×0.3×2.5×34
Calculating this gives:
F=2×0.3×2.5×0.75=2.25N
Step 9: Final Calculation
Thus, the force F exerted by the monkey is:
F=2N
Conclusion
The constant force applied by the monkey is 2N.
We have two identical masses A and B each with a mass of 0.3kg connected by a 10cm long inextensible string. The monkey pulls the midpoint of the string, causing the masses to approach each other.
When the masses are 6cm apart, the distance from the midpoint to each mass is 3cm.
The problem states that the relative acceleration of the two masses is 5m/s2. Since both masses are identical and are accelerating towards each other, the acceleration of each mass a can be calculated as:
a=ar2=5m/s22=2.5m/s2
Let F be the force exerted by the monkey. The tension T in the string acts on both masses. The component of tension that pulls each mass towards the other is Tsinθ, where θ is the angle between the string and the line joining the masses.
In the triangle formed by the string and the line joining the masses:
- The total length of the string is 10cm, so each half is 5cm.
- When the masses are 6cm apart, the horizontal distance from the midpoint to each mass is 3cm.
- Using Pythagoras' theorem, we can find the vertical distance y:
y=√52−32=√25−9=√16=4cm
From the triangle, we can find tanθ:
tanθ=oppositeadjacent=4cm3cm=43
The net force acting on each mass can be expressed as:
Tsinθ=ma
Substituting T=F2cosθ into the equation gives:
F2cosθsinθ=ma
Rearranging gives:
F=2macosθsinθ=2macotθ
Substituting m=0.3kg, a=2.5m/s2, and cotθ=34:
F=2×0.3×2.5×34
Calculating this gives:
F=2×0.3×2.5×0.75=2.25N
Thus, the force F exerted by the monkey is:
F=2N
The constant force applied by the monkey is 2N.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Subjective Question
27 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Single Correct
90 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
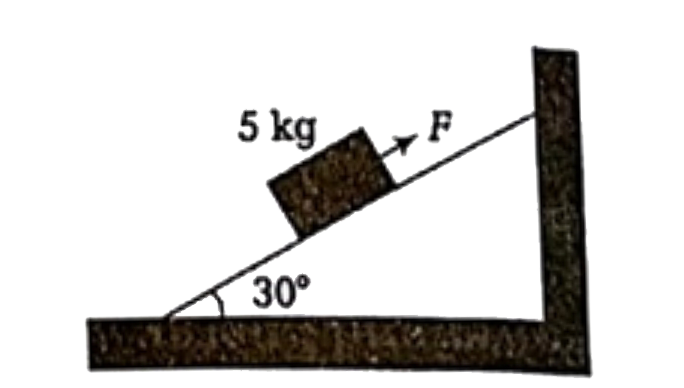
A force F=75 N is applied on a block of mass 5 kg along the fixed smooth incline as as shown in the figure. Here gravitational acceleration g = 10 m s−2 . The acceleration of the block is
View SolutionA body is moving with a velocity 5m/s along a striaght line.A force of 10N is applied to the body perpendicular to the line of motion. If the body is of mass 2kg, then the acceleration of the body along the initial straight line of motion is :
View SolutionTwo smooth blocks of masses m and m' connected by a light inextensible strings are moving on a smooth wedge of mass M. If a force F acts on the wedge the blocks do not slide relative to the wedge. Find the (a) acceleration of the wedge and (b) value of F.
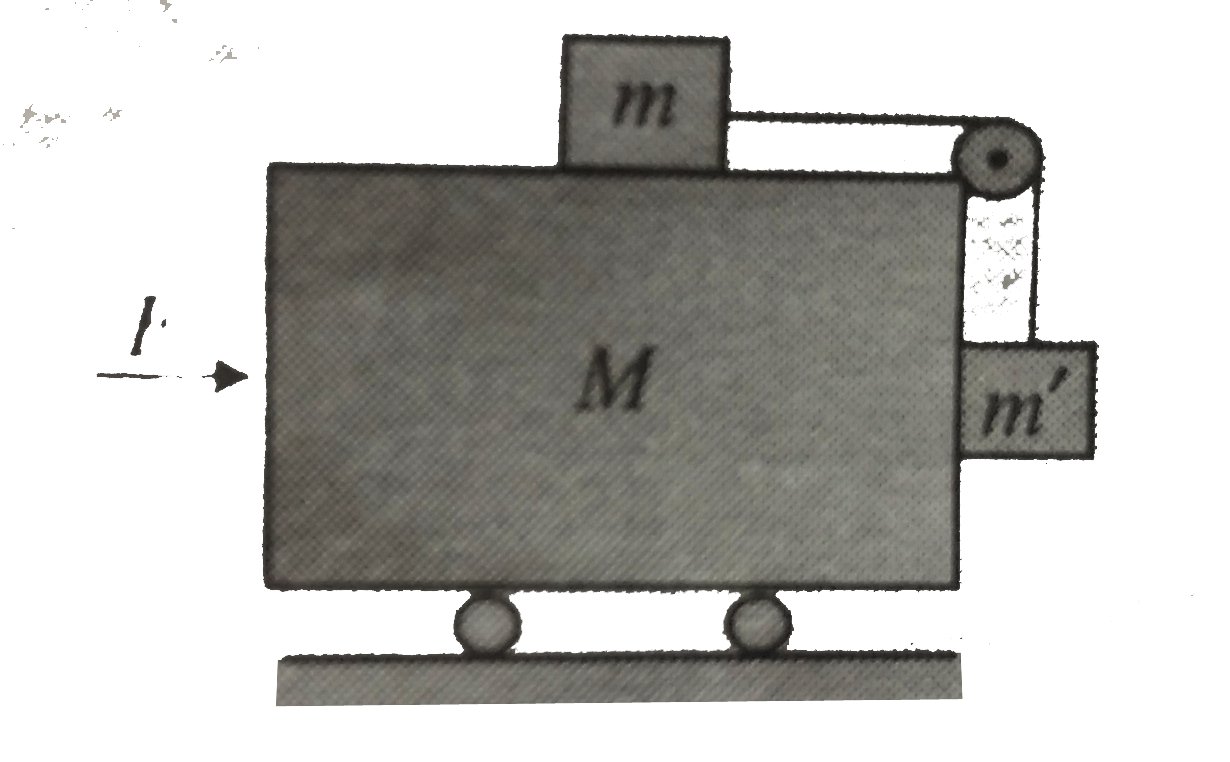
View SolutionTwo smooth blocks of masses m and m' connected by a light inextensible strings are moving on a smooth wedge of mass M. If a force F acts on the wedge the blocks do not slide relative to the wedge. Find the (a) acceleration of the wedge and (b) value of F.

View SolutionTwo smooth blocks of masses m and m' connected by a light inextensible strings are moving on a smooth wedge of mass M. If a force F acts on the wedge the blocks do not slide relative to the wedge. Find the (a) acceleration of the wedge and (b) value of F.

View SolutionTwo bodies of masses 4 kg and 6 kg connected by means of a light string are lying on a smooth horizontal surface. A horizontal pulling force is applied on the lighter body. Two seconds later the string connecting the two masses is cut. After two more seconds if the velocity of the heavier mass is 2ms−1, the force initially applied is
View SolutionTwo blocks A and B of masses 2kg and 3kg are connected by a light string as shown in the figure and placed on a horizontal surface. μ between all surfaces is 0.1 and g = 10ms−1. The acceleration of the system is, when the force applied F = 45N
View SolutionTwo blocks each of mass 4 kg connected by a light string which is passing over an ideal pulley is as shown in figure. If the system is released from rest then the acceleration (in m/s2) of centre of mass is (g = 10 m/s2)
View SolutionA block of mass 2 kg hangs by an inextensible light string that passes over smooth pulley Another mass 1 kg hangs from the other end of the string and is connected with a vertical massless spring . The system is stationary. Just after the string is cut, the acceleraion of 1 kg and 2 kg are respectively . Given that g=10m/s2

View SolutionTwo identical blocks A and B each of mass M are connected to each other through a light string. The system is placed on a smooth horizontal floor. When a constant force F is applied horizontally on the block A, find the tension in the string.
View Solution
