Question

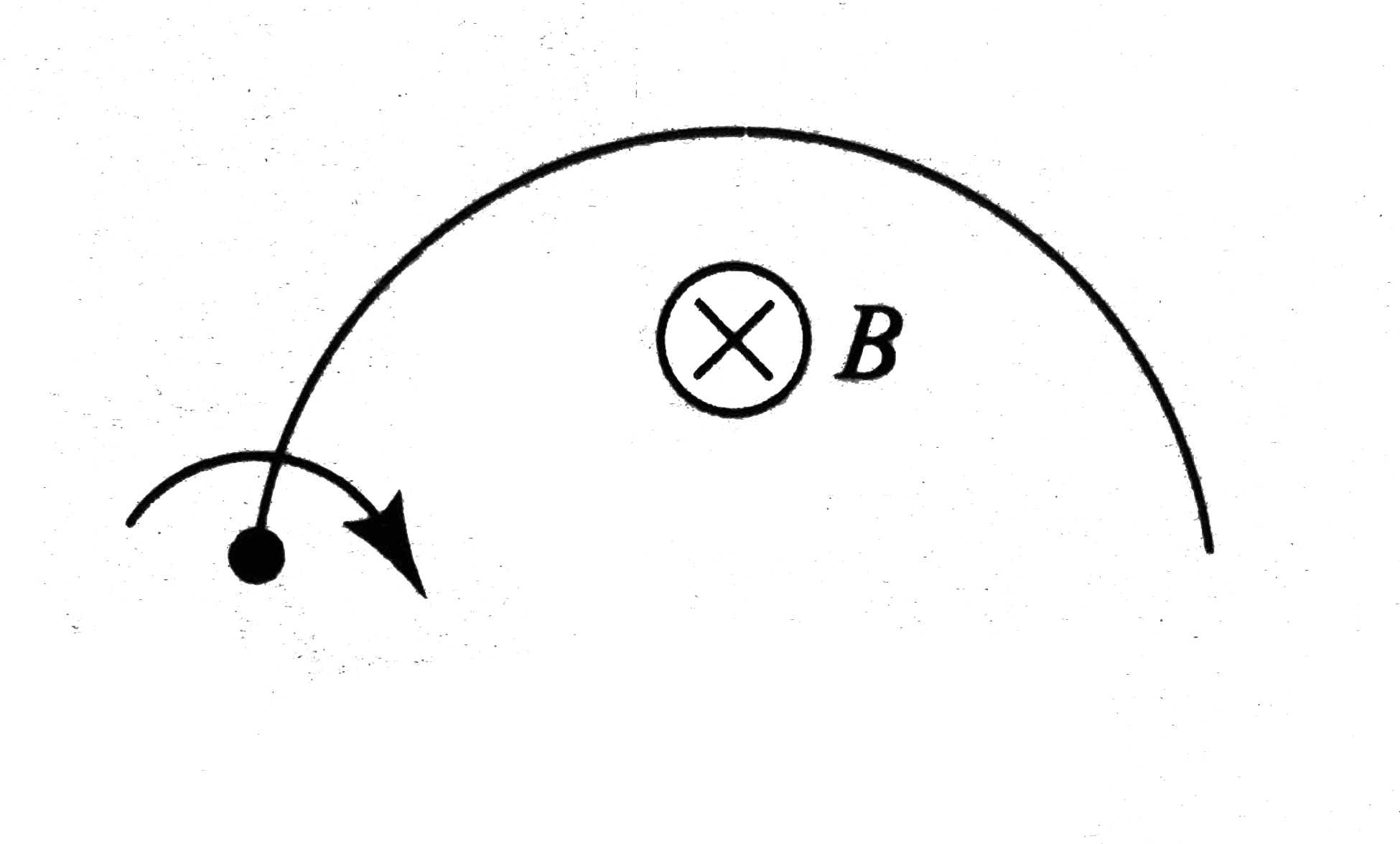
A metallic rod of 1 m length is rotated with a frequency of 50 $rev/s$, with on end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular at to the plane of the ring. A constant uniform magnetic field of 1 T parallel to the axis is persent eveywhere. what is the e.m.f. between the centre and the metallic ring?

Options

A. 157V
B. 117V
C. 127V
D. 137V

Answer

A

Explanation

To solve the problem of finding the electromotive force (e.m.f.) generated between the center and the metallic ring due to the rotation of the rod in a magnetic field, we can follow these steps:

1. Identify the given values:
- Length of the rod (L) = 1 m
- Frequency (f) = 50 rev/s
- Magnetic field (B) = 1 T

2. Convert frequency to angular velocity (ω):
- The angular velocity (ω) can be calculated using the formula:
\( \omega = 2\pi f \)
- Substituting the value of frequency:
\( \omega = 2\pi \times 50 = 100\pi \, \text{rad/s} \)

3. Use the formula for e.m.f. (E):
- The formula for the induced e.m.f. in a rotating rod in a magnetic field is:
\( E = \frac{1}{2} B \omega L^2 \)

4. Substitute the values into the e.m.f. formula:
- Now substituting B, ω, and L into the formula:
\( E = \frac{1}{2} \times 1 \, \text{T} \times (100\pi \, \text{rad/s}) \times (1 \, \text{m})^2 \)
- This simplifies to:
\( E = \frac{1}{2} \times 1 \times 100\pi \times 1 \)
\( E = 50\pi \, \text{V} \)

5. Calculate the numerical value:
- Approximating π as 3.14:
\( E \approx 50 \times 3.14 = 157 \, \text{V} \)

Final Answer:
The e.m.f. between the center and the metallic ring is approximately 157 V.

---