Question

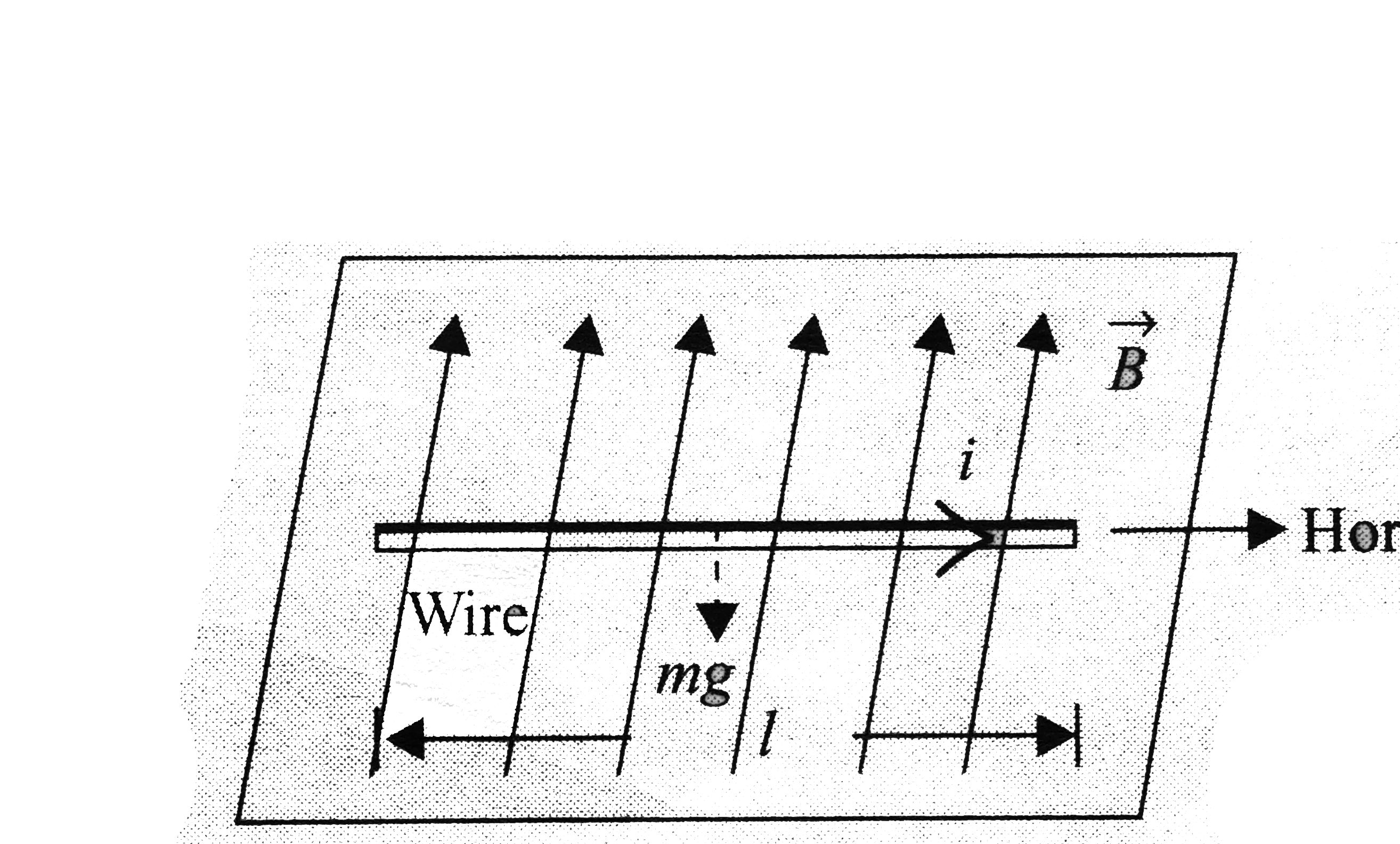
A 2.5 m long straight wire having mass of 500 g is suspended in mid air by a uniform horizontal magnetic field B. If a current of 4 A is passing through the wire then the magnitude of the field is $\left(\text{Take g}10m{s}^{-2}\right)$

Options

A. 0.5T
B. 0.6T
C. 0.25T
D. 0.8T

Answer

A

Explanation

To solve the problem, we need to find the magnitude of the magnetic field $$B$$ that can suspend a wire of length $$L$$ and mass $$m$$ when a current $$I$$ is flowing through it. The magnetic force acting on the wire must equal the weight of the wire for it to be suspended in mid-air.

1. Identify the Forces Acting on the Wire:
The wire experiences two main forces: the magnetic force \( F_m \) acting upwards and the gravitational force \( F_g \) acting downwards. For the wire to be suspended in mid-air, these forces must be equal:
\( F_m = F_g \)

2. Express the Gravitational Force:
The gravitational force \( F_g \) can be calculated using the formula:
\( F_g = mg \)
where:
- \( m = 500 \text{ g} = 0.5 \text{ kg} \) (convert grams to kilograms)
- \( g = 10 \text{ m/s}^2 \)

Thus,
\( F_g = 0.5 \text{ kg} \times 10 \text{ m/s}^2 = 5 \text{ N} \)

3. Express the Magnetic Force:
The magnetic force \( F_m \) on a current-carrying wire in a magnetic field is given by:
\( F_m = BIL \)
where:
- \( B \) is the magnetic field strength,
- \( I = 4 \text{ A} \) (current),
- \( L = 2.5 \text{ m} \) (length of the wire).

4. Set the Forces Equal:
Since \( F_m = F_g \), we can set the equations equal to each other:
\( BIL = mg \)

5. Rearrange to Solve for \( B \):
Rearranging the equation gives us:
\( B = \frac{mg}{IL} \)

6. Substitute the Known Values:
Now, substitute the values into the equation:
\( B = \frac{(0.5 \text{ kg})(10 \text{ m/s}^2)}{(4 \text{ A})(2.5 \text{ m})} \)

7. Calculate:
\( B = \frac{5 \text{ N}}{10 \text{ A m}} = 0.5 \text{ T} \)

Final Answer:
The magnitude of the magnetic field \( B \) is \( 0.5 \text{ T} \) (Tesla).