The logical statement (p→q)∨(q→~p) is :
A
~p
B
~q
C
t (tautology)
D
F (fallacy)
Video Solution
Text Solution
The correct Answer is:C
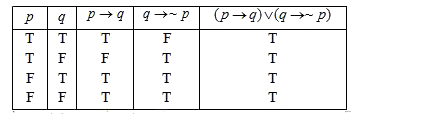
Given statement is a tautology .
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The logical statement (~q⇒~p)∨(~q⇒p) is equivalent to :
Ap→qBq→pC~(~p→q)DTautology - Question 2 - Select One
(p∧q)∨(~q∧p)≡
Aq∨pBPC~qDp∧q - Question 3 - Select One
The statement p∨q is
Aa tautologyBa contradictionCcontingencyDnone of these
The logical statement [~(~p∨q)∨(p∧r)]∧(~q∧r) is equivalent to (a) (~p∧~q)∧r (b) ~p∨r (c) (p∧r)∧~q (d) (p∧~q)∨r
View SolutionThe statement
(p→(q→p))→(p→(p∨q)) is :View SolutionThe nagation of statement (p∧q)∨(q∨~r)
View SolutionIf p and q are logical statements, then p⇒(~q⇒p) is equivalent to
View SolutionThe logically equivalent statement of
(p∧q)∨(p∧r) isView Solution
