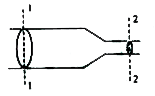
The area of cross section of a current carrying conductor is A0 and A04 at section (1) and (2) respectively. If vα1,vα2andE1,E2 be the drift velocity and electric field at sections 1 and 2 respectively then :
 Solution in Telugu
Solution in Telugu
vα1:vα2=1:4
vα1:vα2=4:1
E1:E2=4:1
E1:E2=1:4
The correct Answer is:A, D
Step by step video, text & image solution for The area of cross section of a current carrying conductor is A_(0) and (A_(0))/(4) at section (1) and (2) respectively. If v_(alpha_(1)),v_(alpha_(2)) and E_(1), E_(2) be the drift velocity and electric field at sections 1 and 2 respectively then : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE- II LEVEL-I (MAIN) (Straight Objective Type Questions))
14 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE- II LEVEL- II (ADVANCED) (Straight Objective Type Questions))
11 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE-I LEVEL- II (ADVANCED) (Straight Objective Type Questions))
4 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
44 Videos
Similar Questions
The resistance of the wire is 121 ohm. It is divided into .n. equal parts and they are connected in parallel, then effective resistance is 1 ohm. The value of .n. is
View SolutionA thick spherical shell has inner radius a and outer b. The material has resistivity ρ . When a potential difference is applied between the inner and outer surfaces, its resistance is
View SolutionThe charge flowing in a conductor varies with time as Q=at−12bt2+16ct3 where a, b and c are positive constants. If at time t, the current in the conductor is i, which of the following graphs is correct ?
View SolutionA battery of emf 10 V and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 0.5 A, what is the resistance of the resistor? What is the terminal voltage of the battery when the circuit is closed?
View SolutionTwo separate monochromatic light beams A and B of the same intensity are falling normally on a unit area of a metallic surface. Their wave lengths are λA and λB, respectively. Assuming that all the incident light is used in ejecting the photoelectrons the ratio of the photoelectrons from the beam A to that from B is :
View SolutionA liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h . Then
View SolutionA liquid flows through a horizontal tube as shown in figure. The velocities of the liquid in the two sections, which have areas of cross-section A1 and A2 and v1 and v2 respectively. The difference in the levels of the liquid in the two vertical tubes is h, then

View SolutionThe velocity of an electron at point A_(1) is V_(0) where cross sectional area is A. The velocity of electron at the end of contraction at point B, where cross sectional area is 2A, is V_(1) . Find the correct option:
View SolutionIf cross- sectional area of limb I is A1 and that of limb II is A2 then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)

View SolutionGiven a1cosα1+α2cosα2+…+ancosαn=0 and a1cos(α1+θ)+a2cos(α2+θ)+…+ancos(αn+θ)=0(θ≠kπ), then the value of a1cos(α1+λ)+a2cos(α2+λ)+…+ancos(αn+λ) is
View SolutionProve that ∣∣ ∣ ∣∣a1α1+b1β1a1α2+b1β2a1α3+b1β3a2α1+b2β1a2α2+b2β2a2α3+b2β3a3α1+b3β1a3α2+b3β2a3α3+b3β3∣∣ ∣ ∣∣=0.
View SolutionGiven a1cosα1+α2cosα2+…+ancosαn=0 and a1cos(α1+θ)+a2cos(α2+θ)+…+ancos(αn+θ)=0(θ≠kπ), then the value of a1cos(α1+λ)+a2cos(α2+λ)+…+ancos(αn+λ) is
View SolutionA liquid flows through a horizontal tube. The velocities of the liquid ind the two sections, which have areas of cross -section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h. a. The volume of the liquid flow through the tube in unit tims is A_(1)v_(1) b. v_(2)-v_(1)=sqrt(2gh) c. v_(2)^(2)-v_(1)^(2)=2gh d. The energy per unit mass of the liquid is the same in both sections of the tube.
View SolutionA liquid flows through a horizontal tube as shown in figure. The velocities of the liquid in the two sections, which have areas of cross-section A1 and A2 and v1 and v2 respectively. The differnece in the levels of the liquid in the two vertical tubes is h. then

View SolutionIf cross- sectional area of limb I is A1 and that of limb II is A2 then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)

View SolutionProve that ∣∣ ∣ ∣∣a1α1+b1β1a1α2+b1β2a1α3+b1β3a2α1+b2β1a2α2+b2β2a2α3+b2β3a3α1+b3β1a3α2+b3β2a3α3+b3β3∣∣ ∣ ∣∣=0.
View Solution
