The locus of the point at which two given unequal circles subtend equal angles is: (A) a straight line(B) a circle (C) a parabola (D) an ellipse
a straihght line
a circle
a parabola
none of these
The correct Answer is:B
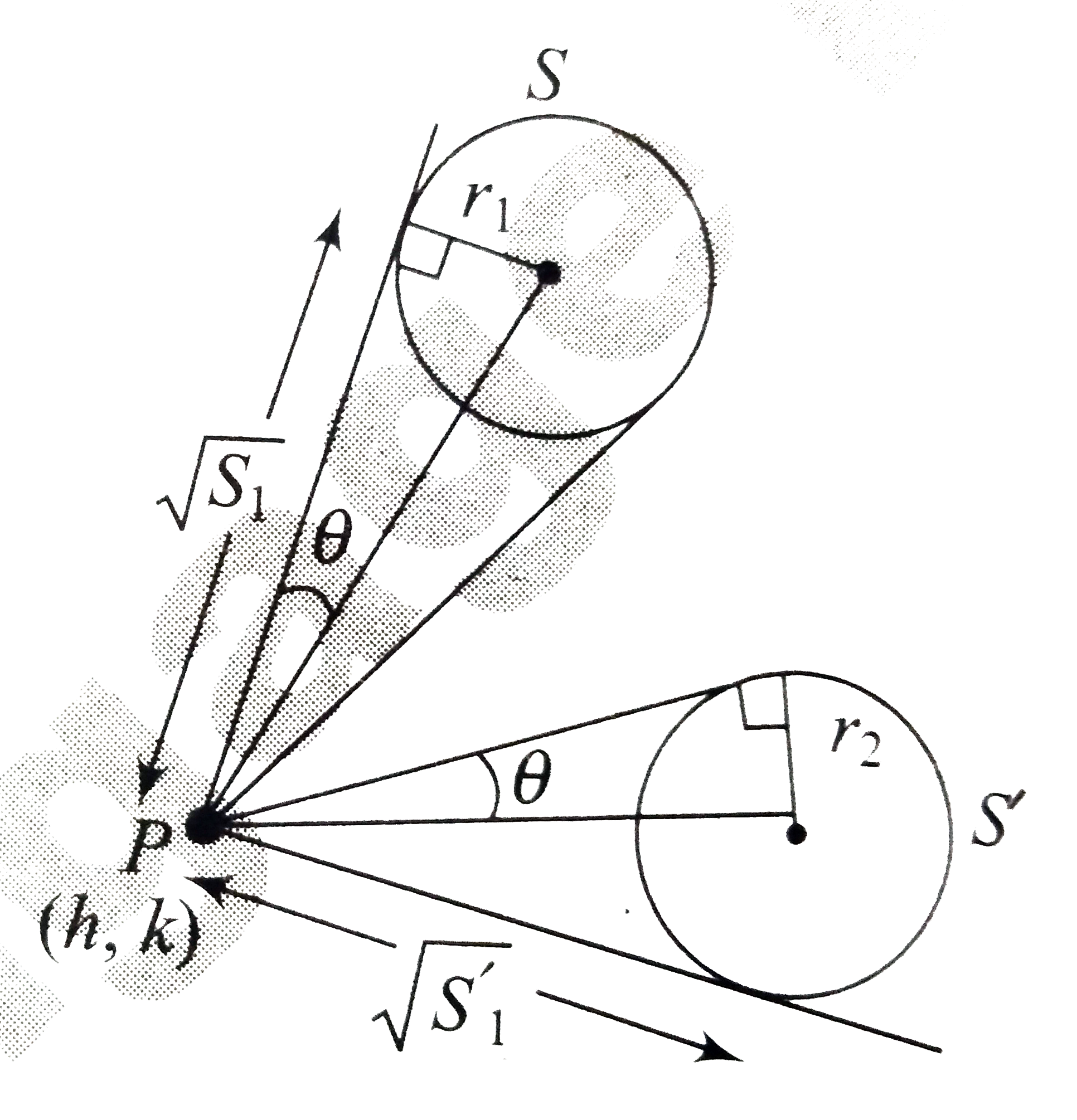
Let two circles be S=0 nd S'=0 having radii r1 and r2. Respectively
From the figure, we have
r1√S1=r2√S'1
⇒S'1r21=r22S1
⇒S1−(r1r2)2S'1=0
∴ Locus of P(h,k)
S−(r1r2)2S'=0, which represents the equation of a circle,
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Multiple Correct Answers Type
9 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE
7 Videos
- COMPLEX NUMBERSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERSEXERCISE - MATRIX MATCH TYPE
9 Videos
Similar Questions
The locus of the points of trisection of the double ordinates of the parabola y2=4ax is : (A) a straight line (B) a circle (C) a parabola (D) none of these
View SolutionFind the locus of the point at which two given portions of the straight line subtend equal angle.
View SolutionIf Re(2z+1iz+1)=1, the the locus of the point representing z in the complex plane is a (A) straight line (B) circle (C) parabola (D) none of these
View SolutionThe locus of the centre of a circle which touches two given circles externally is a
View SolutionThe locus of the centre of a circle which cuts a given circle orthogonally and also touches a given straight line is (a) circle (c) parabola (b) line (d) ellipse
View SolutionConsider a curve ax2+2hxy+by2=1 and a point P not on the curve. A line drawn from the point P intersect the curve at points Q and R. If he product PQ.PR is (A) a pair of straight line (B) a circle (C) a parabola (D) an ellipse or hyperbola
View SolutionThe locus of the point (√3h,√3k+2) if it lies on the line x−y−1=0 is (a) straight line (b) a circle (c) a parabola (d) none of these
View SolutionProve Equal chords of a circle subtend equal angles at the centre.
View SolutionProve Equal chords of congruent circles subtend equal angles at the centre.
View SolutionProve that equal chords of congruent circles subtend equal angles at their centre.
View Solution
