Find the area enclosed by y=g(x), x-axis, x=1 and x=37, where g(x) is inverse of f(x)=x3+3x+1.
Required area, A=37∫1g(x)dx=37∫1f−1(x)dx.
Let f−1(x)=torx=f(t)
Using intelligent gusessing, f(3)=37andf(0)=1
∴ A=3∫0tf'(t)dt=[tf(t)]30−3∫0f(t)dt
=3f(3)−3∫0(t3+3t+1)dt
=111−1474=2974
Alternative method :
f(x)=x3+3x+1.
∴ f'(x)=3x2+3>0,∀∈R.
∴ f(x) is an increasing function.
Also, x3+3x+1=xorx3+2x+1=0 has no positive root.
So, line y=x never meet curve y=f(x) for x>0.
Graph of y=f(x)andy=f−1(x) are as shown in the following figure.
When y=1,x3+3x+1=1,x=0.
When y=37,x3+3x=36,x=3
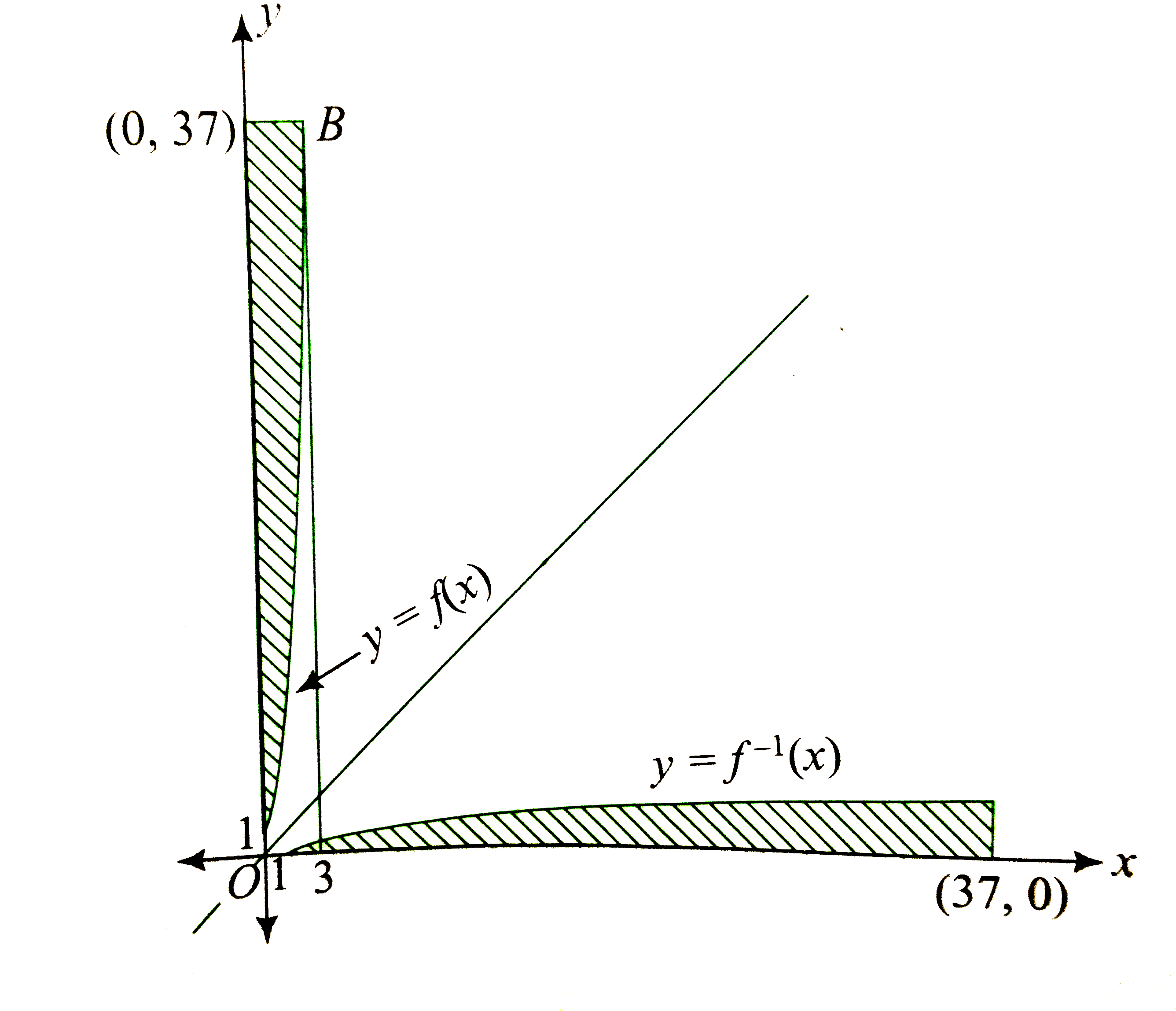
Area bounded by curves y=f−1(x), x-axis, x=1 and x=37 is same as area bounded by curves y=f(x),y-axis, y=1andy=37.
∴ Required area =3∫0(37−x3−3x−1)dx
=[36x−x44−3x22]30=2974 sq. units.
Topper's Solved these Questions
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Solved Examples
10 Videos
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Concept Application Exercise 9.1
9 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Comprehension Type
5 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
Similar Questions
If the area enclosed by g(x),x=−3,x=5 and x-axis where g(x) is the inverse f(x)=x3+3x+1 is A, then [A] is
View SolutionFind the area enclosed between curve y=x2+2, x-axis, x=1 and x=2.
View SolutionFind the area enclosed by y=loge(x+e) and x=loge(1y) and the x-axis.
View SolutionFind the area enclosed betweent the curve y=x2+3,y=0,x=−1,x=2.
View SolutionFind the area of the region enclosed by the curves
y=x2−4x+3 and the x-axis
View Solutionf(x)=xx,x∈(0,∞) and let g(x) be inverse of f(x) , then g(x)' must be
View SolutionFind area bounded by y=f−1(x),x=4,x=10 and the x-axis, given that the area bounded by y=f(x),x=2,x=6 and the x-axis is 30 sq. units, where f(2)=4 and f(6)=10, and that f(x) is an invertible function.
View SolutionLet the area bounded by the curve y=f(x), x-axis and the ordinates x=1 and x=a be (a−1)sin(3a+4).
Statement-1: f(x)=sin(3x+4)+3(x−1)cos(3x+4).
Statement-2: If y=∫h(x)g(x)f(t)dt, then dydx=f(h(x))h'(x)−f(g(x))g'(x).
(A) Both 1 and 2 are true
(B) Both 1 and 2 are false
(C) 1 is true but 2 is false
(D) 1 is false but 2 is true
View SolutionA point chanrge of magnitude q=4.5nC is moving with speed v=3.6×107ms−1 parallel to the x-axis along the line y=3m. Find the magnetic field at the origin produced by this charge when the charge is at the point x=−4m, y=3m, as shown in Fig.

View SolutionIf g(x)=3x−1andf(x)=4g(x)+3. What is f(2)?
View Solution
