Question

The area common to regions $x^2+y^2-2x\le 0$
and ygeq sin (pix/2)

Explanation

`"For "x^(2)+y^(2)-2xle0`
points lie on or inside circle `(x-1)^(2)+y^(2)=1`
`"For "yge sin""(pix)/(2),` points lie on or above `y=sin""(pix)/(2).`
`y=sin""(pix)/(2)` has period 4.
The graphs of curves and the required region is as shown in teh follwoing figure

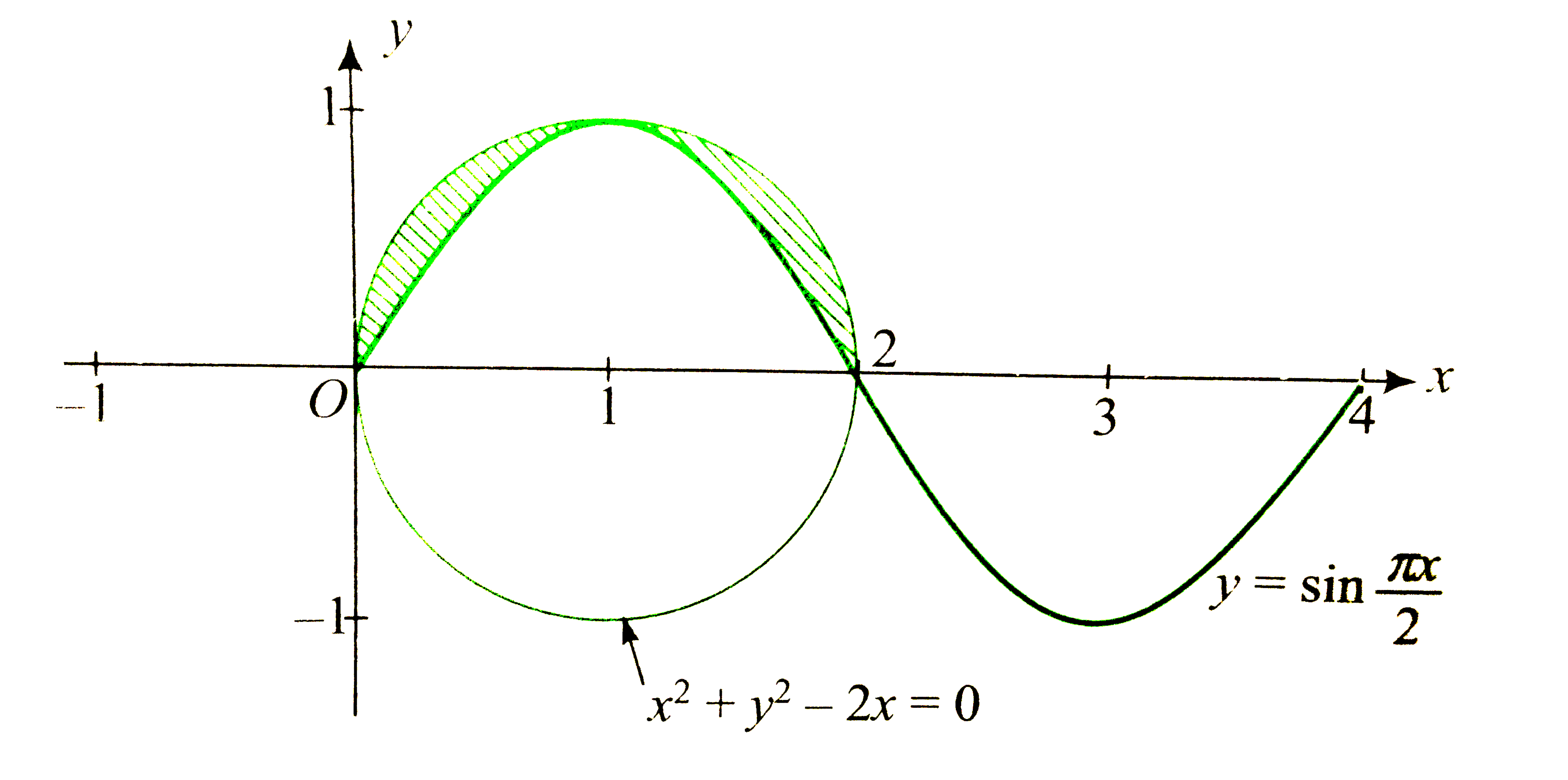
For the figure, required area = area of semicircle `-overset(2)underset(0)int sin""(pix)/(2)dx`
`=(pi)/(2)+(2)/(pi)[cos""(pix)/(2)]_(0)^(2)`
`=(pi)/(2)-(4)/(pi)`