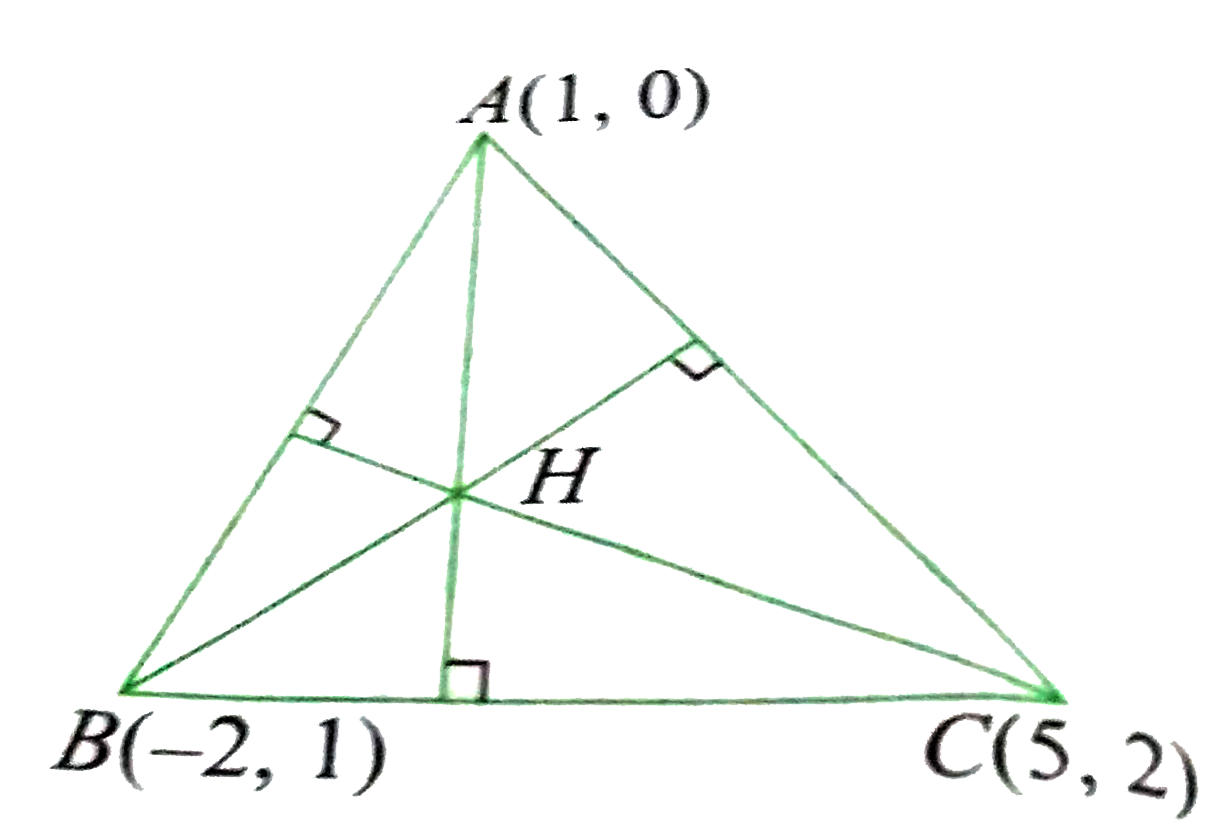
Find the orthocentre of with vertices and
Let the orthocenter be H(h,k).
Slope of
Slope of
Slope of
or
or
Also,
Slope of
or
or
Solving (1) and (2), we get and . Hence, the orthocenter is
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.49
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.50
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.47
1 Videos
- COORDINATE SYSTEMBOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE ENGLISHCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Find the orthocentre of ΔABC with vertices A(1,0),B(−2,1), and C(5,2)
View SolutionFind the orthocentre of ΔABC with vertices A(1,0),B(−2,1), and C(5,2)
View SolutionFind the centroid of ΔABC whose vertices are A(0,−1) , B(−2,5) and C(2,8).
View SolutionFind the centroid of the triangle whose vertices are A(-1,0), B(5, -2) and C(8, 2).
View SolutionFind the angles of △ABC whose vertices are A((−1,3,2),B(2,3,5)andC(3,5,−2).
View SolutionUsing vectors, find the area of the triangle with vertices A(1,1,2), B(2,3,5)and C(1,5,5)
View SolutionUsing vectors, find the area of the triangle with vertices A(1,1,2), B(2,3,5)and C(1,5,5)
View SolutionUsing vectors, find the area of the triangle with vertices A(1,1,2), B(2,3,5)and C(1,5,5)
View SolutionFind the area of the triangle with vertices A(1, 1, 2), B(2, 3, 5) and C(1, 5, 5).
View SolutionUsing integration, find the area of the triangle ABC whose vertices are A(−1,1),B(0,5) and C(3,2).
View Solution
