If area of a triangle is 2 sq. units, then find the value of the product of the arithmetic mean of the lengths of the sides of a triangle and harmonic mean of the lengths of the altitudes of the triangle.
To solve the problem, we need to find the product of the arithmetic mean of the lengths of the sides of a triangle and the harmonic mean of the lengths of the altitudes of the triangle, given that the area of the triangle is 2 square units.
1. Understanding the Area of the Triangle:
The area (Δ) of a triangle can be expressed using the formula:
Δ=12×base×height
Given that the area is 2 square units, we have:
Δ=2
2. Relating Sides and Altitudes:
For a triangle with sides a, b, and c, and corresponding altitudes H1, H2, and H3, we have the relationships:
aH1=bH2=cH3=2Δ
Since Δ=2, we can write:
aH1=bH2=cH3=4
3. Finding the Reciprocals of the Altitudes:
From the above relationships, we can express the reciprocals of the altitudes:
1H1=a4,1H2=b4,1H3=c4
4. Summing the Reciprocals of the Altitudes:
Adding these together gives:
1H1+1H2+1H3=a+b+c4
5. Finding the Arithmetic Mean of the Sides:
The arithmetic mean (AM) of the sides of the triangle is given by:
AM=a+b+c3
6. Finding the Harmonic Mean of the Altitudes:
The harmonic mean (HM) of the altitudes is given by:
HM=31H1+1H2+1H3=3×4a+b+c
7. Calculating the Product of AM and HM:
Now, we need to find the product of the arithmetic mean of the sides and the harmonic mean of the altitudes:
Product=AM×HM=(a+b+c3)×(12a+b+c)
Simplifying this gives:
Product=123=4
Final Answer:
The value of the product of the arithmetic mean of the lengths of the sides of the triangle and the harmonic mean of the lengths of the altitudes of the triangle is 4.
The area (Δ) of a triangle can be expressed using the formula:
Δ=12×base×height
Given that the area is 2 square units, we have:
Δ=2
For a triangle with sides a, b, and c, and corresponding altitudes H1, H2, and H3, we have the relationships:
aH1=bH2=cH3=2Δ
Since Δ=2, we can write:
aH1=bH2=cH3=4
From the above relationships, we can express the reciprocals of the altitudes:
1H1=a4,1H2=b4,1H3=c4
Adding these together gives:
1H1+1H2+1H3=a+b+c4
The arithmetic mean (AM) of the sides of the triangle is given by:
AM=a+b+c3
The harmonic mean (HM) of the altitudes is given by:
HM=31H1+1H2+1H3=3×4a+b+c
Now, we need to find the product of the arithmetic mean of the sides and the harmonic mean of the altitudes:
Product=AM×HM=(a+b+c3)×(12a+b+c)
Simplifying this gives:
Product=123=4
The value of the product of the arithmetic mean of the lengths of the sides of the triangle and the harmonic mean of the lengths of the altitudes of the triangle is 4.
Topper's Solved these Questions
Similar Questions
The product of the arithmetic mean of the lengths of the sides of a triangle and harmonic mean of the lengths of the altitudes of the triangle is equal to: a. 1 b. 2 c. 3 d. 4
View SolutionFind the length of the third side of the triangle.
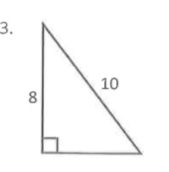
View SolutionFind the length of the third side of the triangle.
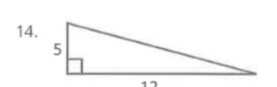
View SolutionIf the lengths of the sides of a triangle are 8, 15 and 17 units, the R the circum-radius is :
View SolutionIf the sides of a triangle are 17,25and28, then find the greatest length of the altitude.
View SolutionIf the sides of a triangle are 17,25and28, then find the greatest length of the altitude.
View SolutionFind the length of side BC in the given triangle.
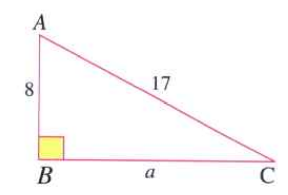
View SolutionIf the lengths of the side of a triangle are 3,4 and 5 units, then find the circum radius R.
View SolutionThe lengths of the sides of a triangle are in the ratio 2:3:4. If the perimeter of the triangle is 63 cm, find the lengths of the sides of the triangle.
View SolutionIf the altitudes of a triangle are in A.P,then the sides of the triangle are in
View Solution
