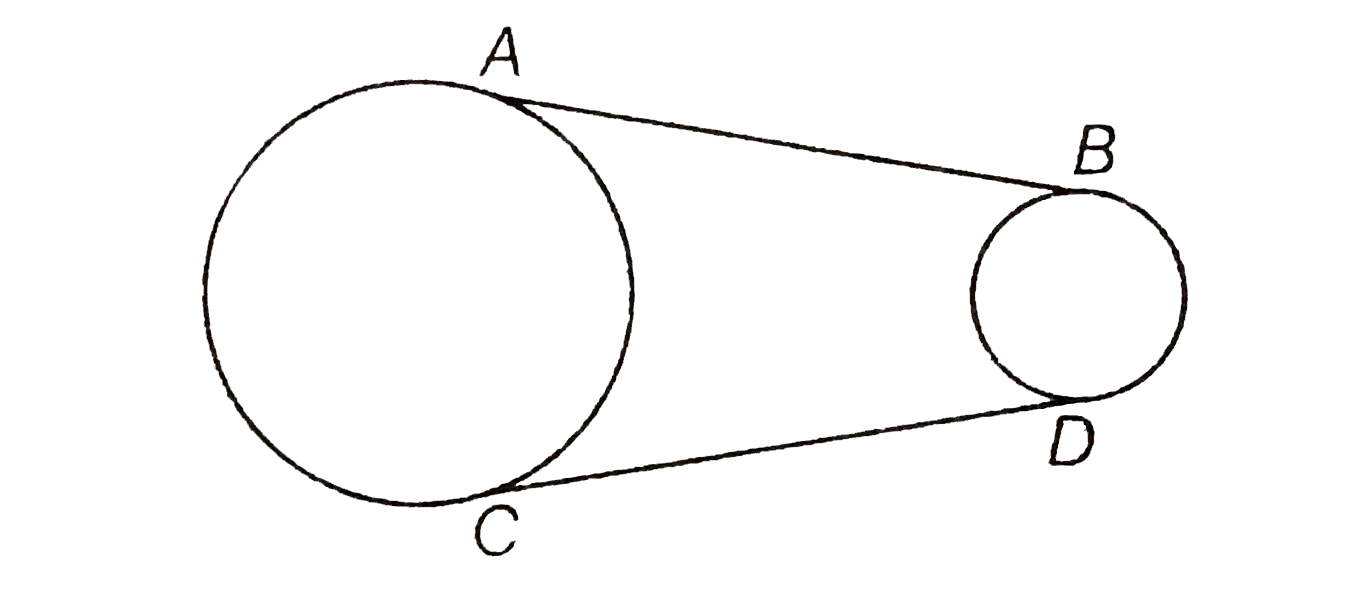
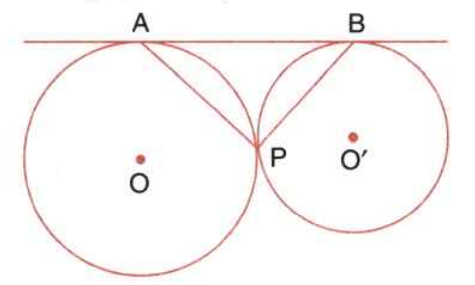
In figure, AB and CD are common tangents to two circles of equal radii. Prove that AB=CD.
Given AB and CD are tangents to two circles of equal radii.
To prove AB=CD
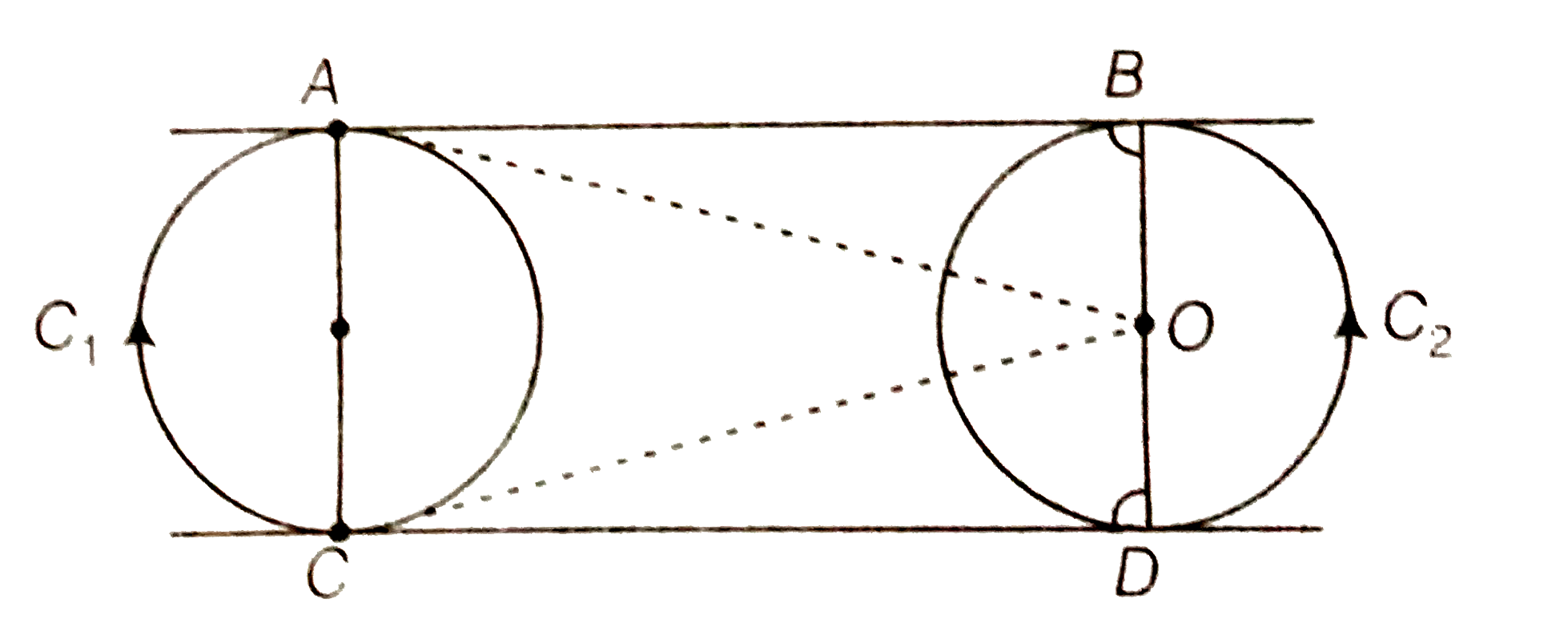
Construction Join OA, OC, O' B and O'D
Proof Now, ∠OAB=90∘
[tangent at any point of a circle is perpendicular to radius through the point of contact]
Thus, AC is a straight line.
Also, ∠OAB+∠OAB=180∘
∴AB/CD
Similarly, BD is a straight line
and ∠O'BA=∠O'DC=90∘
Also, AC=BD [radii of two circles are equal]
In quadrilateral ABCD, ∠A=∠B=∠C=∠D=90∘
and AC=BD
ABCD is a rectangle
Hence, AB=CD [opposite sides of rectangle are equal]
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - EXERCISE 9.4 LONG ANSWER TYPE QUESTIONS
14 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - EXERCISE 9.2 VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- ARITHMETIC PROGRESSIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Long Answer Type Questions
10 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.4 Long Answer type Questions
7 Videos
Similar Questions
Knowledge Check
In the figure, AB and CD are the diameters of the circle. What is the value of x?
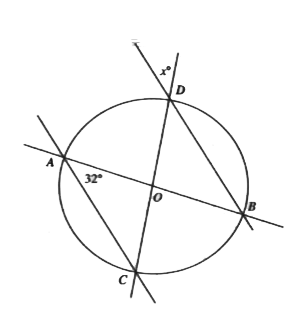 A16∘B18∘C26∘D32∘
A16∘B18∘C26∘D32∘
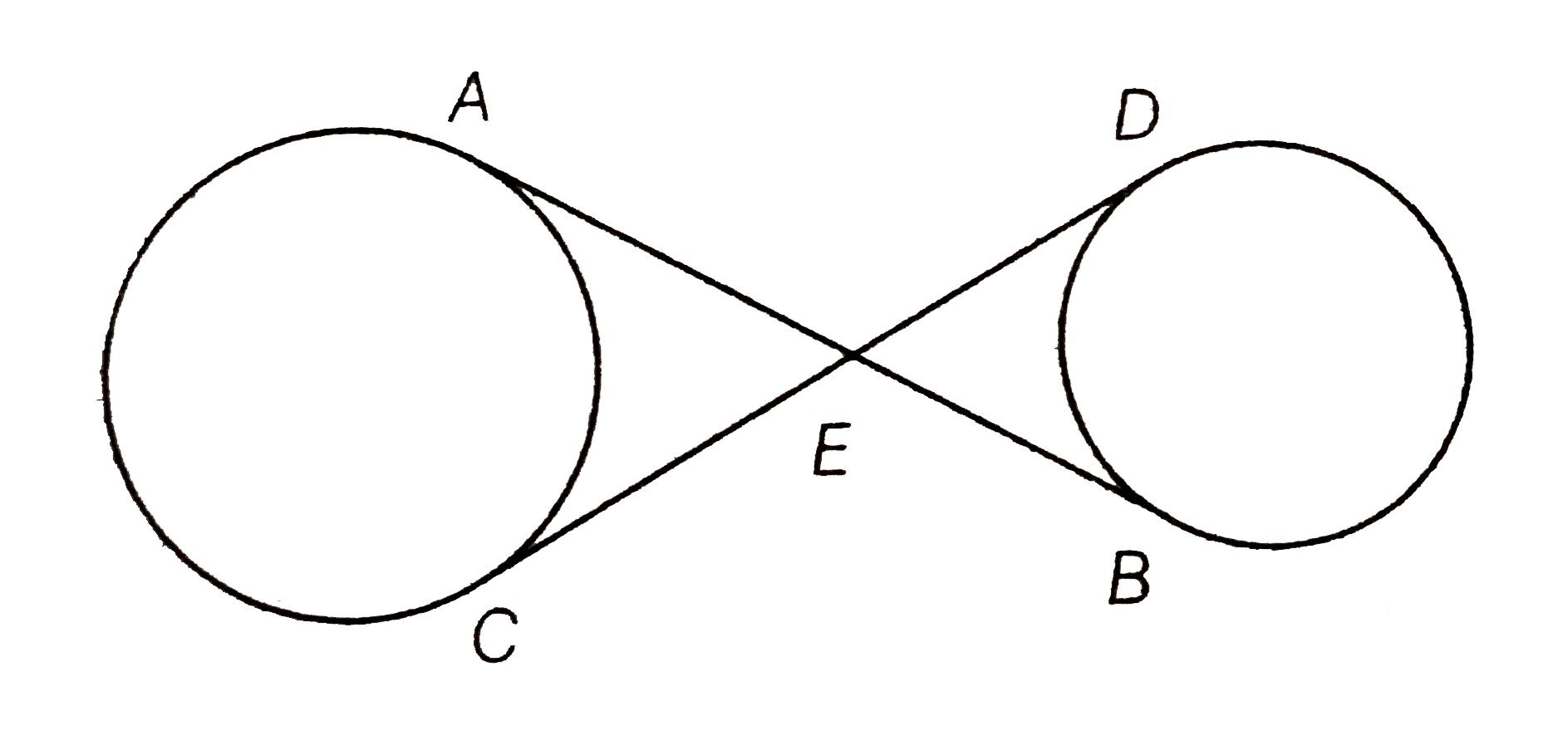
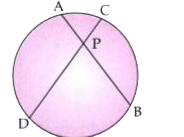
In the adjoining figure, common tangents AB and CD to two circles intersect at P.
Prove that AB=CD.

View SolutionAB and CD are two chords of a circle intersecting at P. Prove that AP×PB=CP×PD
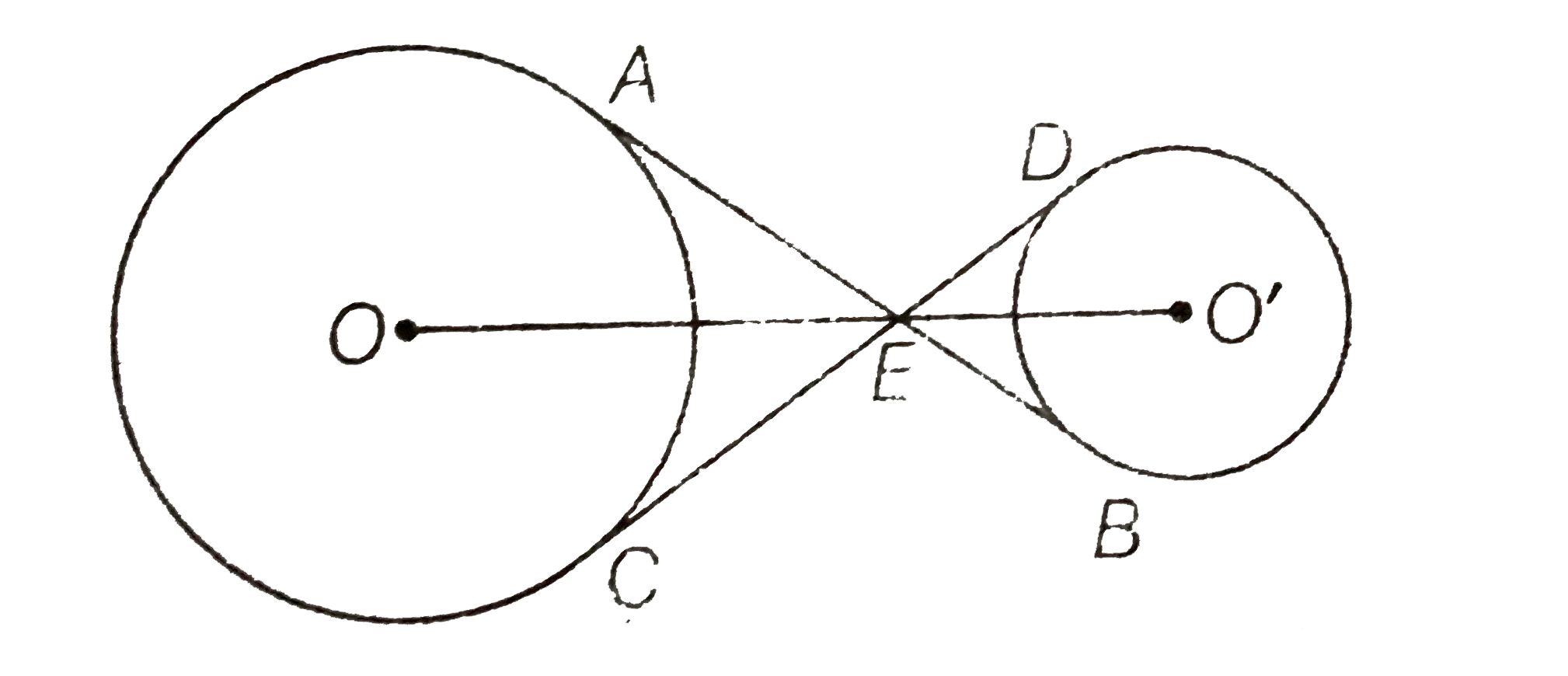
View SolutionIn a figure the common tangents, AB and CD to two circles with centers O and O' intersect at E. Prove that the points O, E and O' are collinear.
View SolutionIn the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:
(i) tangent at point P bisects AB
(ii) angle APB=90∘
View SolutionIn Figure, a quadrilateral ABCD is drawn to circumscribe a circle .
Prove that
AB+CD=BC+AD

View SolutionIn Figure, a quadrilateral ABCD is drawn to circumscribe a circle .
Prove that
AB+CD=BC+AD

View SolutionIn Figure, AB is a diameter of the circle, CD is a chord equal to the radius of the circle. AC and BD when extended intersect at a point E. Prove that ∠AEB=60∘
View Solution