Show that the diagonals of a parallelogram divide it into four triangles of equal area.

Video Solution

Text Solution

Verified by Experts

GIVEN A ||gm ABCD. Its diagonals AC and BD intersect at O.
TO PROVE $a r (△ O A B) = a r (△ O B C)$
$= a r (△ O C D) = a r (△ O A D)$

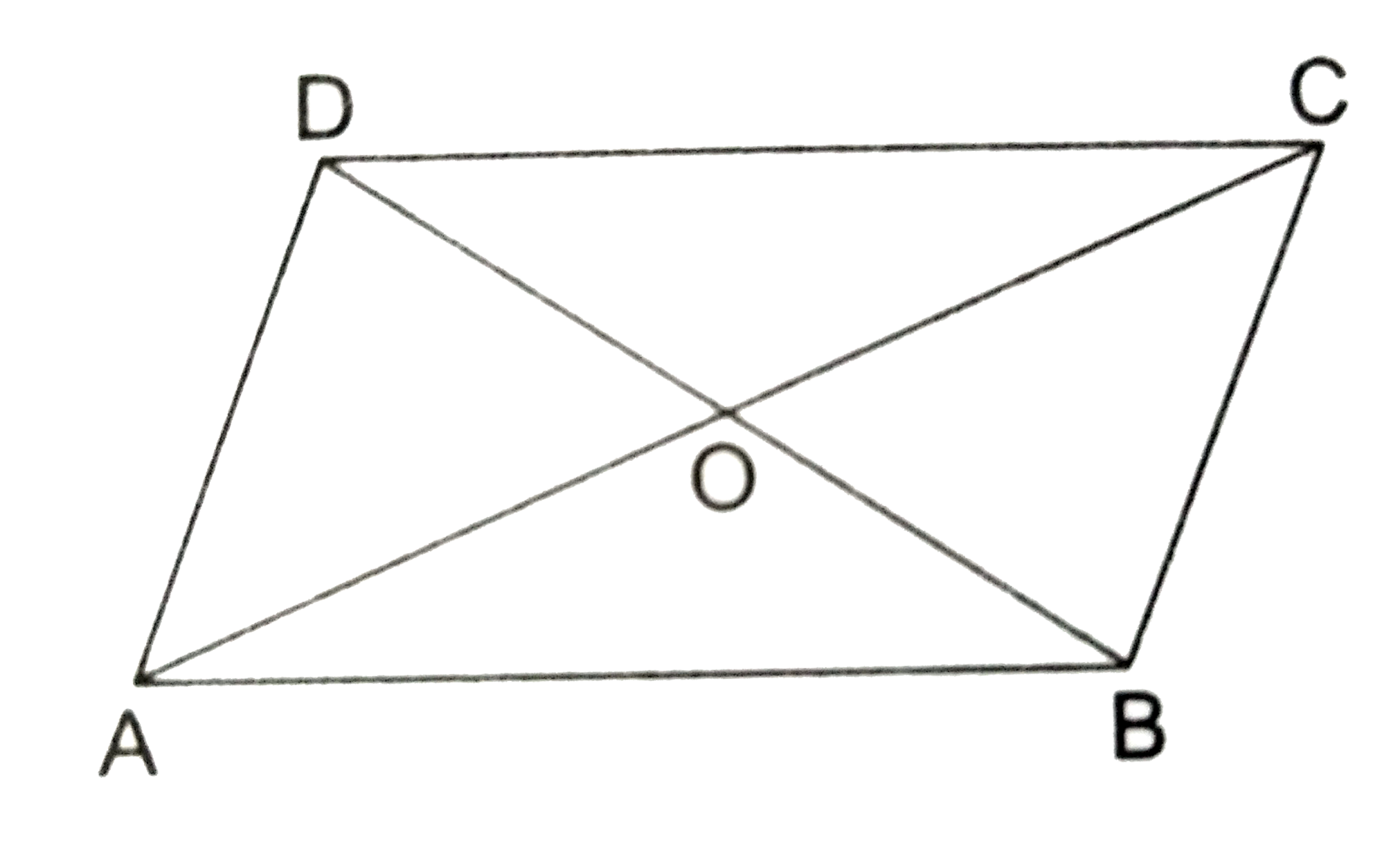
PROOF Since the diagonals of a ||gm bisect each other, we have
$O A = O C and O B = O D$ .
Also, a median of a triangle divides it into two $△$ of equal area.
Now, in $△$ ABC, BO is the median.
$∴ a r (△ O A B) = a r (△ O B C) . ... (i)$
In $△ A B D, A O$ is the median.
$∴ a r (△ O A B) = a r (△ O A D) . ... (i i)$
In $△ A C D, D O$ is the median.
$∴ a r (△ O A D) = a r (△ O C D) . ... (i i i)$
From (i), (ii) and (iii), we get
$∴ a r (△ O A B) = a r (△ O B C) . ... (i)$

|

Updated on:21/07/2023

Class 9 MATHS AREA OF PARALLELOGRAMS AND TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Show that each diagonal of a parallelogram divide it into two congruent triangles.
The following are the steps involved in showing the above result. Arrange them in sequential order.
A) In $△ A B C$ and $△ C D A$ , AB=DC and BC=AD $(∴$ opposite angles of parallelogram) AC=AC (common side).
B) Let ABCD be a parallelogram. Join AC.
C) By SSS congruence property, $△ A B C ≅ △ C D A$ .
D) Similarly, BD divides the triangle into two congruent triangles.
ABACD
BBDAC
CBADC
DBDCA
Question 2 - Select One
Asserion (A) : The diagonals of a ||gm divide it into four triangle of equal area.
Reason (R ) : A diagonal of a ||gm divides it into two triangle of equal area.
ABoth Assertion (A) and Reason (R ) are true and Reason (R ) is a correct explansion of Assertion (A).
BBoth Assertion (A) and Reason (R ) are true but Reason (R ) is not a correct explansion of Assertion (A).
CAssertion (A) is true and Reason (R ) is false.
DAssertion (A) is false and Reason (R ) is true.

RS AGGARWAL-AREAS OF PARALLELOGRAMS AND TRIANGLES -ASSERTION & REASON TYPE

Home
Ask Doubt
Profile