Let a force →F be acting on a body free to rotate about a point O and let →r the position vector of any point P on the line of action of the force then torque (τ) of this force about point O is defined as →τ=→r×→F given →F=(2ˆi+3ˆj−ˆk)N and →r=(ˆi−ˆj+6ˆk) m. find the torque of this force Solution in Telugu
→τ=→r×→F=∣∣
∣
∣∣ˆiˆjˆk1−1623−1∣∣
∣
∣∣=ˆi(1−18)+ˆj(12+1)ˆk(3+2)
→τ=(−17ˆi+13ˆj+5ˆk)N−m
Topper's Solved these Questions
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - SHORT ANSWER TYPE QUESTIONS
9 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
4 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
6 Videos
Similar Questions
State parallelogram law of vectors . Derive an expression for the magnitude and direction of the resultant vector .
View SolutionState and prove the parallelogram law of vectors.
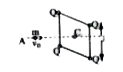
View SolutionFour identical point charges are fixed at the four corners of a square of a side length l. Another charged particle of mass m and charge +q is projected towards centre of square from a large, distance along the line perpendicular to plane of square. The minimum value of initial velocity V0 in m/s required to cross the square is? (m=1gm.l=4√2m,Q=1μc,q=0.5μc)
View SolutionA fish is at a depth of 12 cm in water is viewed by an observer on the bank of a lake. To what height the image of the fish is raised in cm ?
View SolutionLet →F be the force acitng on a paritcle having positon vector →rand→T be the torque of this force about the origin. Then
View SolutionLet →F be the force acitng on a paritcle having positon vector →rand→T be the torque of this force about the origin. Then
View SolutionLet a force F be acting on a body free to rotate about a point O and let r the position vector of ant point P on the line of aciton of the force. Then torque (tau) of this force abot point O is defined as tau = rxxF Given, F = (2hati + 3hatj - hatk)N and r = (hati- hatj+6hatk)m Find the torque of this force.
View SolutionFind the torque of a force →F=3ˆi+4ˆj+5ˆkN about a point O, whose position vector is →r=ˆi+ˆj+ˆkm.
View SolutionFind the moment of a force , →F=3→i+2→j acting at point →r=→i+2→k about a point of position vector →r=→i+→j.
View SolutionLet vecF be the force acting on a particle having position vector vecr and vec(tau) be the torque of this force about the origin. Then
View SolutionThe torque of a force vecF = 6hati acting at a point vecr= 4hatj about origin will be
View SolutionLet vecF be the force acting on a particle having position vector vecrandvectau be the torque of this force about the origin. Then :
View SolutionLet →F be the force acting on a particle having position vector →rand→T be the torque of this force about the origin. Then
View SolutionLet vec(F) be the force acting on a particle having position vector vec(r ) and vec(tau) be the torque of this force about the origin. Then
View SolutionIf a position vector of a point is →r=7ˆi+3ˆj+ˆk and force acting on it is →F=−3ˆi+ˆj+5ˆk then find torque.
View Solution
