Question

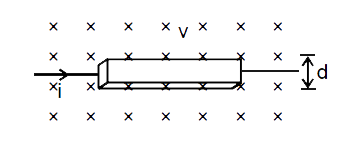
A strip of copper $75.0\mu m$ thick and 4.5 mm wide is placed in a uniform magnetic field $\overrightarrow{B}$ of magnitue 0.65 T. with $\overrightarrow{B}$ perpendicular to the strip. A current I = 57 A is then sent through the strip such that a hall potential difference V appears across the width of the strip. Calculate V. (The number of charge carries per unit volume for coppper is $8.47\times {10}^{28}$ electrons/$m^3$).

Explanation

To calculate the Hall potential difference $$V$$ across the width of the copper strip, we can use the formula for the Hall voltage:

$$V=\frac{BI}{nLe}$$

where:
- $$V$$ is the Hall potential difference,
- $$B$$ is the magnetic field strength,
- $$I$$ is the current,
- $$n$$ is the number of charge carriers per unit volume,
- $$L$$ is the thickness of the strip,
- $$e$$ is the charge of an electron.

1. Identify the given values:
- Thickness of the strip \( L = 75.0 \, \mu m = 75.0 \times 10^{-6} \, m \)
- Width of the strip (not needed for calculation) \( = 4.5 \, mm = 4.5 \times 10^{-3} \, m \)
- Magnetic field strength \( B = 0.65 \, T \)
- Current \( I = 57 \, A \)
- Number of charge carriers \( n = 8.47 \times 10^{28} \, electrons/m^3 \)
- Charge of an electron \( e = 1.6 \times 10^{-19} \, C \)

2. Substitute the values into the formula:
\( V = \frac{(0.65 \, T)(57 \, A)}{(8.47 \times 10^{28} \, electrons/m^3)(75.0 \times 10^{-6} \, m)(1.6 \times 10^{-19} \, C)} \)

3. Calculate the denominator:
- Calculate \( n \cdot L \cdot e \):
\( n \cdot L \cdot e = (8.47 \times 10^{28})(75.0 \times 10^{-6})(1.6 \times 10^{-19}) \)
- First, calculate \( 75.0 \times 10^{-6} \times 1.6 \times 10^{-19} \):
\( 75.0 \times 10^{-6} \times 1.6 \times 10^{-19} = 1.2 \times 10^{-24} \)
- Now multiply by \( n \):
\( 8.47 \times 10^{28} \times 1.2 \times 10^{-24} = 1.0164 \times 10^{5} \)

4. Calculate \( V \):
\( V = \frac{(0.65)(57)}{1.0164 \times 10^{5}} = \frac{37.05}{1.0164 \times 10^{5}} \approx 3.64 \times 10^{-4} \, V \)

5. Final result:
\( V \approx 3.64 \times 10^{-5} \, V \)