The distance of the point (1,1) from the line 2x−3y−4=0 in the direction of the line x+y=1 , is
A
√2
B
5√2
C
1√2
D
none of these
Video Solution
Text Solution
The correct Answer is:A
The equation of a line passing through P(1,1) and parallel to x+y=1, is
x−1cos3π4=y−1sin3π4
Let PM=r. Then , the coordinates of M are given by
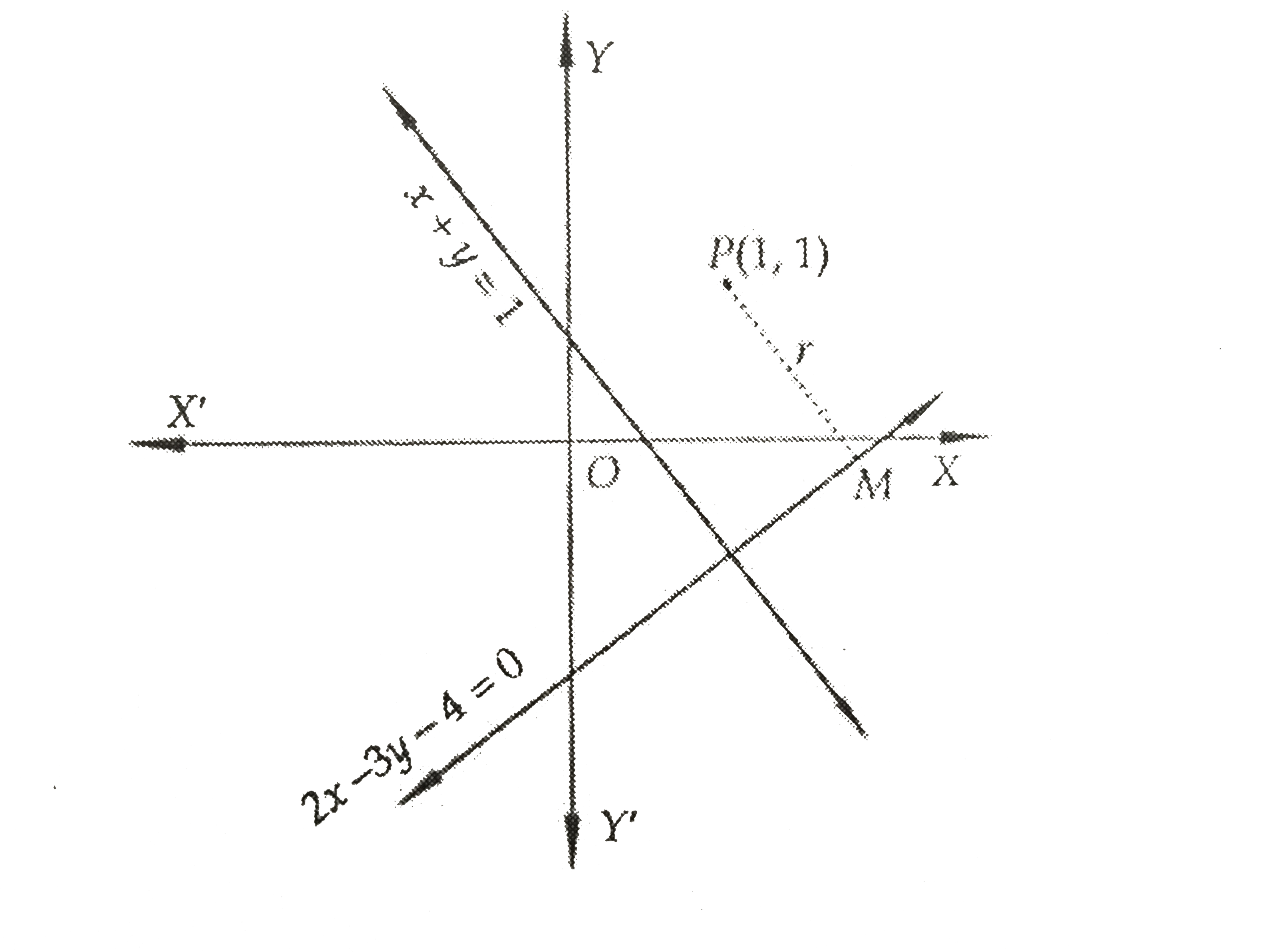
x−1cos(3π)4=y−1sin(3π)4=r or , (1−r√2,1+r√2)
∵ M lies on 2x−3y−4=0
∴2(1−r√2)−3(1+r√2)−4=0
⇒2−2r√2−3−3r√2−4=0⇒−5−5r√2=0⇒r=−√2
Hence , PM =√2
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The distance of the point (1,1) from the line 2x−3y−4=0 in the direction of the line x+y=1 , is
A√2B5√2C1√2Dnone of theseThe distance of the point P(1,−3) from the line 2y−3x=4 is
A13B2√2C√13D113The distance of the point (1, 3) from the line 2x+3y=6, measured parallel to the line 4x+y=4, is
A5√13 unitsB3√17 unitC√17 unitsD√172 units
The distance of the line 2x−3y=4 from the point (1, 1) in the direction of the line x+y=1 is
View SolutionDistance of point (1,3) from the line 2x−3y+9=0 along x−y+1=0
View SolutionFind the distance of the point (2,3) from the lilne 2x−3y+9=0 measured along a line x−y+1=0.
View SolutionThe distance of the point (3,5) from the line 2x+3y−14=0 measured parallel to the line x−2y=1 is
View SolutionThe distance of the point (1 , 3) from the line 2x + 3y = 6 measured parallel to the line 4 x + y = 4 , is :
View Solution
