Let a given line L1 intersect the X and Y axes at P and Q respectively. Let another line L2 perpendicular to L1 cut the X and Y-axes at Rand S, respectively. Show that the locus of the point of intersection of the line PS and QR is a circle passing through the origin
From the figure in ΔPQS, PQ⊥SR,PR⊥QS
Then we have QT⊥PS (as the altitudes of a triagnel are concurrent ).
Therefore, point O,R,T, and S are concyclic.
Hence, the locus of T is a circle which passes through the origin.
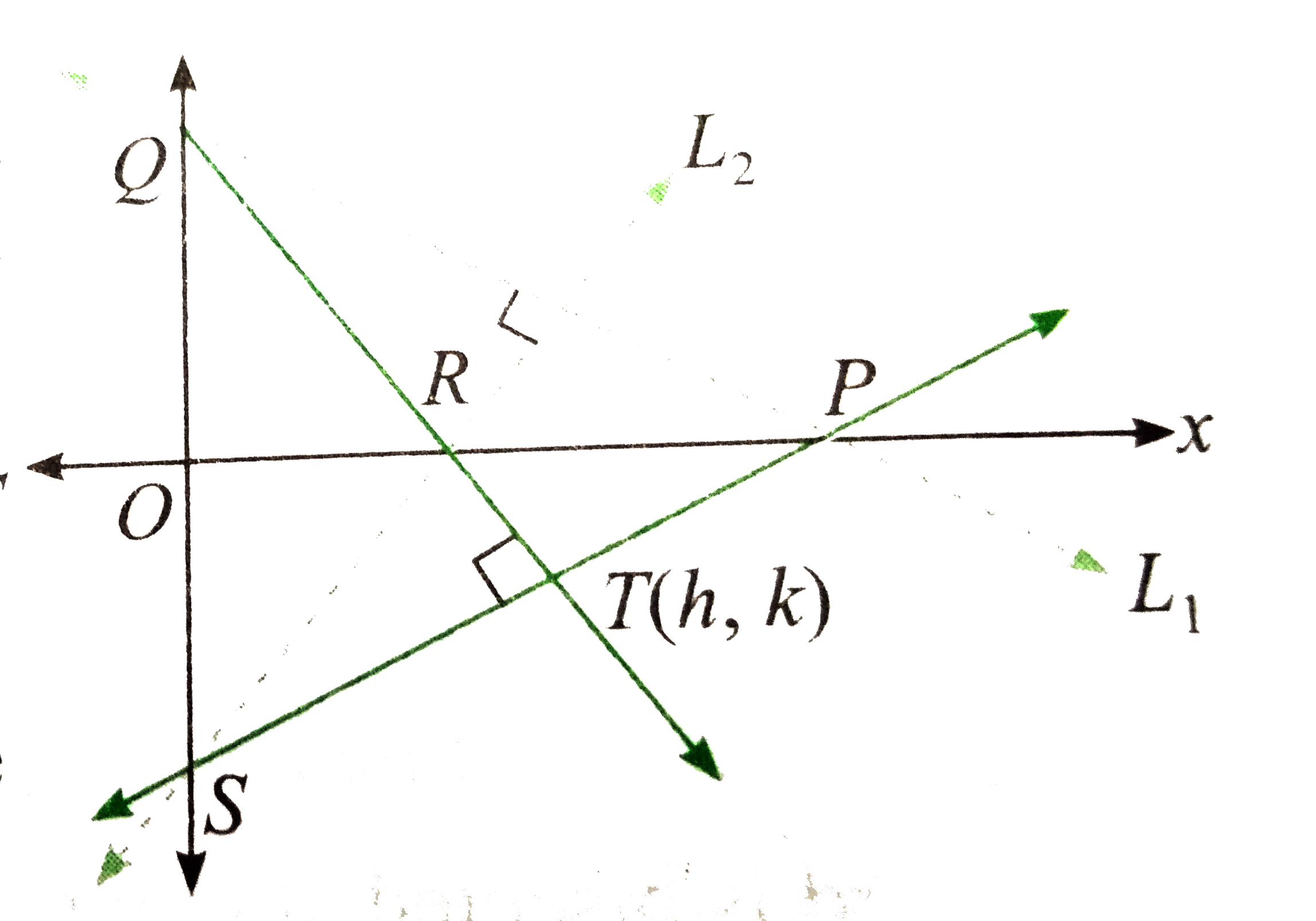
Topper's Solved these Questions
Similar Questions
Let a line L1:3x+2y−6=0 intersect the x and y axes at P and Q respectively. Let another line L2 perpendicular to L1 cut the x and y axes at R and S respectively.The locus of point of intersection of the lines PS and QR is
View SolutionA given line L1 cut x and y-axes at PandQ respectively and has intercepts aandb2 on x and y-axes respectively. Let another line L2 perpendicular to L1 cut x and y-axes at RandS respectively. Let T be the point of intersection of PSandQR. A straight line passes through the centre of locus of T. Then locus of the foot of perpendicular to it from origin is : (A) a straight line (B) a circle (C) a parabola (D) none of these
View Solution
Knowledge Check
A line L1=x10+y8=1 intersects the coordinate axes at points A and B. Another line L2 perpendicular to L1 intersects the coordinate axes at C and D. The locus of circumcentre of ΔABD is
A5x−4y=9B5x−4y=18C4x−5y=9D4x−5y=18
A given line L1 cut x and y-axes at PandQ respectively and has intercepts aandb2 on x and y-axes respectively. Let another line L2 perpendicular to L1 cut x and y-axes at RandS respectively. Let T be the point of intersection of PSandQR. Locus of T is a circle having centre at (A) (a,b) (B) (a,b2) (C) (a2,b) (D) (a2,b4)
View SolutionThe line x+y=1 cuts the coordinate axes at P and Q and a line perpendicular to it meet the axes in R and S. The equation to the locus of the point of intersection of the lines PS and QR is
View SolutionA given line L1 cut x and y-axes at PandQ respectively and has intercepts aandb2 on x and y-axes respectively. Let another line L2 perpendicular to L1 cut x and y-axes at RandS respectively. Let T be the point of intersection of PSandQR. If two chords each bisected by x-axis can be drawn from (a,b2) to the locus of T, then (A) a2>2b2 (B) b2>2a2 (C) a2<2b2 (D) b2<2a2
View SolutionThe line L1≡4x+3y−12=0 intersects the x-and y-axies at A and B, respectively. A variable line perpendicular to L1 intersects the x- and the y-axis at P and Q , respectively. Then the locus of the circumcenter of triangle ABQ is (a) 3x−4y+2=0 (b) 4x+3y+7=0 (c) 6x−8y+7=0 (d) none of these
View SolutionThe straight line xa+yb=1 cuts the axes in A and B and a line perpendicular to AB cuts the axes in P and Q. Find the locus of the point of intersection of AQ and BP .
View SolutionLet a line ax+yb=1 intersects the x-axis at A and y-axis at B resapectively. A line paralolel to it is drawn to intersect the axes in A and B respectively. The extermities of the lines are joined transversely. If the locus of point of intersection of the line joining them is xa=cyb, then c is equal to
View SolutionA line L1:x10+y8=1 intersects the coordinate axes at points A and B . Another line L2 perpendicular to L1 intersects the coordinate axes at C and D. The locus of circumcentre of ΔABD is
View Solution
