Question

On dividing $(x^3-3x^2+x+2)$by a polynomial g(x), the quotient and remainder are $(x-2)\text{and}(-2x+4)$ respectively. Find g(x).

Explanation

Let `f(x) = (x^(3)-3x^(2)+x+2), q(x) = (x-2) and r(x) = (-2x+4).`
Then, `f(x) = g(x)*q(x) + r(x)`
` rArr g (x) = ({f(x)-r(x)})/(q(x)).` …(i)
Now, ` {f(x) - r (x)} = (x^(3)-3x^(2)+x+2)-(-2x+4)`
` = (x^(3)-3x^(2)+3x-2).`
` :. g(x)=((x^(3)-3x^(2)+3x-2))/((x-2)).` [using (i)]
On dividing `(x^(3)-3x^(2)+3x-2)` by (x-2) , we get g(x).

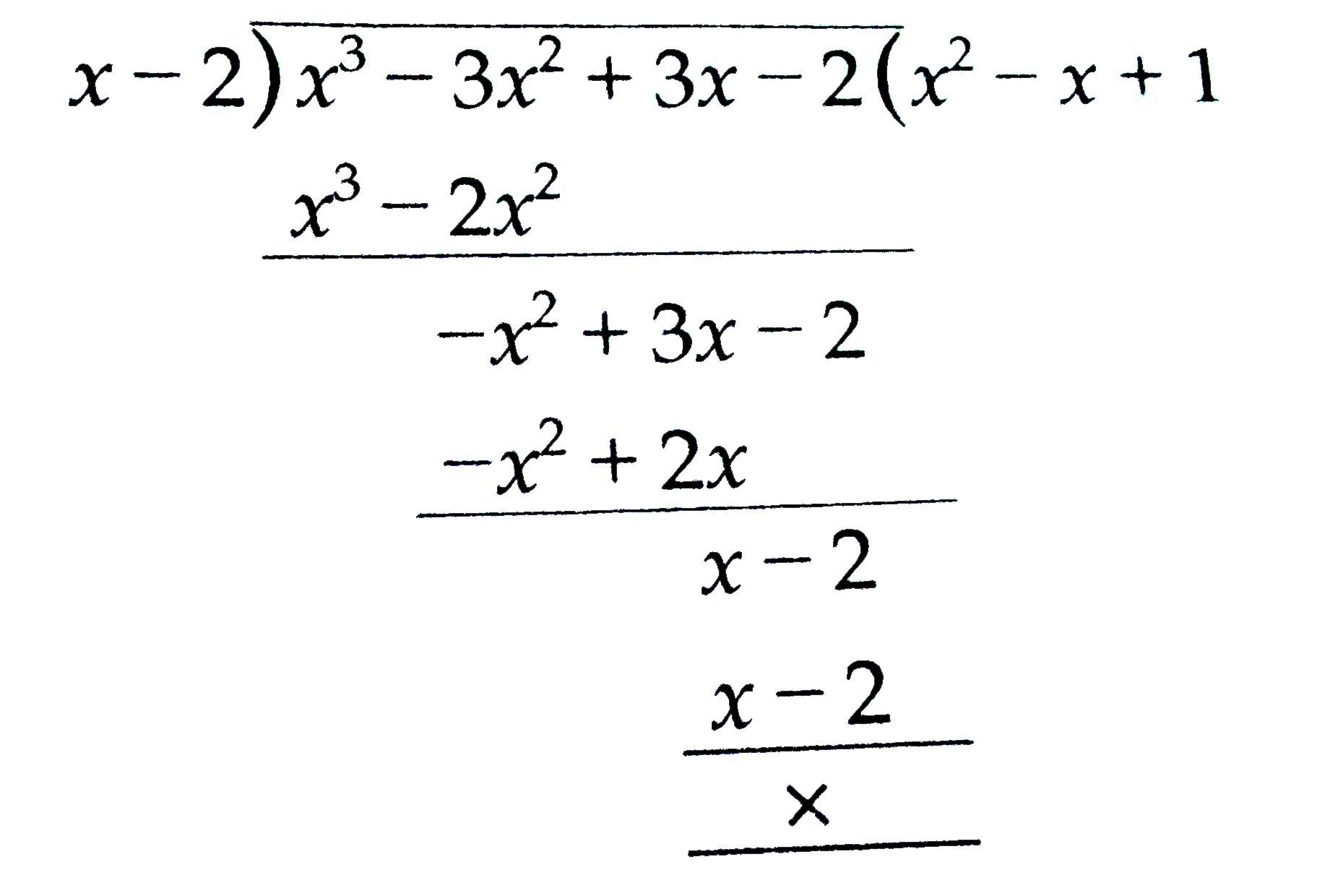
`:. g(x) = (x^(2)-x+1).`