An intially parallel cyclindrical beam travels in a medium of refractive index , where and are positive constants and is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
As the beam enters the medium, it will
diverge
converge
diverge near the axis and converge near the periphery
traval as a cylindrical beam
The correct Answer is:B
Step by step video, text & image solution for An intially parallel cyclindrical beam travels in a medium of refractive index mu (I) = mu_(0) + mu_(2) I, where mu_(0) and mu_(2) are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius. Answer the following questions : As the beam enters the medium, it will by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
An initially parallel cylindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is the intensity of the light beam. The intensity of the beam is decreasing with increasing radius.
30 . At the beam enters the medium, it will
View SolutionAn initially parallel cylindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is the intensity of the light beam. The intensity of the beam is decreasing with increasing radius.
31. The initial shape of the wavefront of the beam is
View Solution
Knowledge Check
An intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
The speed of light in the medium isAthe same everywhere in the beamBdirectly proportional to the intensity ICmaximum on the axis of the beamD( d) minimum on the axis of the beamAn intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
The initial shape of the wavefront of the beam isAconcaveBconvex near the axis and concave near the peripheryCplanarDconvexAn initially parallel cylindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is the intensity of the light beam. The intensity of the beam is decreasing with increasing radius.
32. The speed of light in the medium isAminimum on the axis of the beamBthe same everywhere in the beamCdirectrly proportional to the intensity IDmaximum on the axis of the beam
A concave lens having a material of refractive index μ1 is kept in a medium of R.I. μ2, where μ1<μ2. A parallel beam of light is incident on the lens. The concave lens will act as
View SolutionAn unpolarised beam of intensity I0 falls on a polariod. The intensity of the emergent light is :
View SolutionLight travels from a medium of refractive index μ1 to another of refractive index μ2(μ1>μ2). For total internal reflection of light, which is NOT true?
View SolutionLight travels from a medium of refractive index H, to another of refractive index μ2(μ1>μ2). For total internal reflection of light, which is NOT true?
View SolutionThree transparent media of refractive indices μ1,μ2,μ(3) respectively, are stacked as shown. A ray of light follows the path shown. No light enters the third medium.
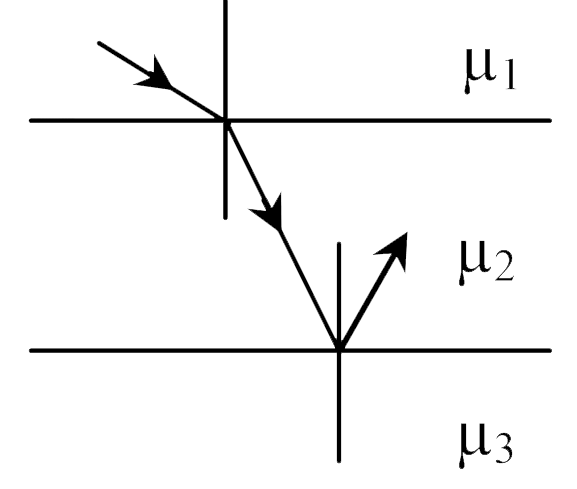
Then-
View Solution
