Which graph corresponds to an object moving with a constant negative acceleration and a positive velocity ?

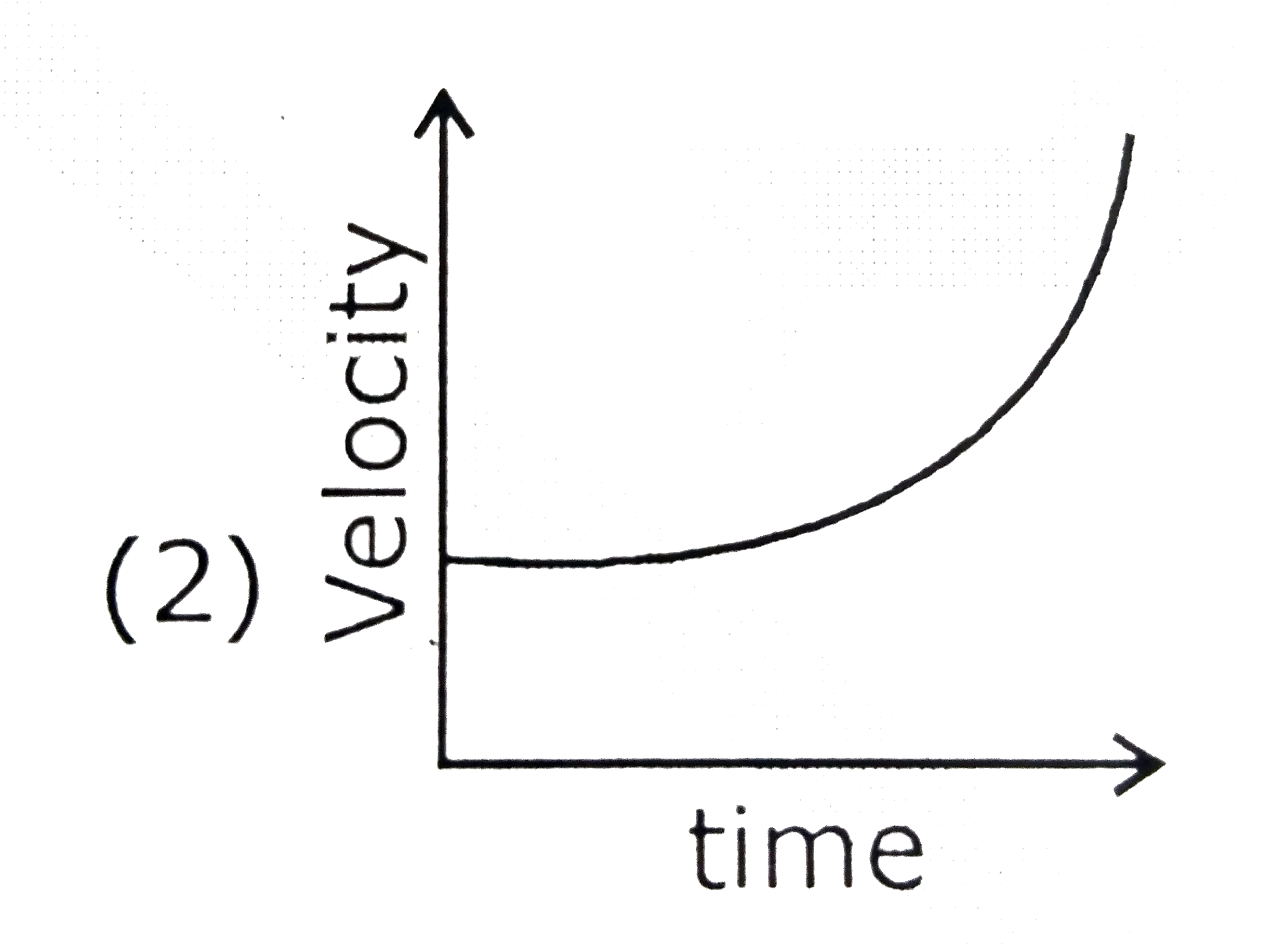

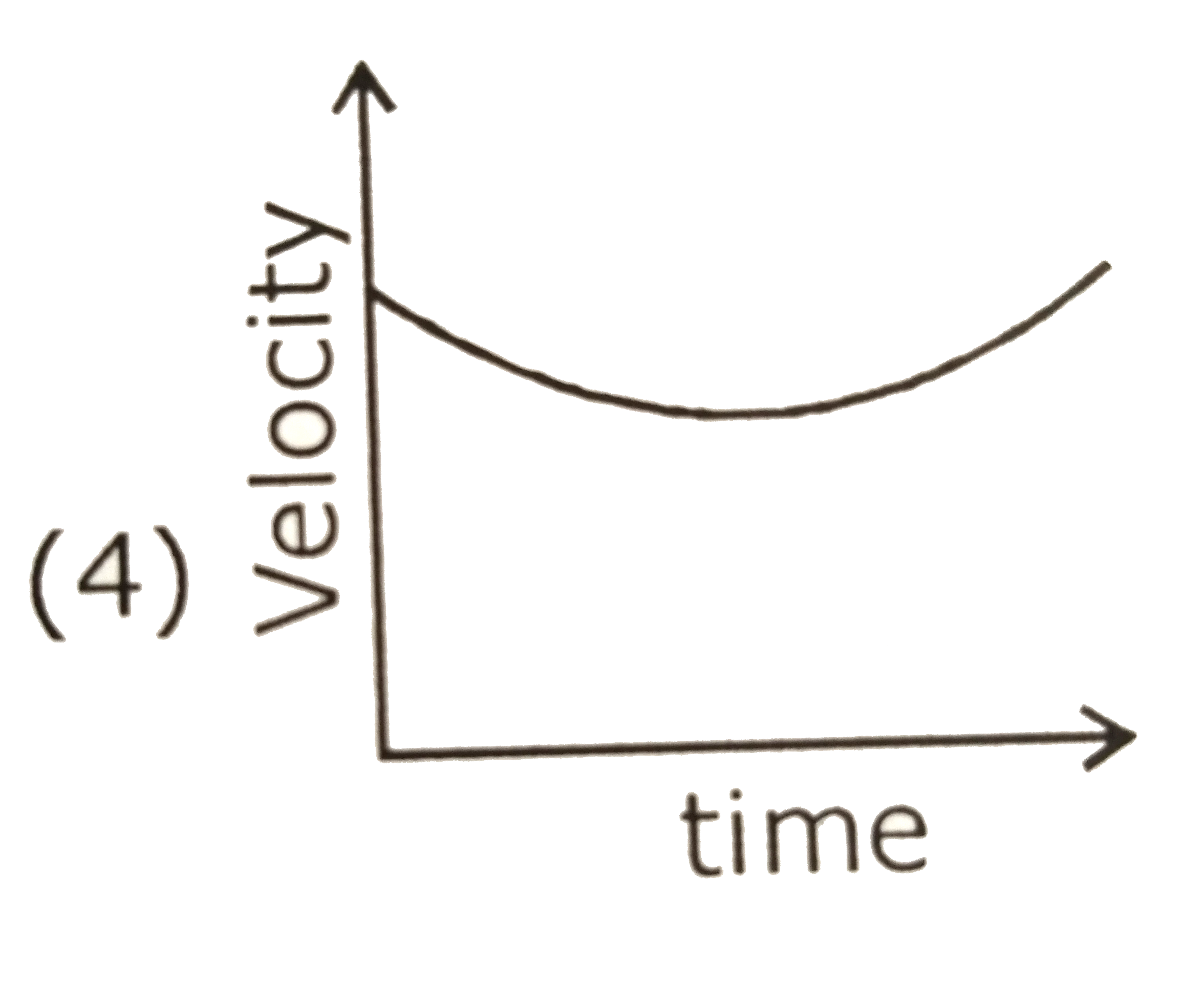
The correct Answer is:C
To determine which graph corresponds to an object moving with a constant negative acceleration and a positive velocity, we can follow these steps:
1. Understanding the Terms:
- Constant Negative Acceleration: This means that the object is slowing down. The acceleration is negative, which indicates that it acts in the opposite direction to the velocity.
- Positive Velocity: This means that the object is still moving in the positive direction, but its speed is decreasing due to the negative acceleration.
2. Mathematical Representation:
- Let the acceleration be a=−c (where c is a positive constant).
- According to the kinematic equations, we can relate acceleration, velocity, and displacement.
3. Using the Relationship Between Velocity and Displacement:
- We can use the equation vdvdx=a.
- Substituting a=−c, we have:
vdvdx=−c
4. Separating Variables:
- Rearranging gives:
vdv=−cdx
5. Integrating Both Sides:
- Integrate both sides:
∫vdv=∫−cdx
- This results in:
v22=−cx+k
- Rearranging gives:
x=−v22c+kc
6. Analyzing the Equation:
- The equation x=−v22c+kc is a quadratic equation in terms of v and represents a downward-opening parabola.
- As v decreases (due to negative acceleration), x will also change, indicating that the object is moving in the positive direction but slowing down.
7. Graphical Interpretation:
- The graph will show a downward-opening parabola, indicating that as the displacement x increases, the velocity v decreases.
- Therefore, the correct graph corresponds to a curve that starts from a positive value and slopes downwards.
8. Conclusion:
- The graph that matches this description is option 3, which shows a downward trend in velocity as displacement increases.
- Constant Negative Acceleration: This means that the object is slowing down. The acceleration is negative, which indicates that it acts in the opposite direction to the velocity.
- Positive Velocity: This means that the object is still moving in the positive direction, but its speed is decreasing due to the negative acceleration.
- Let the acceleration be a=−c (where c is a positive constant).
- According to the kinematic equations, we can relate acceleration, velocity, and displacement.
- We can use the equation vdvdx=a.
- Substituting a=−c, we have:
vdvdx=−c
- Rearranging gives:
vdv=−cdx
- Integrate both sides:
∫vdv=∫−cdx
- This results in:
v22=−cx+k
- Rearranging gives:
x=−v22c+kc
- The equation x=−v22c+kc is a quadratic equation in terms of v and represents a downward-opening parabola.
- As v decreases (due to negative acceleration), x will also change, indicating that the object is moving in the positive direction but slowing down.
- The graph will show a downward-opening parabola, indicating that as the displacement x increases, the velocity v decreases.
- Therefore, the correct graph corresponds to a curve that starts from a positive value and slopes downwards.
- The graph that matches this description is option 3, which shows a downward trend in velocity as displacement increases.
Topper's Solved these Questions
Similar Questions
The distance coverd by an object between times t1andt2 is given by the area under the v−t gaph between t1andt2 Prove this staement for an object moving with negative acceleration and gaving and having a positive velocity at time t1andand¬ativevelocityattimet_(2).
View SolutionWhich of the following x-t graphs shows an object moving with negative acceleration ?
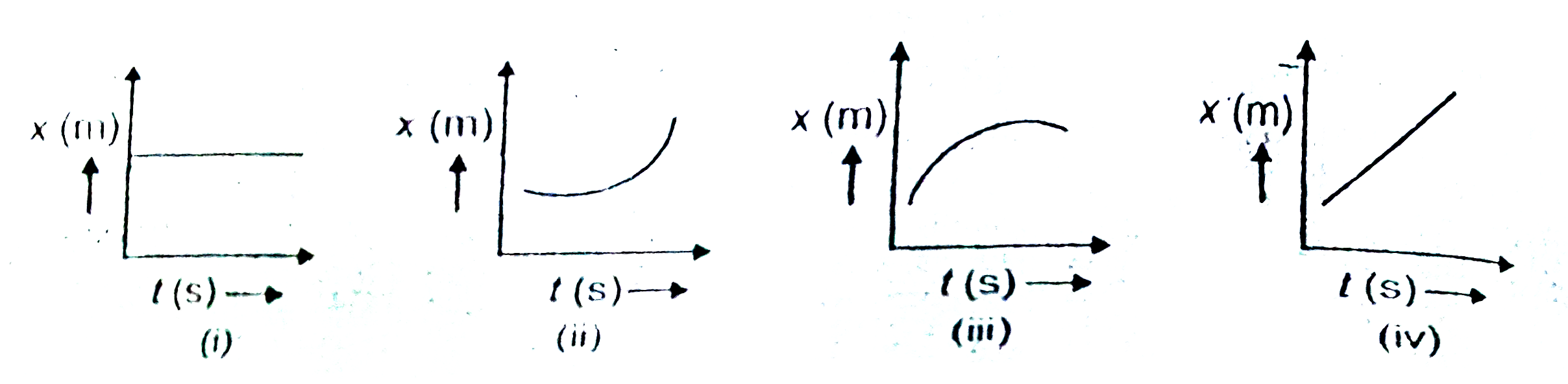
View Solution
Knowledge Check
Position - time graph for motion with negative acceleration is
A B
B C
C D
D
A : The area under acceleration - time graph is equal to velocity of object.
R : For an object moving with constant acceleration, position - time graph is a straight line.AIf both Assertion & Reason are true and the reason is the correct explanation of the assertion, then mark (1).BIf both Assertion & Reason are true but the reason is not the correct explanation of the assertion, then mark (2).CIf Assertion is true statement but Reason is false, then mark (3).DIf both Assertion and Reason are false statement, then mark (4).
Which of the following x - t graphs shows an object moving with negative acceleration?

View SolutionDraw the velocity-time graph of an object moving with uniform negative acceleration.
View SolutionDraw the position-time graph of an object moving with positive accceleration.
View SolutionShow that the area under the velocity-time graph of an object moving with constant acceleration in a straight line in certain time interval is equal to the distance covered by the object in that interval.
View SolutionShow that area under the velocity-time graph of an object moving with constant acceleration in a straight line in certain time interval is equal to the distance covered by the object in the interval.
View SolutionDraw the velocity-time graph of an object moving with uniform positive acceleration.
View Solution
