The two vectors $\to a$ and $\to b$ have equal magnitudes of $10.0$ m and the angles are $θ_{1} = 30^{\circ} and θ_{2} = 105^{\circ} .$ Find the (a ) x and (b) y components of their vector sum $\to r,$ (c ) the magnitude oof $\to r$ makes with the positive direction of the x axis.

Video Solution

Text Solution

Verified by Experts

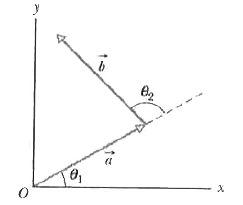
$(a) 1.59 m, (b) 12.1 m, (c) 12.2 m, (d) {82.5}^{\circ}$

Updated on:21/07/2023

The x and y components of a position vector P have numerical values 5 and 6 respectively. Direction and magnitude of vector P are
A ${tan}^{- 1} (\frac{6}{5})$ and $\sqrt{61}$
B ${tan}^{- 1} (\frac{5}{6})$ and $\sqrt{61}$
C $60^{\circ}$ and 8
D $30^{\circ}$ and 9
A displacement vector, at an angle of $30^{\circ}$ with y-axis has an x-component of $10$ units. Then the magnitude of the vector is-
A5
B10
C11.5
D20
If the unit vectors $\to a and \to b$ are inclined of an angle $2 θ$ such that $∣ ∣ \to a - \to b ∣ ∣ < 1 and 0 \leq θ \leq π$ then $θ$ in the interval
A $[0, \frac{π}{6}) \cup (5 π / 6, π]$
B $[0, π]$
C $[π / 6, π / 2]$
D $[π / 2, 5 π / 6]$