Two buses are running antiparallel in East-West. Direction. Calculate the velocity of Bus II with respect to Bus I. the speeds of Bus I and II are 60km h−1 and 90 kmhr−1 respectively. Also calculate the velocity of road with respect to Bus I.
To solve the problem, we need to calculate the velocity of Bus II with respect to Bus I and also the velocity of the road with respect to Bus I. Let's break it down step by step.
Step 1: Convert the speeds from km/h to m/s
We know that:
1 km/h = (1000 m / 3600 s) = (5/18) m/s
For Bus I:
- Speed of Bus I = 60 km/h
- Convert to m/s:
Speed of Bus I in m/s=60×518=30018≈16.67 m/s
For Bus II:
- Speed of Bus II = 90 km/h
- Convert to m/s:
Speed of Bus II in m/s=90×518=45018=25 m/s
Step 2: Assign directions to the velocities
- Let’s assume the positive direction is East.
- Therefore, the velocity of Bus I (going East) is +16.67 m/s.
- The velocity of Bus II (going West) will be negative, so it is -25 m/s.
Step 3: Calculate the velocity of Bus II with respect to Bus I
The relative velocity of Bus II with respect to Bus I can be calculated using the formula:
v2 with respect to 1=v2−v1
Substituting the values:
v2 with respect to 1=(−25)−(16.67)=−25−16.67=−41.67 m/s
This means Bus II is moving at 41.67 m/s towards the West with respect to Bus I.
Step 4: Calculate the velocity of the road with respect to Bus I
The velocity of the road with respect to Bus I can be calculated using:
vroad with respect to 1=vroad−v1
Since the road is at rest, its velocity is 0:
vroad with respect to 1=0−16.67=−16.67 m/s
This indicates that the road is moving at 16.67 m/s in the opposite direction (West) with respect to Bus I.
Final Answers:
1. The velocity of Bus II with respect to Bus I is -41.67 m/s (indicating it is moving towards the West).
2. The velocity of the road with respect to Bus I is -16.67 m/s (indicating it is moving towards the West).
---
We know that:
1 km/h = (1000 m / 3600 s) = (5/18) m/s
- Speed of Bus I = 60 km/h
- Convert to m/s:
Speed of Bus I in m/s=60×518=30018≈16.67 m/s
- Speed of Bus II = 90 km/h
- Convert to m/s:
Speed of Bus II in m/s=90×518=45018=25 m/s
- Let’s assume the positive direction is East.
- Therefore, the velocity of Bus I (going East) is +16.67 m/s.
- The velocity of Bus II (going West) will be negative, so it is -25 m/s.
The relative velocity of Bus II with respect to Bus I can be calculated using the formula:
v2 with respect to 1=v2−v1
Substituting the values:
v2 with respect to 1=(−25)−(16.67)=−25−16.67=−41.67 m/s
This means Bus II is moving at 41.67 m/s towards the West with respect to Bus I.
The velocity of the road with respect to Bus I can be calculated using:
vroad with respect to 1=vroad−v1
Since the road is at rest, its velocity is 0:
vroad with respect to 1=0−16.67=−16.67 m/s
This indicates that the road is moving at 16.67 m/s in the opposite direction (West) with respect to Bus I.
1. The velocity of Bus II with respect to Bus I is -41.67 m/s (indicating it is moving towards the West).
2. The velocity of the road with respect to Bus I is -16.67 m/s (indicating it is moving towards the West).
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE ( A.(MULTIPLE CHOICE QUESTIONS))
20 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE ( B.(MULTIPLE CHOICE QUESTIONS))
74 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - REVISION EXERCISE (LONG ANSWER QUESTIONS)
5 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)
12 Videos
Similar Questions
A bus takes 30 minutes to travel a distance of 25 kilometres. Calculate the speed of the bus in km/h.
View SolutionA man is walking on a road with a velocity 3kmhr. Suddenly rain starts falling. The velocity of rain is 10km/hr in vertically downward direction. the relative velocity of rain with respect to man is :-
View Solution
Knowledge Check
Two motor cars A and B start simultaneously from the same point on two straight roads at 60∘ to one another with velocity 30 and 40 km/h, respectively. The velocity of A with respect to B will be equal to
A10/√2B10√(13)C15√(13)D5√3
two train A and B are moving on parallel tracks with velocities of 60kmh−1and90kmh^(-1) respectively but in oppiste directions. Find (i) the relative velocity of train A.w.r.t train B and (ii) the relative velocity of ground w.r.t tain A.
View SolutionA bus travelled a total distance s. it covered its journey in consecutive one-third distances with speeds 60km/hr, 70km/hr and 80km/hr, respectively. Calculate the average speed of bus for entire journey.
View SolutionTo a driver going east in a car with a velocity of 40kmh−1, a bus appears to move towards north with a velocity of 40√3kmh−1. What is the actual velocity and direction of motion of the bus ?
View SolutionA bus is moving rightward with a velocity of 15m/sec and on the bus a monkey is renning oppositely with a velocity of 5m/sec( with respect to the bus ). Nearby a helicopter is rising vertically up with a velocity of 10m/sec.

Find out the direction of the bus as seen by the helicopter's pilot.
View SolutionA bus is moving rightward with a velocity of 15m/sec and on the bus a monkey is renning oppositely with a velocity of 5m/sec( with respect to the bus ). Nearby a helicopter is rising vertically up with a velocity of 10m/sec.
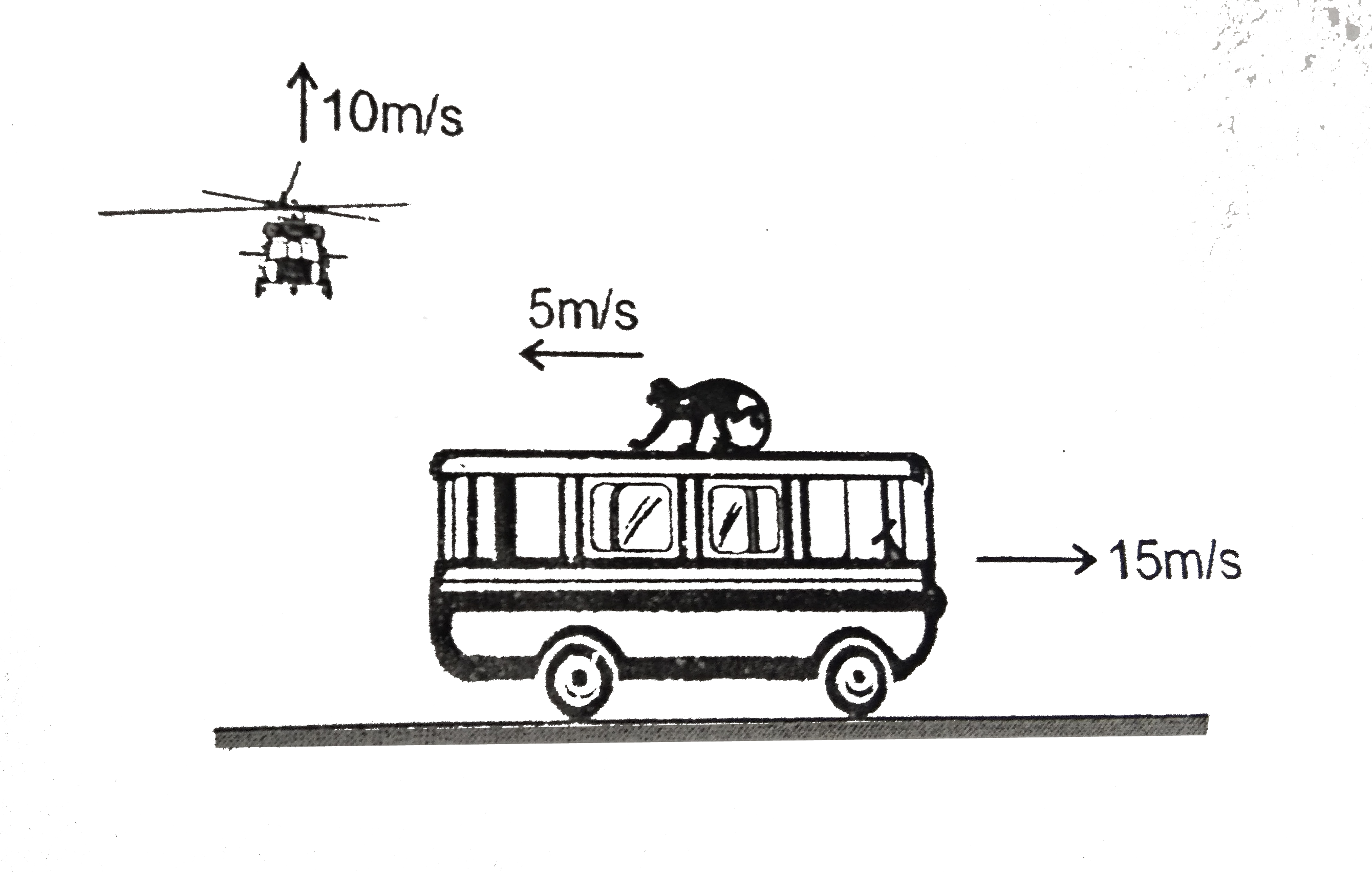
Find out the direction of the helicopter as seen by the monkey.
View SolutionA bus is moving with a velocity of 40kmh−1 due south, and a car is moving due west with a velocity of 50kmh−1. What is the velocity of car w.r.t. a passenger in the bus ?
View SolutionA bus decreases its speed from 72km/h to 54km/h in 10s. Calculate the acceleration of the bus.
View Solution
