A car of mass m travelling at speed v moves on a horizontal track. The centre of mass of the car describes a circle of radius r. If 2a is the separation of the inner and outer wheels and h is the height of the centre of mass above the ground, show that the limiting speed beyond which the car will overturn in given by
v2=grah
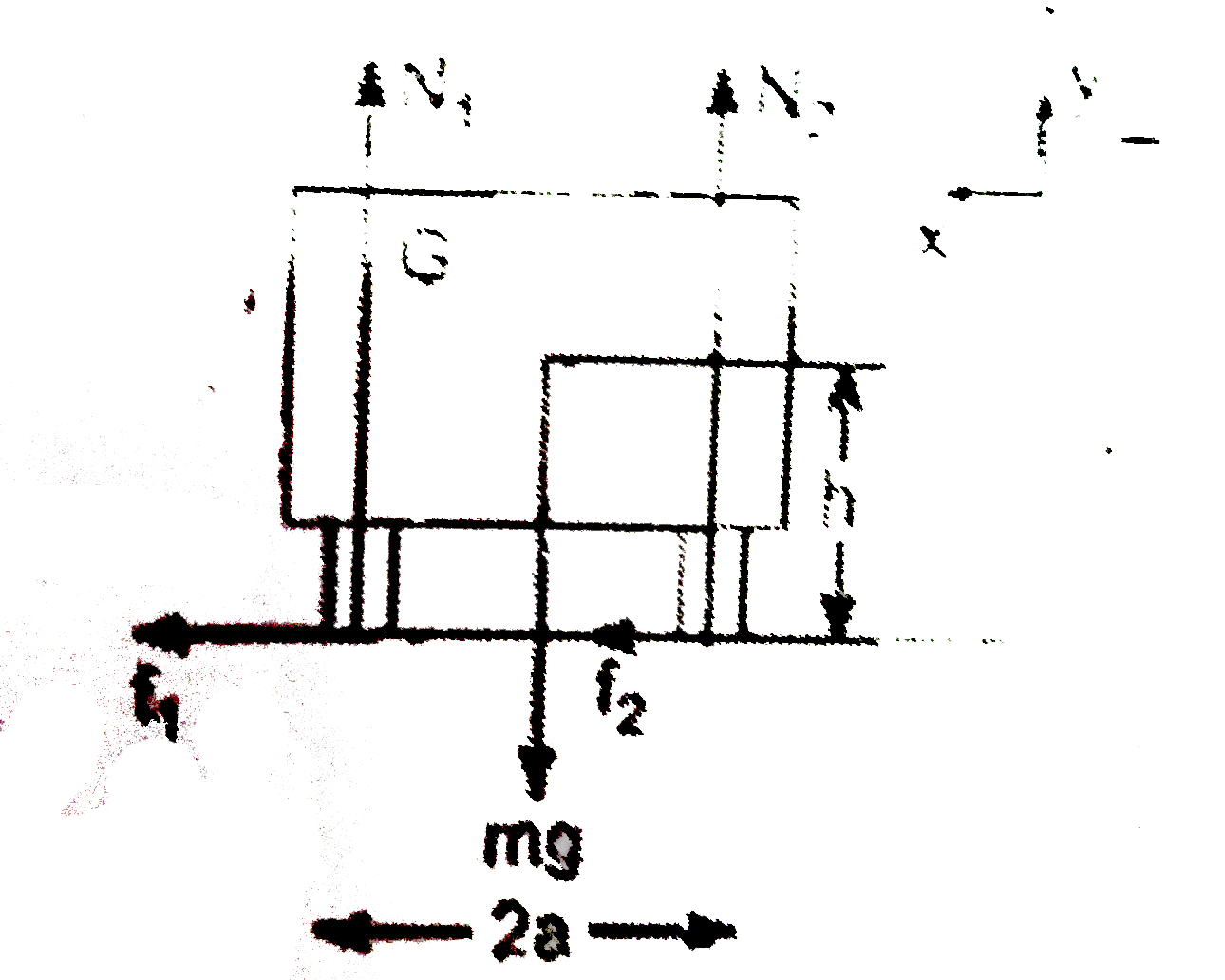
Figure 11-22a shows the rear view of the car that is turn ing toward left. If you have watched any action movie where car overturns, you may know that it overturns outward of the curve when traveling at a high speed.
Frictional force on the tires provides a centripetal force while turning. When car is going at a high speed, the inner tires will lose contact with the ground. We can consider car in equilibrium in its own frame .

Calculations: As it will be a non-inertial reference frame, we need to apply pseudo centrifugal force
∑Fx=F1+f2−mv2r=0 (11-32)
∑Fx=FN1+fN2−mg=0 (11-33)
From condition of rotational equilibrium, sum of moments about center of mass G must be zero:
∑τ=(f1+f2)h+FN1a−FN2a=0(11-34)
f1+f2=(FN1−FN2)ah(11-35)
On combining Eqs. 11-32 and 11-35, we can eliminate f1+f2 to obtain
FN1−FN2=mv2har(11-36)
Now, we can solve Eqs. 11-33 and 11-36 simultaneously to obtain
2FN1=mg−mv2hr and 2FN2=mg+mv2hr
The inner wheels will leave the ground when N1 becomes zero, that is,
mg=mv2har
v2=grah
Learn: As expected in Eq. 11-36, we can see that as v increases the FN1, will decrease and car will overturn out wardly.
If frictional force is not sufficient to provide centripetal acceleration, sideways skidding will occur instead of overturning.
f1+f2≤μ(FN1−FN2)
Equality sign holds when skidding starts:
FN1−FN2=mg
and f1+f2=mv2r
Therefore, the limiting speed at which skidding occurs is given by v2+μgr. If the car is to skid rather than overturn,
μgr<grah or μ<ah
Topper's Solved these Questions
- RIGID BODY DYNAMICS-IIBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - EXAMPLE
1 Videos
- RIGID BODY DYNAMICS-IIBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - CHECKPOINT
4 Videos
- RIGID BODY DYNAMICS - IBOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE))
3 Videos
- TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION BOOK - RESNICK AND HALLIDAYCHAPTER - TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION EXERCISE - PRACTICE QUETIONS (Integer Type)
4 Videos
Similar Questions
A car of mass m travelling at speed v moves on a horizontal track so that the centre of mass describes a circle of radius r . Show that the limiting speed beyond which the car will overturn is given by
v2=grah
where 2a is the separation of the inner and outer wheels and h is the height of the centre of mass above the ground .
View SolutionA car moves with speed v on a horizontal circular track of radius R. A head-on view of the car is shown in figure. The height of the car's centre of mass above the ground h, and the separation between its inner and outer wheel, is d. The road is dry, and the car does not skid. Show that the maximum speed the car can have without overturning is given by vmax=√gRd2h. To reduce the risk of rollover, should one increase or decrease h? Should one increase or decrease the width d of the wheel base?

View Solution
Knowledge Check
A car while travelling at a speed of 72 km/hr. Passes through a curved portion of road in the form of an arc of a radius 10 m. If the mass of the car is 500 kg the reaction on the car at the lowest point P is-
A25 KNB50 KNC75 KNDNone of theseA car moves with speed v on a horizontal circular track of radius R. A head-on view of the car is shown in figure. The height of the car's centre of mass above the ground is h, and the separation between its inner and outer wheels is d The road is dry, and the car does not skid. The maximum speed the car can have without overturning is :
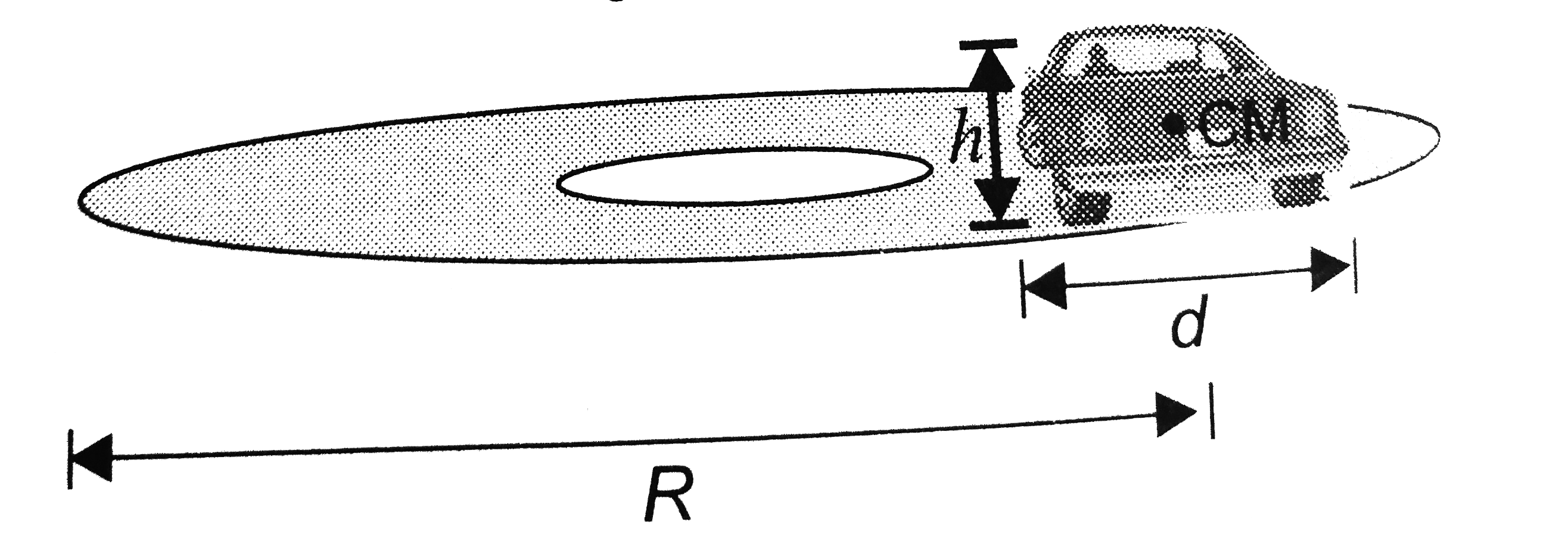 .A√gRd2hB√gRdhC√2gRdhD√2gRd3h
.A√gRd2hB√gRdhC√2gRdhD√2gRd3hA car of mass m moves in a horizontal circular path of radius r metre. At an instant its speed is Vm/s and is increasing at a rate of am/sec2.then the acceleration of the car is
AV2rBaC√a2+(V2r)2D√a+(V2r)
A car of maas M is moving on a horizontal circular path of radius r. At an instant its speed is v and is increasing at a rate a.
View SolutionA car goes on a horizontal circular road of radius R. The speed of car is increasing at constant rate a m/s2. If the friction coefficient between the road and the tyre is μ, then the limiting speed of car is
View SolutionA block of mass m moves on a horizontal rough surface with initial velocity v. The height of the centre of mass of the block is h from the surface. Consider a point A on the surface.
View SolutionA solid sphere of mass M and radius R is placed on a smooth horizontal surface. It is given a horizontal impulse J at a height h above the centre of mass and sphere starts rolling then, the value of h and speed of centre of mass are –
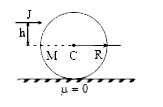
View SolutionA car of mass m moves in a horizontal circular path of radius r meter. At an instant its speed is Vm/s and is increasing at a rate of a m/sec2. Then the acceleration of the car is:
View Solution
