In the following questions, a series is given, with one term missing. Choose the correct alternative from the given ones.
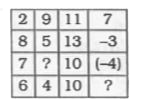
3 and 2
(-3) and 2
3 and (-2)
(-3) and (-2)
The correct Answer is:C
First number + Second number = Third number in each row
Second number - First number = Fourth number in each row.
First Row
2+9=11,9−2=7
Second Row
8+5=13,5−8=−3
Third Row
7+?=10
⇒10−7=3
3−7=−4
Fourth Row
6+4=10,4−6=−2
Similar Questions
In the following question, a series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
 View Solution
View SolutionIn each of the following questions, a series is given, with one term missing. Choose the correct alternative from the given ones that will complete the series.
 View Solution
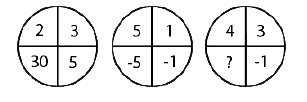
View SolutionIn each of the following questions, a series is given, with one term missing. Choose the correct alternative from the given ones that will complete the series
 View Solution
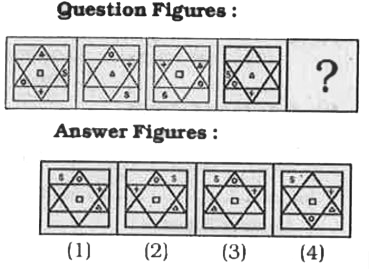
View SolutionIn the following question a series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
 View Solution
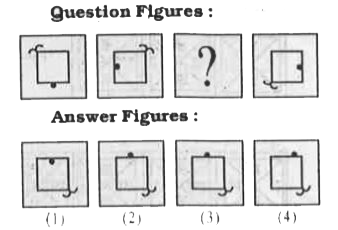
View SolutionIn each of the following questions a series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
 View Solution
View SolutionIn each of the following questions a series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
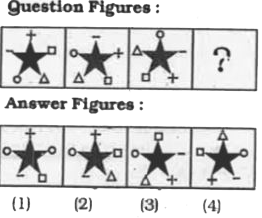 View Solution
View SolutionIn each of the following questions a series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
 View Solution
View SolutionIn each of the following questions a series is given, with one term missing. Choose the correct alternatives from the given ones that will complete the series.
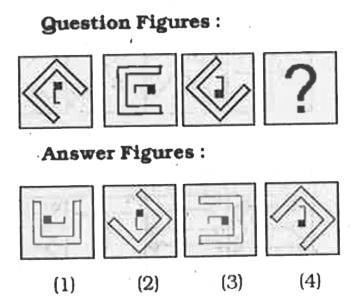 View Solution
View SolutionIn each of the following questions a series is given, with one term missing. Choose the correct alternatives from the given ones that will complete the series.
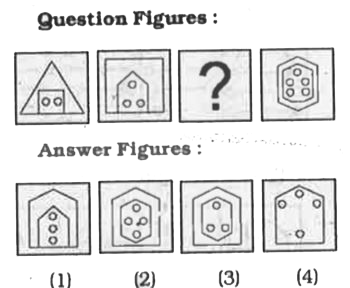 View Solution
View SolutionIn each of the following questions, a series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
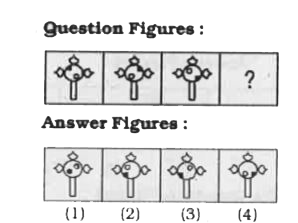 View Solution
View Solution
